1. Introduction

The ferroelectricity was first discovered in Rochelle salt (sodium potassium tartrate tetrahydrate) in 1920 by Valasek [1], who also confirmed the single polarization hysteresis loop and the piezoelectric response [2]. To data, ferroelectric (FE) materials have found a plethora of applications in FE random access memory (FeRAM) [3], energy storage capacitors [4], FE tunneling junctions (FTJs) [5], medical ultrasonic imaging transducers [6], ultrasonic motors [7], electromechanical sensors [8], etc. The notion of antiferroelectricity dates back to the early 1950s, and the formal definition of an antiferroelectric (AFE) state was proposed by Kittel in 1951 based on the antiferromagnetism scheme [9]. A material that exhibits a distinct double polarization hysteresis loop without remanent polarization (Fig. 1a) may be considered as an antiferroelectric [10, 11]. Almost all ferroelectrics show a decrease in dielectric permittivity with bias voltage, and the relative permittivity always decreases to 400 ~ 500 after full ferroelectric polarization [12]. Antiferroelectrics are capable of offering higher dielectric permittivities and peak-value responses with bias voltage (Fig. 1b), which allow for the development of high-energy-density capacitors and stable operation at elevated temperatures [12, 13]. Unlike ferroelectrics with typical butterfly-like strain responses, antiferroelectrics display a double strain loop (Fig. 1c). The perovskite lead zirconate (PbZrO₃) with orthorhombic Pbam space group symmetry was assigned as the first AFE material because of its dielectric behavior [14] and antiparallel Pb²⁺ ion displacement along the <110> direction [15], as shown in Figs. 1d-f. Unfortunately, electric-field-induced phase transformation between AFE and FE states in PbZrO₃ bulk ceramics was only accessible at higher temperatures (above 210 ℃) because the critical electrical field for the phase transition was higher than the breakdown field [16]. To reduce the critical field and obtain well-developed double polarization hysteresis loops at room temperature, substantial efforts have been made by using chemical substitutions [17-30], such as Ca²⁺, Sr²⁺, Ba²⁺, La³⁺ and Sm³⁺ at the A site and Sn⁴⁺, Ti⁴⁺ and Nb⁵⁺ at the B site. Among these substituted AFE systems, (Pb, La)(Zr, Ti)O₃ (PLZT) [22, 31, 32], (Pb, La)(Zr, Sn, Ti)O₃ (PLZST) [23-25, 33-38] and Pb(Zr, Sn, Ti)NbO₃ (PNZST) [26, 27, 39-41] are the most promising. Phase diagrams of (Pb_0.97La_0.02)(Zr, Sn, Ti)O₃ and Pb_0.99(Zr, Sn, Ti)_0.98Nb_0.02O₃ were first constructed by Berlincourt [20] in 1966. The electric-field-induced phase transitions between AFE and FE states were easily achieved at room temperature when the PbZrO₃-based antiferroelectrics had a tetragonal structure [11, 20]. The emergence of multiple AFE phases and field-induced phase transitions over a broad compositional range make PNZST and PLZST the most-studied PbZrO₃-based AFE materials to date.

The discussion of antiferroelectricity was taken beyond lead-containing AFE perovskites to include some lead-free AFE perovskites such as AgNbO₃ [42, 43], NaNbO₃ [44] and modified BiFeO₃ [45], pyrochlore groups [46], liquid crystals [47], hydrogen-bonded systems [48], two-dimensional materials such as SnTe [49] and β′−In₂Se₃ [50] and even ice [51]. Both NaNbO₃ and AgNbO₃ exhibit AFE phases with a complex sequence of temperature-driven phase transitions, and the room temperature AFE phases are isostructural in orthorhombic space group Pbcm [52, 53]. The antipolar displacements of Nb are responsible for the designation as an antiferroelectric [54]. In the last 5 years, there has been a surge of research activity in searching lead-free AFE materials.Fig. 2a provides statistics for refereed publications on antiferroelectrics from 1990 to the present. However, state-of-the-art antiferroelectrics still require Pb²⁺ as the A-site cation, which is mainly due to the absence of lead-free AFEs whose properties are competitive with those of their lead-based counterparts. The high level of toxicity of lead-based AFEs is mainly due to Pb or PbO, and the release of Pb occurs in the following steps: raw material extraction, calcination and sintering where PbO evaporates, hard machining of components, usage, recycling and waste disposal after usage. The release of Pb poses a risk to the sustainable development of the economy and society, and harms the environment, and eventually, contaminants will affect human beings through the food chain [55]. Effective regulation of the life cycle can reduce the release of Pb into the environment, but the toxicity of this element and increasing environmental risk will limit practical applications in the future. Compared to ferroelectrics, AFE materials have been much less investigated in the past several decades and have relatively limited applications [11, 56]. Nevertheless, AFE materials have unique field-induced first-order phase transitions between antipolar AFE and polar FE states in response to external stimuli (electric field, temperature and stress), which provides great opportunities for highly-efficient energy technologies, such as energy storage capacitors [4, 57, 58], pyroelectric energy conversion devices [59, 60], electrocaloric refrigeration [61], explosive energy conversion [62], and digital displacement transducers and sensors [63, 64], as shown Fig. 2b.

From this perspective, we first review recent advances in the development of AFE-based perovskites, including PbZrO₃-based, AgNbO₃-based, NaNbO₃-based and AFE-like (Na, Bi)TiO₃-based systems, for energy storage and conversion applications, followed by a discussion of issues and design strategies of AFE perovskites for practical applications. Additionally, we include the current understanding of the origin of antiferroelectricity and structure-property correlation. Finally, we provide an outlook on the search for new candidate lead-free AFE materials.

2. Recent advances in high energy performance antiferroelectrics 2.1. Antiferroelectrics for energy storage

In response to the insatiable demand for smaller, lighter, and more advanced electronic devices, the development of energy storage and conversion technologies has become an area of intense research interest. Dielectric capacitors present faster charging/discharging rates and better stability than batteries or supercapacitors [65], however, the key issues of today's dielectric capacitors (linear dielectric and anti-/ferroelectric capacitors) are their low energy density and efficiency. Theoretically, the recoverable energy storage density (W_r) of dielectric material is calculated by the following equation [4]: , whereP_r, P_max and E are the remanent polarization, the maximum polarization, and the applied electric field, respectively. The energy efficiency is given by η = W_r/(W_r + W_loss), where W_loss is the loss of energy density [4]. AFE materials show great promise due to their atypical polarization loops without remnant polarization and unique field-induced phase transitions between AFE and FE states, which can transform the energy with high efficiency [66]. For instance, an ultrahigh recoverable energy-storage density of > 10 J/cm³ and energy efficiency of > 80% were achieved in (Pb_0.98La_0.02)(Zr_0.55Sn_0.45)_0.995O₃ and (Pb_0.98–xLa_0.02Sr_x)(Zr_0.9Sn_0.1)_0.995O₃ AFE bulk ceramics [67, 68]. significant research has been reported in the last 5 years on the development of lead-free antiferroelectrics including AgNbO₃ (AN)-based [43, 58, 69-75], NaNbO₃ (NN)-based [76-78] and AFE-like (Na, Bi)TiO₃ (NBT)-based [79-83] systems in the quest to replace lead-containing counterparts as the main material for energy storage devices. As shown in Fig. 3a, the overall energy storage performance of these lead-free AFE materials is inferior to their lead-based counterparts [54, 57, 67, 68, 84-104]. In addition, the typical energy efficiency of lead-free AFE materials is generally lower than 80%. Such low energy densities and efficiencies will hinder the real application of lead-free AFEs for energy storage devices. In the future, it is still of significant importance to developing lead-free AFE materials that simultaneously possess high energy storage density as well as high energy efficiency. Moreover, not only the energy density and energy efficiency, but also the discharge behavior (peak current, power density, fatigue cycling, discharging time, and longevity) of AFE materials should be seriously considered especially for pulse-power applications such as accelerators, electron beams, and electromagnetic pulse weapons, etc. [54]. In general, the AFE to FE phase transition occurs on a time scale of microseconds or ever nanoseconds, as displayed in Fig. 3b. To data, works in this direction are still limited, and are mainly focused on lead-based systems [23, 67, 68, 76, 77, 85, 97, 100, 105-120]. In addition to high energy density and energy efficiency, good stability upon varying environmental temperatures and fatigue cycling is highly desirable to extend the potential for applications in the energy storage area.

Before antiferroelectrics can be put into real application for energy storage, several critical issues must be addressed to promote the applications of AFE materials. Both high energy density and high energy efficiency are essential prerequisites. On one hand, the stored electrical energy can be further enhanced utilizing increasing both electrical polarization and dielectric breakdown strength (DBS). The DBS of AFE materials can be improved by optimizing ceramic preparation techniques, while the electrical polarization can also be enhanced through crystallographic design or chemical modification [11, 54]. On the other hand, the most reported energy efficiencies of AFE materials are less than 80% due to the electrical hysteresis effect during the phase transition between AFE and FE. As a result, more than 20% of the total stored energy will be dissipated into the environment as heat energy. This heat dissipation will result in rising working temperatures and aggravate the failure progress of the AFE materials. Most studies on AFEs for energy storage capacitors have focused on improving the energy storage density and efficiency as discussed above. However, much less attention has been given to improving the reliability of the materials (temperature dependence and/or fatigue stability) for practical energy storage applications.

2.2. Antiferroelectrics for explosive energy conversion

Low-cost and eco-friendly energy conversion plays an important role in addressing the challenges of finite natural fuels [121]. Ferroelectric materials have aroused much attention in this area owing to their unique response to external stimuli. For example, the pressure-driven depolarization behavior in poled FE materials enables the fast conversion of mechanical energy to electrical energy, which has been extensively studied in this field for many years [122]. The field-driven FE-AFE phase transition of AFE materials seems to be the most straightforward approach due to the antipolar nature of the AFE state [62, 123]. This means that the bound electric charges of the field-induced metastable FE phase can be completely released within a short period (microseconds), generating a sharp voltage/current pulse. Therefore, AFE materials have been studied for many decades since the 1960s due to their numerous applications as explosive or pulse power energy conversion devices in renewable energy, medical industries, defenses, and mining areas [62, 123-125]. For example, −2% Nb-doped Pb(Zr_0.95Ti_0.05)O₃ (PZT 95/5) still dominates the commercial explosive energy conversion market after more than 50 years due to its well-developed phase transition from FE to AFE under low pressure [123]. Due to the success of the pressure-forced FE-AFE phase transformation, a record-high energy density of 5.401 J/g and generated pulse current peak value of 22 A have been observed in lead-free (Ag_0.935K_0.065)NbO₃ (AKN) ceramics [62], which is substantially higher than that of PZT 95/5 ceramics (2.929 J/g) and other FE ceramics [123-125], as shown in Fig. 3c. Thus, AKN ceramics may be an effective lead-free alternative to currently commercially used PZT 95/5 (2.929 J/g) in terms of explosive energy power conversion applications. However, the high cost of AgNbO₃-based ceramics limits their applications in practice. Research in this direction is rare, and it may emerge as an important area in the field of antiferroelectrics.

2.3. Antiferroelectrics for solid-state cooling

Over the past decades, there has been increasing interest in the development of alternative cooling technologies with higher efficiency based on an eco-friendly mechanism to solve our heat problem and to reduce the use of greenhouse gases that are mature and heavily employed in modern refrigeration [30-32, 61, 126-137]. Among these alternatives, electrocaloric materials undergo a significant adiabatic temperature change ΔT near the phase transition when an electric field is cyclically applied and withdrawn [134]. The ΔT can be calculated using an indirect method based on the thermodynamic Maxwell relations [134]:

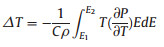
where C and ρ are the specific capacity and the density of the material, respectively. Benefitting from the phase transitions between AFE and FE states, AFE materials have recently received widespread attention for solid-state cooling devices [31, 32]. In 2006, a giant electrocaloric effect (ECE) with a ΔT of 12 K was reported in the PbZr_0.95Ti_0.05O₃ thin film near its Curie point of 222 ℃ [61]. Recent theoretical and experimental studies have demonstrated that both positive (ΔT > 0) and negative (ΔT < 0) effects exist in both FE and AFE materials [138-141]. As shown in Fig. 3d, PbZrO₃-based AFE materials enable outstanding electrocaloric performances (large positive or negative electrocaloric responses and electrocaloric strength, ΔT/ΔE), which offer the potential for highly efficient solid-state cooling devices. Compared to refrigeration utilizing positive ECE only, the cooling efficiency of a device combining negative and positive effects in one cooling cycle will be much improved [32, 127]. Unfortunately, many studies of the ECE in AFEs have been performed by the indirect thermodynamic method, which may lead to unreliable results. Li et al. [32] performed direct measurements on negative ECEs in Pb_0.97-xBa_xLa_0.02Zr_0.95Ti_0.05O₃ bulk ceramics, and confirmed that the coexistence of positive and negative ECEs can be used to improve the refrigeration efficiency and cooling capacity of solid-state refrigeration in a designed cooling cycle. Despite many advances that have been achieved over the last decade, there is no available solid-state cooling device based on AFE capacitors so far. In addition to high electrocaloric response and cooling efficiency, a broad operating temperature range and high reliability are highly desirable to extend the potential for real applications in the solid-state cooling area [142-144].

2.4. Antiferroelectrics for electro-mechanical energy conversion

The arrival of artificial intelligence offers opportunities for smart transducers and actuators that operate in various environmental conditions with high-performance. AFE materials are capable of achieving "digital displacement transducers" due to their unique reversible strain response behavior [145]. Currently, the most active investigations of AFE materials for actuator applications have concentrated on lead-based systems, such as PLZST-based polycrystalline materials with a large longitudinal strain in the range of 0.2%–0.85% [10, 23, 24, 146]. The high-precision positioning systems of actuators simultaneously require high strain and low hysteresis for practical applications [63, 147, 148]. To intuitively compare the actuator performance of lead-based and lead-free AFE materials, we summarize the strainversus hysteresis in Fig. 3e. Nevertheless, we can find that lead-free AFE or AFE-like materials usually exhibit a smaller strain than lead-based systems in strain versus electric field curves [149-157]. A combination of a giant strain response and low hysteresis, good thermal and frequency reliability, and high fatigue resistance are highly desirable for actuator applications. In particular, a large strain response with a low hysteresis is the key figure of merit for precisely controlled devices. It should be emphasized that an AFE material with a large strain response accompanied by a high hysteresis can also be employed for the development of digital displacement transducers.Fig. 3f shows the "ON" and "OFF" bistable strain output of the digital device driven under an applied triangular voltage. The output has a nearly rectangular shape, suggesting better controllability for a discrete motion device than traditional analogue displacement transducers [156].

2.5. Antiferroelectrics for pyroelectric energy conversion

The pyroelectricity effect rigorously relates a change in temperature to a change in spontaneous polarization [158]. The conventional pyroelectrics with reversible and stable pyroelectric responses are poled materials, which are operated below the Curie temperature [159, 160]. Lead-based AFE materials have been shown to possess more interesting phase-switching pyroelectric responses near the phase transition temperature and a DC bias, which enables technologically useful applications such as pyroelectric energy harvesting, thermal imaging, flame, fire alarms, and next-generation high-sensitivity infrared detectors [11, 30, 161]. In recent years, the composition/temperature effects on the pyroelectricity, depolarization temperature, FE-AFE phase transition behavior, and energy harvesting performance of lead-based AFE ceramics have been intensively investigated [30, 60, 161-165]. The large pyroelectric coefficient on the order of 10^–7–10^–6 C cm⁻² K⁻¹ near the depolarization temperature is only a one-time effect, but the pyroelectric response could be reversible during the heating or cooling process with a DC field [163]. The DC-field-driven pyroelectric response in AFEs is far larger than that of conventional phase transition pyroelectric materials, which is beneficial for the development of thermal imaging sensors and high-sensitivity infrared detectors [163, 166]. AFE materials can be used for thermal to electric energy conversion based on an Olsen cycle, making it an alternative for the reuse of waste heat [167, 168]. Great efforts have been made for decades to develop energy harvesting technologies for low-temperature waste heat. However, the field-forced method based on AFE materials still possesses low pyroelectric energy density, limited efficiency, low repetition rates, and low duty cycles, thus hindering their practical applications [57, 59]. Ongoing development efforts should be made to further understand the field-forced pyroelectric response of AFEs, and to establish strategies to improve the pyroelectric energy conversion performance of AFE materials.

2.6. Challenges and future opportunities

AFE materials show great potential for high energy density capacitors, high power output, solid-state cooling devices, pyroelectric energy conversion, and digital displacement transducers due to their unique field-induced phase transitions. Although the search for lead-free AFE materials has received growing attention, consistent efforts should lead to breakthroughs in the near future. Here, we will discuss the current challenges and several strategies to further improve the overall energy performance of AFE materials.

2.6.1. Explosive energy conversion

For the explosive energy conversion application, the energy storage density per unit of weight in the field-induced FE phase can be calculated via the following equation [169]: W = P_r²/2(ε₀×ε_r×ρ), where P_r, ε₀, ε_r and ρ are the remanent polarization of the induced FE phase, the permittivity of vacuum, the relative dielectric permittivity of the depoled AFE phase, and the bulk density, respectively. To date, the P_r achieved in polycrystalline antiferroelectrics is generally lower than 40 μC/cm². The low P_r is the most challenge in achieving higher explosive energy performance of AFEs. Here, we suggest three strategies to further improve the P_r of the induced FE phase: (ⅰ) conceptual design of morphotropic phase boundary (MPB) region by chemical substitution; (ⅱ) crystallographic texturing of polycrystalline AFE ceramics with MPB composition; (ⅲ) domain engineering. It is easy to realize field-induced the AFE-to-FE phase transition and obtain metastable induced FE phase in AFE materials with MPB composition due to the small energy difference between AFE and FE states. Crystallographic texturing of AFE ceramics offers a means of achieving significant enhancement in the P_r of the field-induced FE phase. Furthermore, the induced P_r of an AFE single crystal with specific orientations can be largely enhanced through the design of domain engineering, as plotted in Fig. 3g. We expect that a P_r higher than 60 μC/cm² can be realized in textured polycrystalline AFE ceramics or AFE single crystals in the near future. Therefore, high pulse voltage (> 100 kV), high current (> 100 A), and high power (> 10 MW) can be generated through a stress-induced FE-AFE phase transition. The crystallographic design is a good strategy to further improve the energy conversion performance of AFE materials [35]. It is expected that chemical optimized MPB and crystallographic design will bring new opportunities for the enhancement of explosive energy performance of antiferroelectrics.

2.6.2. Energy storage and high-power capacitors

The utilization of AFE materials is an effective approach to enhance the energy storage performances (energy density and efficiency) of dielectric capacitors. However, the state-of-the-art AFE materials are facing the most challenge of enhancing one parameter at the cost of the other. Especially, the energy efficiency of lead-free AFEs is generally very low (< 80%), leading to the high energy dissipation (more than 20% of the total energy) and inferior reliability associated with the first-order AFE–FE phase transition. A good approach to mitigate these issues is to synthesize relaxor-like AFE materials with slim double polarization hysteresis loops, as schematically shown in Fig. 3h. It has been demonstrated that the energy efficiency of "slim" PLZT AFE ceramics can reach 92% [170]. However, a suitable strategy combining relaxor and antiferroelectric features to design new AFE systems with "slim" double polarization loops remains an open research topic. The current widely used strategy lies in employing chemical doping approaches to introduce local structural heterogeneity in antiferroelectrics [66, 71, 171]. Recently, a high energy density of 6.3 J/cm³ with high efficiency of 90% has been reported in 0.45AgNbO₃–0.55AgTaO₃ AFE ceramics by constructing the local structure heterogeneity and AFE ordering of the phase boundary [171]. However, the doping amount, doping sites, and types of doping elements are usually determined through trial and error experimental attempts, which are still far away from sufficient ways with high accuracy. As a result, for the doping strategy, much effort can be devoted to perfecting and improving the database of doping elements in relaxor-like AFEs, including the doping amount, element types, and effectiveness, etc.

Despite the design of relaxor-like AFE materials, ceramic fabrication techniques should be taken into consideration. Much emphasis should be given to the ceramic sintering to deal with composition deviate from the designed stoichiometry at high temperature (e.g., volatilization of elements (Pb or Na) in AFEs or decomposition of silver oxides in AgNbO₃). Chemical approaches such as the atmosphere for sintering will contribute to the fabrication of AFE ceramics. The excess volatilization or decomposition can be effectively eliminated by using the newly-developed cold sintering technique [172], which may hold promise in the sintering of AFE ceramics.

The ceramic capacitors are usually stacked into multilayer structures for practical energy storage applications. For example, due to the higher electrical breakdown field, the energy storage density can be further enhanced by fabricating AFE multilayer ceramic capacitors (MLCCs). It has been reported that a high energy density of 9.5 J/cm³ and high energy efficiency of 92% can be achieved in "slim" (Na_0.5Bi_0.5)TiO₃-0.45(Sr_0.7Bi_0.2)TiO₃ AFE-like MLCCs [83]. In general, high-energy-density AFE capacitors are expected for use in high-power applications. However, AFE ceramic capacitors are less competitive in power conditioning or large pulse power systems requiring very high total energy levels (kJ to MJ). For instance, an energy density of 3 J/cm³ was achieved in multilayer PZT 95/5 AFE film energy storage devices with giant power density on the order of 2 MW/cm³ [173]. It is clear that multilayers will open new opportunities for the enhancements of energy density and power density of lead-based and lead-free antiferroelectrics. From the material and chemical point of view, the tape-casting technique [83] will benefit the ceramic layers with finer grain size and lower porosity, leading to a higher breakdown field. To date, the investigation of the energy storage and conversion performances of AgNbO₃- and NaNbO₃-based AFEs is in the infancy stage and no MLCCs based on these systems have yet been reported. We can be optimistic that much more works will concentrate on the energy performance of lead-free AFE MLCCs in the next few years.

2.6.3. Electro-mechanical energy conversion

Relaxor-like AFE materials also have a low hysteresis of strain response, which will benefit the development of precisely controlled smart actuators and sensors. It is known that the field-induced phase transition between AFE and FE is usually accompanied by a large electrical polarization generation or release as well as a massive volumetric expansion or contraction [24]. The volumetric expansion/contraction will aggravate the failure progress of the AFE materials. This issue is a common drawback concerning the first-order AFE-FE phase transition of AFE materials, which can give rise to mechanical failure, and degradation, and reduce the mechanical and functional endurance of AFE materials. Therefore, it will be of equal importance to remove the unwanted strain response and take into account the mechanical confinement of AFE materials [174]. Separating polarization and strain responses in AFE materials remains a central feature of both fundamental science and technological importance, but a strong intrinsic coupling between polarization and strain is the main challenge for practical applications of today's antiferroelectrics. Antiferroelectric material has received a lot of attention over the last years due to its large longitudinal strain (up to 0.9%) and short response time (1−2 μs) [23, 24]. Past investigations on AFEs have concentrated on polycrystalline materials, but their applications are limited by the crystallographic isotropy [11]. It is well acknowledged that anisotropic single crystals with specific crystallographic orientations could find many superior properties than their polycrystalline counterparts [156, 175]. We predict a 2–3 times larger longitudinal strain (up to 2%) in single-crystal form, similar single-crystal enhancement having been empirically known in Pb(Mg_1/3Nb_2/3)O₃-PbTiO₃ (PMN-PT) [175]. Besides large strain response, satisfactory cycling reliability should also be taken into account for electro-mechanical energy conversion devices.

2.6.4. Thermal-electrical energy interconversion

It was shown in the 2.3 section that thin films show giant ECE because they can withstand ultrahigh electric fields. Electrocaloric AFE thin films, however, can only pump a small amount of heat, thus limiting the solid-state refrigeration application. The electrocaloric effects tend to be relatively small in AFE bulk materials because they cannot support very large fields without electrical breakdown [32]. This is the main reason why the ECE in anti-/ferroelectrics has reached a plateau over the last years. The current most effective approach to overcome the above problems without compromising the tolerance to high electric fields in ferroelectrics is to fabricate a multilayer structure comprising many electrode films [176, 177]. The metallic electrodes themselves can be used to help transfer heat between the rest of the thermal pathway and the electrocaloric layers in a designed cooling device. AFEs have giant adiabatic temperature change, but no attempts of MLCCs based on good AFE materials have yet to be investigated. Improvements in the fabrication process and AFE MLCC structures could also be important if they permit larger fields to drive larger electrocaloric effects. Further study of AFE MLCCs that show electrocaloric effects is now timely, and the research prospects of ECE remain open and there would be significant potential for the development of commercially viable solid-state cooling devices.

Despite advances in the pyroelectric coefficient and pyroelectric energy density, much needs to be done to increase the efficiency of the pyroelectric energy conversion process. Recent efforts have moved even further by considering hybrid energy conversion cycles. For example, combined antiferroelectric/ferroelectric cycles have been proposed in a single device, taking full advantage of the nature of the large pyroelectric coefficients in these materials [59]. Within the conventional pyroelectric energy conversion approach, there is a need for a targeted design of materials with respect to a specific waste-heat source and also to minimize heat losses to auxiliary structures. The development of high-performance pyroelectrics will enable further investment in a burgeoning field of pyroelectric energy conversion, and a sustained research effort.

To sum it up, the developments of AFE materials have made great strides in the last 5–10 years, and some materials such as PLZT ceramics are available for smaller market segments and may require the next step of optimization for energy conversions. Specifically, the following suggestions can be provided for the development and scientific understanding of AFE materials for energy storage and conversion applications:

(a) Pay attention to the chemical composition, crystallographic design, and fabrication of MLCCs. (b) Explore the nature of field-dependent phase transitions between AFE and FE states. (c) Establish interactions between structure-property, overall energy performance, and general reliability.

3. Origin of antiferroelectricity and structure-property correlation

Developing improved AFE materials for real-world applications requires a fundamental comprehension of the crystallography and microstructure at different length scales. A better understanding of the structural origin of antiferroelectricity offers opportunities for comprehending structure-property correlation and exploring new AFE materials [178]. Unfortunately, a complete physical picture of antiferroelectrics has not been obtained to date owing to the complex lattice dynamics of AFE materials, thus heavily restricting the possibility of engineering the properties towards applications. The theoretical definition of AFE configuration was proposed by Kittel in 1951 as neighboring dipoles pointing in antiparallel directions [9]. In parallel, Shirane et al. [14] experimentally demonstrated AFE behavior in PbZrO₃ ceramic based on its characteristic dielectric anomaly and double hysteresis loop. Following comprehensive structural investigations, the basic dipole ordering arrangement of prototypical AFE PbZrO₃ was established in which the lead ions are aligned alternately in this way (↑↑↓↓↑↑↓↓), as shown in Fig. 4a. This defines the AFE character as per Kittel's definition [9], and it results in a commensurately modulated structure (CMS) and the appearance of [110] superlattice spots in the [001] selected area electron diffraction (SAED) pattern [179], as shown in the inset of Fig. 4a. In principle, there are three characteristic AFE features of orthorhombic PbZrO₃, which can be associated with the instabilities of the phonon modes in the first Brillouin zone of the cubic PbZrO₃ crystal lattice [178, 180]. First, the antiparallel Pb ion displacements along the [110] direction are associated with instability at the Brillouin zone-boundary phonon mode [181]. Second, the dielectric anomaly during the high-temperature cubic to orthorhombic AFE phase transition is related to instability in the Brillouin zone center mode [182]. Third, the opposite oxygen octahedral rotations in the pseudocubic unit cell along the (001)-axis originate from instability at the R corner of the Brillouin zone [183]. The softening of opposite oxygen octahedral rotational modes plays a significant role in structural phase transformations for FEs and AFEs [184, 185]. The recent polarized infrared ray and Raman spectroscopic results indicated that PbZrO₃ has multiple soft modes, resulting in a flat soft polarization branch near the AFE wave vector [180]. In PbZrO₃-based AFE materials, chemical modifications generally drive the AFE phase from CMS to an incommensurately modulated structure (IMS) [179, 186]. In 1990s, transmission electron microscopy (TEM) studies of IMS were performed in PbZrO₃-based ceramics [187, 188]. Later, the rather complex IMS with a sinusoidal modulation of A-site cations was first studied in (Pb_0.96La_0.04)(Zr_0.9Ti_0.1)_0.99O₃ ceramics using atomic-resolved scanning transmission electron microscopy (STEM) [189]. Recently, Ma et al. further revealed that the A-site cations were antiparallel but in a nearly orthogonal arrangement in (Pb_0.99Nb_0.02[(Zr_0.57Sn_0.43)_1−yTi_y]_0.98O₃ ceramics [179]. The incommensurate modulations manifest themselves as fine fringes in bright-field TEM images and satellite spots in reciprocal space (Fig. 4b). Most recently, the ferrielectric (FiE) configuration, containing FE segments with either angle or magnitude modulation of electrical dipoles [188, 190], was experimentally found in Pb_0.97La_0.02(Zr_0.50Sn_xTi_0.50-x)O₃ ceramics using atomic-resolved STEM [191], as shown in Fig. 4c. This FiE phase mainly stems from the coupling between A-site cations and O-anions [191]. Other functionalities may be accessed based on FiE segments, in which the sublattice polarizations do not completely cancel, resulting in a net spontaneous polarization. These effects would show the characteristic electric-field-induced transition of antiferroelectrics, but could also have a switchable polarization at lower fields. By combining advanced synchrotron X-ray, neutron and three-dimensional electron diffraction techniques, the incommensurately modulated intermediate state was revealed recently in Pb_0.99{Nb_0.02[(Zr_0.57Sn_0.43)_0.94Ti_0.06]_0.98}O₃ ceramics, which display imbalanced polarization and flexible configuration of a sinusoidal wave functional [41], as shown in Fig. 4d. Incommensuration in PbZrO₃-based AFE materials is related to the competing interactions between a zone-boundary ∑ phonon mode favouring AFE ordering in an orthorhombic structure and a zone-center Γ phonon mode favouring FE ordering in a rhombohedral structure [186]. In addition, incommensurate modulations were observed in (Pb, La)(Zr, Sn, Ti)O₃ AFE tetragonal single crystals [156], and field-induced transformation between incommensurate AFE and commensurate FE modulations enabled the emergence of complex domain structures and domain memory effects (Fig. 4e). Past investigations revealed that the generation of incommensurate superlattice reflections is related to the addition of La³⁺ in the (Pb, La)(Zr, Ti)O₃ and (Pb, La)(Zr, Sn, Ti)O₃ ceramics [192]. These studies have been considered an important step forward to the understanding of cation displacement and dipole arrangement in IMS.

For a typical commensurate AFE PbZrO₃, an unconventional intermediate phase with cycloidal order of polarization was obtained during the dynamic structure investigation using the negative-spherical-aberration imaging technique [193]. The emergence of spontaneous polarization (P_S) in the intermediate phase is resulted from the breaking of spatial inversion symmetry by the antiferrodistortive–ferrodistortive transition and hierarchical evolution of the oxygen octahedra. Specifically, the tails of the polarization vectors (Psx and Psy) can be approximated by a curtate cycloid (Fig. 4f). Tagantsev et al. [194] revisited antiferroelectricity in single-crystal PbZrO₃ by employing the X-ray scattering technique and Brillouin light scattering to study its lattice dynamics. The AFE state in PbZrO₃ is a missed incommensurate phase, and the paraelectric to AFE phase transition is attributed to mode softening via flexoelectric coupling [194]. As shown in Fig. 4g, the experimental diffuse scattering maps match well with simulations that consider flexoelectric coupling. However, later experimental measurements of the flexoelectric coefficient and flexo-coupling suggested that the flexoelectric effect of PbZrO₃ and AgNbO₃ at room temperature is too small to stabilize the AFE phases [195].

The antiferroelectric features of the lead-free compounds, including NaNbO₃, AgNbO₃, and (Bi_1/2Na_1/2)TiO₃, are quite different from those of PbZrO₃-based AFE oxides. For example, the IMS in the AFE P phase (space group Pbma) provides a bridge structure during the polarization reversal and AFE-to-FE phase transition and demonstrates the existence of competition between AFE and FE states in NaNbO₃-based AFE ceramics [196, 197]. Superlattice diffraction has been observed in AgNbO₃-based ceramics [198] and AFE-like (Bi_1/2Na_1/2)TiO₃-based solid solutions [199], but discussions on IMS, dipole arrangement, and cation displacement are still in their infancy. Despite systematic experimental and theoretical studies of antiferroelectrics over the last 70 years, the physical reason for the antiferroelectricity and modulated structures remains elusive, largely due to the complex sub-domain microstructures. Capturing a complete picture of the relationship between structure and property in antiferroelectrics requires advanced characterization techniques that can achieve deeper insight into the origin of antiferroelectricity and modulated structures. It was found that the modulation period of IMS appears to be linked with macroscopic properties including the dielectric constant, switching field, energy storage performance, etc. [191]. Studies including high-resolution TEM, atomic-resolved STEM, advanced synchrotron X-ray diffraction, and neutron diffraction are needed to disclose the mystery of the origin of antiferroelectricity, and explore the nature of field-induced ferroelectric/antiferroelectric phase transitions and comprehend structure-property correlations at multiple scales. In the near future, it is of significant importance to grow high-quality AFE single crystals because they offer a better platform for the fundamental understanding of antiferroelectricity, structure-property relationship, and crystal symmetry effects than typically used polycrystalline material where electrical and mechanical effects depend on the grain size and grain boundaries [11, 156]. A hindrance to fundamental structure-property investigations of AFEs is the great difficulty to grow high-quality single-crystal AFE, and AFE single crystals with well-developed double polarization loops are rarely observed experimentally. Attempts to grow AFE single crystals of PLZST have been made by Q. Li et al. [200] since 1997, and intensive research has been made on the growth defects, flux selection, composition optimization, domain structure, and field-induced phase transition of as-grown PLZST AFE single crystals [33-35, 56, 156]. Some new crystals (e.g., PbZr_1–xSn_xO₃ [201], Pb(Lu_0.5Nb_0.5)O₃-based AFE crystals [101], K₃Nb₃B₂O₁₂ [202]) have been proved to exhibit antiferroelectricity, much more effort is needed to made: (a) Seeking new methods or improving the growth method to grow large-size and high-quality AFE single crystals; (b) Measuring the single-crystal electric properties for [001], [011] and [111] crystallographic directions, and studying the structure-property relationship; (c) Understanding the origin of antiferroelectricity, and the mechanism of AFE-to-FE phase transition; (d) Exploring the relationship between modulation period of IMS/CMS and macroscopic properties including the critical switching field, energy performance, etc.

4. Search for new lead-free AFEs through the integration of theory and experiment

The (Bi_1/2Na_1/2)TiO₃-based, AgNbO₃-based, and NaNbO₃-based AFEs are prominent candidates for lead-free AFE material systems. The majority of research in this area has concentrated on the compositional tuning of lead-free perovskites to control essential features such as the critical switching field, field-induced phase transition, and strain response, leading to complex multi-component systems. However, it is still not possible to completely replace lead-containing AFE materials because the overall energy storage and conversion performances of these lead-free AFEs are inferior to that of their lead-containing counterparts. Significant effort should be made to design and discover new lead-free AFE materials with high energy storage and conversion performance.

It is striking that no AFE oxide conforms to the simple Kittel model. The antipolar structure of PbZrO₃ is generated by a zone-boundary phonon mode with auxiliary oxygen-octahedron rotation distortions stabilizing the AFE phase [194]. Therefore, a more promising route to new lead-free antiferroelectrics is to follow the paradigm set by canonical PbZrO₃ [191]. Considering the large body of compounds in the perovskite group and its derivatives, it is better to use theoretical calculations as screening tools to search for potential lead-free AFE candidates. Based on a combination of high-throughput first-principle-based calculations with crystallographic database mining, Xu et al. [203] predicted that lead-free AFE Bi_1–xNd_xFeO₃ systems can allow high energy densities of 100–150 J/cm³ and high energy efficiencies of 80%–88%. Another promising approach to search for the potential lead-free AFEs is from the view of crystal structure for perovskites. It is well known that, for maintaining charge neutrality, replacing Pb²⁺ with an aliovalent metal cation will result in perovskite structures with new formulas AB(Ⅴ)X₆, A₂B(Ⅳ)X₆, AB(Ⅰ)B(Ⅲ)X₆ and A₃B(Ⅲ)₂X₉ [204]. For example, Bi₂Mn_4/3Ni_2/3O₆ is antiferroelectric with an incommensurate structure, which is identified by reverse Monte Carlo modeling of neutron scattering data [205]. Note that antiferroelectricity has been demonstrated in other compounds, including Cs₂Nb₄O₁₁ [206], BiNbO₄ [207], ZrO₂ [208], MgSrSi [209], WO₃ [210] and van der Waals CuInP₂Se₆ [211]. In fact, it seems that there should be many choices for antipolar phonons that could yield an AFE state when coupled with other modes. First-principle-based calculations of full phonon dispersion, including but not limited to perovskites and their derivatives, can be very helpful in identifying phonon modes and AFE structures. For instance, high-throughput first-principles calculations revealed a class of antiferroelectric materials in the orthorhombic MgSrSi structure type [209]. A high-throughput search of antiferroelectrics will allow us to identify a family of perovskites or other compounds that exhibit a variety of polar and antipolar polymorphs and seem to be promising candidates for lead-free antiferroelectrics.

The phase stability and performance of lead-free AFEs are hard to predict through a purely theoretical approach, and thus a theory-experiment integrated method is necessary. Usually, Goldschmidt's tolerance factor (t) is widely used as an empirical rule to roughly estimate the phase stability of perovskites [11, 30, 66]. In general, the AFE phase is stable when t < 1, and the antiferroelectricity is improved by reducing t [66]. The intrinsic stabilities of new AFE compounds can be first confirmed using density functional theory (DFT) calculations [212]. The compounds are then synthesized and their phase stability and properties are studied experimentally. The results from these complementary theory-experiment studies provide directions for modifying the crystal structure/composition and finding potential candidates. Considering the numerous descriptors for key properties and giant composition space, the high-throughput computational method is quite expensive. In contrast, based on known data, proper machine learning may be trained and then used to search for new candidate AFE materials with desirable properties. The phase stability and electrical properties of AFEs may be predicted by finding important descriptors such as Pauling's rules, average ionic radius, electronegativity, and the number of valence electrons. First-principles molecular dynamics (FPMD) simulations allow calculations of generalized complex susceptibilities, which have proven to be powerful tools for examining the dynamics of the AFE phase transition in PbZrO₃ [213, 214]. The kinetics model of the AFE-FE phase transition in lead-free AFEs will also be of future interest. Moriwake et al. studied the polarization switching mechanism in AgNbO₃ using FPMD simulations within the framework of DFT [215], revealing that local polarization switching only takes place parallel to the short axes of the oxygen octahedron and anisotropy in polarization. It has been shown that the phase-field method based on Landau–Devonshire theory is a powerful tool for predicting the formation of domain structures under different boundary conditions, and the structure-property relationship learned from phase-field simulations can be utilized to design high-performance anti-/ferroelectrics [216, 217]. For example, a recent phenomenological phase-field study considering the coupling between the polar mode and the oxygen tilt mode of the AFE phase was performed to understand the morphology of AFE structures, and the formation mechanism of CMS and IMS in perovskite AFEs [217].

5. Summary

The commercialization of AFEs calls for extensive research and development efforts on new AFE materials that are not only highly efficient in energy storage and conversion but also non-toxic and stable. Concomitant changes in the unit cell volume, entropy, and stored charge can be used for applications in some energy-efficient storage and conversion technologies. These technologies exploit the field-induced phase transition between the antipolar AFE ground state and a low-lying FE polar state; the most well-studied functional properties of antiferroelectrics are electric polarization, electric-field-induced strain, and dielectric properties. Over the next few years, an increasing effort is expected to be devoted to the search for new AFE materials with high energy performance. We suggest here a roadmap that may contribute to the development of energy-efficient AFE materials. This roadmap starts with understanding the achievements and challenges of the current and emerging lead-based and lead-free AFE perovskites through more standardized experimental protocols. After gaining an in-depth understanding of the structural origin of antiferroelectricity, the design (including theory-experiment approaches) of new lead-free AFEs will be highly desirable from an environmental perspective. We emphasize that AFE materials can be employed in many other applications such as AFE random access memories [218, 219], memristors [220], above-bandgap photovoltages [221], and topological insulators [209]. Of course, there is still a long way to go before AFEs can be put into real applications for energy storage and conversion applications. Some basic scientific issues yet to be elucidated such as the origin of antiferroelectricity, effective approaches to tailor the relative stability of FE and AFE phases, and the manipulation of the field-induced phase transition between AFE and FE. Fortunately, the development of both experimental and theoretical research tools enables a deeper understanding of antiferroelectrics, and a sustained research effort like that seen in ferroelectrics. Today marks the starting point of a time of further development for AFE materials and their promising applications in the field of energy storage and energy conversion. We expect that the realization of efficient and eco-friendly antiferroelectric energy storage and conversion devices will take place in the near future.

Declaration of competing interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Acknowledgments

This work was supported by the Fundamental Research Funds for the Central Universities (University of Science and Technology Beijing: No. 06500135). Fangping Zhuo acknowledges the Alexander von Humboldt Foundation for financial support. Huimin Qiao acknowledges support from the National Research Foundation of Korea (NRF) grant funded by the Korean government (MSIP; No. 2019R1I1A1A01063888). The computing work is supported by USTB MatCom of Beijing Advanced Innovation Center for Materials Genome Engineering. Jiaming Zhu acknowledges the financial supports of the PolyU Post-Dr Research Grant (No. G-YW5T) from The Hong Kong Polytechnic University.