1 Introduction

There are two distinct patterns of large-scale atmospheric circulation over middle–high latitudes, namely, high-index flow, which has strong zonal westerlies and relatively weak wave perturbations, and low-index flow, which has relatively weak westerlies with large wave amplitudes and usually evolves into blocking (Rossby, 1939; Namias, 1950; Thompson and Wallace, 2001; Li and Wang, 2003; Faranda et al., 2016). Charney and DeVore (1979, hereafter CD) proposed the multiple flow equilibria theory to explain the two distinct flow patterns. They used a low-order (also called “highly truncated”) spectral barotropic channel model and found that multiple equilibrium states may exist in the presence of topographic and thermal forcings. Among the multiple equilibrium states, two equilibrium states of distinct characters, termed high- and low-index flow, were stable. Charney and Straus (1980, hereafter CS) extended CD’s study to a two-layer baroclinic model to investigate the instabilities that produce and feed on multiple equilibrium states. They suggested that topographic instability is merely a triggering mechanism to generate multiple equilibria, and the energy for maintenance of the wave-like equilibria comes from the conversion of mean flow potential energy.

Charney’s pioneering study prompted a great deal of research interest in the low-order spectral model and multiple flow equilibria theory. Zhu and Zhu (1982) and Zhu (1985) used a two-layer low-order spectral model and found that there were some stable equilibrium states with typical characteristics of actual blocking. They emphasized that the zonally asymmetric thermal and topographic forcings and the nonlinearity of flow were the main factors in blocking dynamics.Reinhold and Pierrehumbert (1982, 1985, hereafter RP) extended the model of CS to include synoptic-scale waves and found two distinct weather regime states. They suggested that the wave–wave interactions could transfer the model flow from one regime-equilibrium to another. Legras and Ghil (1985) used a higher-order barotropic spectral spherical model and they reported that the model may exhibit properties of an index cycle. Because Charney’s model was deterministic system, stochastic forcing was added to the model and then the model flow also showed transitions between high- and low-index states (Egger, 1981; Benzi et al., 1984; Sura, 2002). In addition, by using low-order spectral models, some studies explored the physical mechanism of abrupt change in flow patterns over midlatitudes and subtropical region (Li and Luo, 1983; Liu and Tao, 1983; Miao and Ding, 1985; Luo, 1987). Li and Chou (1996, 1997) proved that the joint action of nonlinearity, dissipation, and external forcing was the source of the atmospheric multiple equilibria. Some recent studies used Charney’s multiple flow equilibria theory to demonstrate the roles of the high- and low-index flow patterns in the interdecadal variation of the continental temperature (He et al., 2014, 2018; Huang et al., 2016, 2017a, b ). Similar models and studies have been discussed in many other papers (Tung and Rosenthal, 1985; Cai and Mak, 1987; Cehelsky and Tung, 1987; Christensen and Wiin-Nielsen, 1996; Koo and Ghil, 2002; Crommelin et al., 2004; etc.) and in some review articles (De Swart, 1988; Li and Chou, 2003).

Although many studies have followed Charney’s work, a shortcoming of the classic Charney’s model is that the “thermal forcing” (i.e., the radiative equilibrium temperature field in CS and the direct forcing of the flow wave field in CD) is always artificially specified. Therefore, the feedback from the atmospheric flow to the “thermal forcing” is absent, in other words, the atmospheric flow in Charney’s model cannot change the thermal distribution, but rather, can only be adapted to the “thermal forcing”. To some extent, the effects of “thermal forcing” on large-scale atmospheric motions in Charney’s model may be unrealistic. To overcome this shortcoming, a new model coupling the flow and temperature fields should be developed. The coupled model should include some essential physical processes, for instance, the horizontally inhomogeneous temperature fields give rise to the atmospheric motions, and in turn, the atmospheric motions change the distribution of temperature. Then to compensate for the energy dissipation due to the friction, the external energy input should be the uneven solar heating, which is zonally symmetric and decreases from low to high latitudes. This simple coupled model is established in this paper. We find that there are still multiple equilibria with distinct wave amplitude (i.e., the high- and low-index flow) when the topography is present. Interestingly, the lower layer streamfunction of some stable equilibria is either in phase or out of phase with the topography, i.e., their lower layer ridges or troughs are over the mountains, we call them ridge- or trough-type equilibria. The multiple wave phase equilibria associated with ridge- and trough-types are more prominent than the multiple wave amplitude equilibria associated with high- and low-index types in our coupled model. Besides, the multiple wave phase equilibria are more remarkable in the coupled model than in the uncoupled model. However, compared to multiple wave amplitude equilibria, there have been few studies of multiple wave phase equilibria.

In this study, the multiple wave phase equilibria associated with ridge- and trough-types and the multiple wave amplitude equilibria associated with high- and low-index types are both investigated based on a low-order coupled land–atmosphere model. The paper is organized as follows. The low-order coupled land–atmosphere model is described in Section 2. Our model is similar to the low-order coupled ocean–atmosphere model of Vannitsem et al. (2015). The greatest difference between the two models is that the underlying surface is the land with ideal sinusoidal topography in our model. In Section 3, we present the multiple equilibrium solutions and their stabilities. In Section 4, we explore the role of the land–atmosphere coupling in the existence and properties of equilibrium states. In Section 5, we investigate the ridge- and trough-type equilibria and wave phase. In Section 6, we investigate the high- and low-index equilibria and wave amplitude. The discussion and conclusions are presented in Section 7.

2 Model

Similar to CS, the atmospheric model is a two-layer quasi-geostrophic flow confined to a periodic $\beta $ plane channel with zonal walls at $y = 0$ and $\pi L$ . The equations in pressure coordinates are:

$\frac{\partial }{{\partial t}}({\nabla ^2}{\psi ^1}) + J({\psi ^1}, {\nabla ^2}{\psi ^1}) + \beta \frac{{\partial {\psi ^1}}}{{\partial x}} = - {k'_d}{\nabla ^2}({\psi ^1} - {\psi ^3}) + \frac{{{f_0}}}{{\Delta p}}\omega, $

(1)

$\begin{split} & \frac{\partial }{{\partial t}}({\nabla ^2}{\psi ^3}) + J\left({\psi ^3}, {\nabla ^2}{\psi ^3} + {f_0}\frac{h}{H}\right) + \beta \frac{{\partial {\psi ^3}}}{{\partial x}} \\ &\quad \quad = {k'_d}{\nabla ^2}({\psi ^1} - {\psi ^3}) - \frac{{{f_0}}}{{\Delta p}}\omega - {k_d}{\nabla ^2}{\psi ^3}, \quad\quad\quad\quad\quad\quad \end{split}$

(2)

where $x$ and $y$ are eastward and northward coordinates, respectively; $t$ is time, ${\nabla ^2}$ is the horizontal Laplace operator, $J$ is the Jacobian operator; ${\psi ^1}$ and ${\psi ^3}$ are the geostrophic streamfunction fields at ${p_1} = 250$ and ${p_3} = 750$ hPa, respectively; $\omega = {{{\rm d}p}/{{\rm d}t}}$ is the vertical velocity; ${f_0}$ is the Coriolis parameter at a central latitude ${\phi _0} = 45^\circ N$ , with $\beta = {{{\rm d}f}/{{\rm d}y}}$ as its meridional gradient; $\Delta p = 500$ hPa is the pressure difference between the two layers; $H$ is mean depth of each layer; $h(x, y)$ is the lower boundary topographic height, and we assume that $h \ll H$ . The constants ${k_d}$ and ${k'_d}$ multiply the surface friction term and the internal friction between layers, respectively.

We define

$\psi = ({\psi ^1} + {\psi ^3})/2,\quad \theta = ({\psi ^1} - {\psi ^3})/2, $

(3)

then the atmospheric motion equations become the following:

$\begin{split} \!\!\! \frac{\partial }{{\partial t}}({\nabla ^2}\psi) & \!+\! J(\psi, {\nabla ^2}\psi) \!+\! J(\theta, {\nabla ^2}\theta) \!+\! \beta \frac{{\partial \psi }}{{\partial x}}\!=\! - 0.5\, J\left(\psi, {f_0}\frac{h}{H}\right)\\ & \!+\! 0.5\, J\left(\theta, {f_0}\frac{h}{H}\right) - 0.5\, {k_d}{\nabla ^2}(\psi - \theta),\end{split}$

(4)

$\begin{split} \frac{\partial }{{\partial t}}({\nabla ^2}\theta) & + J(\psi, {\nabla ^2}\theta) + J(\theta, {\nabla ^2}\psi) + \beta \frac{{\partial \theta }}{{\partial x}}= 0.5\, J\left(\psi, {f_0}\frac{h}{H}\right)\\ & - 0.5\, J\left(\theta, {f_0}\frac{h}{H}\right) + 0.5\, {k_d}{\nabla ^2}(\psi - \theta) - 2\, {{k\,'}_{\!\!\!\!\!d}}{\nabla ^2}\theta + \frac{{{f_0}}}{{\Delta p}}\omega .\end{split} $

(5)

In the equation of temperature of the baroclinic atmosphere, a radiative and heat flux scheme is incorporated reflecting the exchanges in energy among the land, atmosphere, and space (Barsugli et al., 1998; Vannitsem et al., 2015; De Cruz et al., 2016):

$\begin{split}{\gamma _{\rm{a}}} & \left(\frac{{\partial {T_{\rm{a}}}}}{{\partial t}} + J(\psi, {T_{\rm{a}}}) - \sigma \omega \frac{p}{R}\right) = - \lambda ({T_{\rm{a}}} - {T_{\rm{g}}}) \\ & + {\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{\rm{g}}^4 - 2{\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{\rm{a}}^4 + {R_{\rm{a}}},\end{split}$

(6)

where ${T_{\rm{a}}}$ and ${T_{\rm{g}}}$ are atmospheric and land temperature, respectively; $\sigma $ is the static stability with $p$ as the pressure; $R$ is the gas constant for dry air; $\omega $ is the vertical velocity in pressure coordinates; ${\gamma _{\rm{a}}}$ is the heat capacity of the atmosphere for a 1000-hPa deep column; $\lambda $ is the heat transfer coefficient between the land and atmosphere; ${\sigma _{\rm{B}}}$ is the Stefan-Boltzmann constant and ${\varepsilon _{\rm{a}}}$ is the longwave emissivity of the atmosphere. ${\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{\rm{g}}^4$ is the longwave radiation emitted from the land that is absorbed by the atmosphere; $ - 2{\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{\rm{a}}^4$ is the longwave radiation emitted from the atmosphere to the land and space; ${R_{\rm{a}}}$ is the shortwave solar radiation directly absorbed by the atmosphere.

The land temperature equation is similar to the atmospheric temperature equation as

${\gamma _{\rm{g}}}\frac{{\partial {T_{\rm{g}}}}}{{\partial t}} = - \lambda ({T_{\rm{g}}} - {T_{\rm{a}}}) - {\sigma _{\rm{B}}}T_{\rm{g}}^4 + {\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{\rm{a}}^4 + {R_{\rm{g}}}, $

(7)

where ${\gamma _{\rm{g}}}$ is the heat capacity of the active layer of the land for a mean thickness of 10 m (Monin, 1986); $ - {\sigma _{\rm{B}}}T_{\rm{g}}^4$ is the longwave radiation emitted from the land; ${\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{\rm{a}}^4$ is the longwave radiation emitted from the atmosphere absorbed by the land; ${R_{\rm{g}}}$ is the shortwave solar radiation absorbed by the land.

Similar to Vannitsem et al. (2015), the quartic terms in the radiative fluxes are linearized. The details of this linearization are described in Appendix A.

The system of equations is closed by the thermal wind relation:

$\theta = \frac{R}{{2{f_0}}}\ln \left(\frac{{{p_3}}}{{{p_1}}}\right){T_{\rm{a}}} \approx \frac{R}{{2{f_0}}}{T_{\rm{a}}}.$

(8)

Let $x$ and $y$ be scaled by $L$ , $t$ by $f_0^{ - 1}$ , $\psi $ and $\theta $ by ${L^2}{f_0}$ , ${T_{\rm{a}}}$ and ${T_{\rm{g}}}$ by ${{{L^2}f_0^2}/R}$ , and let $h' = {h/H}$ , $\omega ' = {\omega /{({f_0}\Delta p}})$ , $\beta ' = {{\beta L}/{{f_0}}}$ , $2k = {{{k_d}}/{{f_0}}}$ , ${k^\prime } = {{{k_d}^{\!\!\!\!\prime} }/{{f_0}}}$ , $\sigma ' = {{\sigma {{(\Delta p)}^2}}/{(2{L^2}}}f_0^2)$ .

Other nondimensional coefficients are

$\left. \begin{aligned}& {{R\,'}_{\rm{\!\!\!\!g}}} = {{{R_{\rm{g}}}R}/{({\gamma _{\rm{g}}}f_0^3{L^2})}}, \quad {R_{\rm{a}}}^{\!\!\!\!\prime} = {{{R_{\rm{a}}}R}/{(2{\gamma _{\rm{a}}}f_0^3{L^2})}} \\& {{\lambda \,'}_{\rm{\!\!\!\!g}}} = {\lambda /{({\gamma _{\rm{g}}}}}{f_0}), \quad {{\lambda\, '}_{\!\!\!\!\rm{a}}} = {\lambda /{({\gamma _{\rm{a}}}}}{f_0}) \\&{\sigma _{\rm B, a}} = {{8{\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{\rm a, 0}^3}/{({\gamma _{\rm{g}}}{f_0})}}, \quad {\sigma _{\rm B, g}} = {{4{\sigma _{\rm{B}}}T_{\rm g, 0}^3}/{({\gamma _{\rm{g}}}{f_0})}} \\&{S_{\rm B, a}} = 8{\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}{{T_{\rm a, 0}^3}/{({\gamma _{\rm{a}}}{f_0})}}, \quad {S_{\rm B, g}} = {{4{\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{\rm g, 0}^3}/{(2{\gamma _{\rm{a}}}{f_0})}}\end{aligned} \right\}.$

(9)

We obtain the nondimensional equations of the model as

$\begin{split}\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\!\! \frac{\partial }{{\partial t'}}({\nabla ^2}\psi ') + J(\psi ', {\nabla ^2}\psi ') + J(\theta ', {\nabla ^2}\theta ') + \beta '\frac{{\partial \psi '}}{{\partial x'}} \\= - 0.5J(\psi ', h') + 0.5J(\theta ', h') - k{\nabla ^2}(\psi ' - \theta '), \end{split} \quad\quad\quad\;\;\; $

(10)

$\begin{split} & \frac{\partial }{{\partial t'}}({\nabla ^2}\theta ') + J(\psi ', {\nabla ^2}\theta ') + J(\theta ', {\nabla ^2}\psi ') + \beta '\frac{{\partial \theta '}}{{\partial x'}} \\ &\quad\quad = 0.5J(\psi ', h') \!-\! 0.5J(\theta ', h')\; \!+\! k{\nabla ^2}(\psi ' \!-\! \theta ') \!-\! 2k'{\nabla ^2}\theta ' \!+\! \omega ' \end{split}, $

(11)

$ \begin{split}\!\!\!\!\!\!\!\!\!\!\!\!\! & \frac{{\partial \theta '}}{{\partial t'}} + J(\psi ', \theta ') - \sigma '\omega ' = - {\lambda '_{\rm{a}}}(\theta ' - 0.5\delta {T_{\rm{g}}}^{\!\!\!\!\!\prime}\;) \\ &\quad\quad + {S_{{\rm B}, {\rm g}}}\delta {T_{\rm{g}}}^{\!\!\!\!\!\prime}\; - {S_{\rm B, a}}\theta ' + \delta {R'_{\rm{a}}}, \;\;\;\quad\quad\quad\quad\quad\quad\quad\quad\quad\quad\quad \end{split}$

(12)

$\frac{{\partial \delta {{T'}_{\!\!\!\!\rm{g}}}}}{{\partial t'}} = - {\lambda _{\rm{g}}}^{\!\!\!\!\!\prime} (\delta {T'_{\!\!\rm{g}}} - 2\theta ') - {\sigma _{\rm B, g}}\delta {T'_{\rm{g}}} + {\sigma _{\rm B, a}}\theta ' + \delta {R'_{\rm{g}}}.$

(13)

Note that Eqs. (12) and (13) have been linearized. The nondimensional atmospheric temperature, ${T'_{\rm{a}}}$ , is already replaced by nondimensional $\theta '$ in Eq. (12) according to Eq. (8); $\delta {T'_{\rm{g}}}$ is the nondimensional land temperature anomaly; $\delta {R'_{\rm{a}}}$ and $\delta {R'_{\rm{g}}}$ are the nondimensional meridional differential shortwave solar radiation absorbed by the atmosphere and the land, respectively. All of the variables are now dimensionless unless otherwise specified. Hereafter, we omit the primes of the nondimensional variables $\psi '$ , $\theta '$ , $\delta {T'_{\rm{g}}}$ and $x'$ , $y'$ , $t'$ for simplicity, but others are retained to avoid confusion.

We follow the work of CS, and truncate the expansions for $\psi $ , $\theta $ , $\delta {T_{\rm{g}}}$ , $\omega '$ , and $h'$ as:

$\left. \begin{aligned}& \psi = \sum\limits_{i = 1}^3 {{\psi _i}} {F_i}, \quad \theta = \sum\limits_{i = 1}^3 {{\theta _i}} {F_i}, \quad \delta {T_{\rm{g}}} = \sum\limits_{i = 1}^3 {{T_{{\rm{g}}, i}}} {F_i} \\& \omega ' = \sum\limits_{i = 1}^3 {{\omega _i}} {F_i}, \quad h' = {h_2}{F_2}\end{aligned} \right\}.$

(14)

We choose

$\left. \begin{aligned}& {F_1} = \sqrt 2 \cos y \\& {F_2} = 2\cos (nx)\sin y \\& {F_3} = 2\sin (nx)\sin y\end{aligned} \right\}.$

(15)

Here, the zonal wavenumber $n$ may be chosen freely, and it is related to the planetary zonal wavenumber $m = {{na\cos ({\phi _0})}/{L = 2.83n}}$ , where $a$ is the radius of the earth. Note that the channel is periodic in $x$ direction over the scale ${{2\pi }/n}$ .

The dimensional boundary topography is given by

$h = H{h_2}{F_2} = 2H{h_2}\cos ({{nx}/L})\sin ({y/L}).$

(16)

In our model, we set ${h_2} = 0.1$ , and thus, the dimensional amplitude of the topography is fixed at $0.2H = $ 1.46 km.

The nondimensional meridional differential shortwave solar radiation absorbed by the land and the atmosphere are given by

$\delta {R'_{\rm{g}}} = {C_{\rm{g}}}^{\!\!\!\! \prime} {F_1}, \quad \delta {R'_{\rm{a}}} = {C'_{\rm{a}}}{F_1}, $

(17)

where ${C'_{\rm{g}}} = {{{C_{\rm{g}}}R}/{({\gamma _{\rm{g}}}f_0^3{L^2})}}$ and ${C_{\rm{a}}}^{\!\!\!\! \prime} = {{C_{\rm{a}}}R}/$ ${(2{\gamma _{\rm{a}}}f_0^3{L^2})}$ . The dimensional forms are

$\delta {R_{\rm{g}}} = \sqrt 2 {C_{\rm{g}}}\cos ({y/L}), \quad \delta {R_{\rm{a}}} = \sqrt 2 {C_{\rm{a}}}\cos ({y/L}), $

(18)

and we set ${C_{\rm{a}}} = 0.4{C_{\rm{g}}}$ . Thus, the dimensional meridional differences in solar heating absorbed by the land and atmosphere between the southern wall $y = 0$ and the northern wall $y = \pi L$ of the channel are $2\sqrt 2 {C_{\rm{g}}}$ and $2\sqrt 2 {C_{\rm{a}}}$ , respectively. The variable C_g is a dimensional parameter, which is an indicator of the meridional difference in solar heating absorbed by the land between the walls, and it is the most important varying parameter in our coupled model. Based on the observations, a typical value of C_g for boreal summer is 15 W m^–2, that for boreal winter is 55 W m^–2, and that for spring or autumn is 35 W m^–2.

We can obtain 12 spectral equations and by eliminating $\omega '$ , the number of equations can be reduced to 9. The final spectral equations are given as follows:

${\dot \psi _1} = - k({\psi _1} - {\theta _1}) - c\tilde h({\theta _3} - {\psi _3}), $

(19)

$({n^2} + 1){\dot \psi _2} = - c{n^2}({\psi _1}{\psi _3} + {\theta _1}{\theta _3}) + \beta n{\psi _3} - {B_1}({\psi _2} - {\theta _2}), $

(20)

$\begin{split} & ({n^2} + 1){\dot \psi _3} = c[{n^2}({\psi _1}{\psi _2} + {\theta _1}{\theta _2}) + \tilde h({\theta _1} - {\psi _1})] \\ &\quad\quad - \beta n{\psi _2} - {B_1}({\psi _3} - {\theta _3}), \end{split}\quad\quad\quad\;\;\;$

(21)

$\begin{split} {C_1}{\dot \theta _1} =& c[{\psi _2}{\theta _3} - {\psi _3}{\theta _2} - \sigma '\tilde h({\psi _3} - {\theta _3})] - {B_3}{\theta _1}\\ & + k\sigma '{\psi _1} - {d_1}{\theta _1} + {d_2}{T_{{\rm g}, 1}} + {C_{\rm{a}}}^{\!\!\!\! \prime} \; ,\end{split}\quad\quad\quad\;\;\;$

(22)

$\begin{split}({n^2} + 1){C_2}{\dot \theta _2} = & c({A_1}{\psi _3}{\theta _1} - {A_2}{\psi _1}{\theta _3}) + \beta n\sigma '{\theta _3} - {B_2}{\theta _2} \\ & + {B_1}\sigma '{\psi _2} - {d_1}{\theta _2} + {d_2}{T_{{\rm {\rm g}}, 2}},\end{split}\;\;\;$

(23)

$\begin{split}({n^2} + 1){C_2}{\dot \theta _3} = & c[{A_2}{\psi _1}{\theta _2} - {A_1}{\psi _2}{\theta _1} + \sigma '\tilde h({\psi _1} - {\theta _1})] \\ & -\beta n\sigma '{\theta _2} - {B_2}{\theta _3} + {B_1}\sigma '{\psi _3} - {d_1}{\theta _3} + {d_2}{T_{{\rm g}, 3}},\end{split}$

(24)

${\dot T_{{\rm g}, 1}} = - {d_3}{T_{{\rm g}, 1}} + {d_4}{\theta _1} + {C'_{\rm{g}}}, $

(25)

${\dot T_{{\rm g}, 2}} = - {d_3}{T_{{\rm g}, 2}} + {d_4}{\theta _2}, $

(26)

$ {\dot T_{{\rm g}, 3}} = - {d_3}{T_{{\rm g}, 3}} + {d_4}{\theta _3}, $

(27)

where the coefficients used here are

$\left. \begin{aligned}& c = \frac{{8\sqrt 2 n}}{{3\pi }}, \quad \quad \, \tilde h = \frac{{{h_2}}}{2} \\& {d_1} = {{\lambda '}_{\!\!\!\! \rm{a}}} + {S_{\rm B, a}}, \quad \, {d_2} = {{{{\lambda '}_{\!\!\!\! \rm{a}}}}/2} + {S_{\rm B, g}} \\& {d_3} = {{\lambda '}_{\!\!\!\! \rm{g}}} + {\sigma _{\rm B, g}}, \quad {d_4} = 2{{\lambda '}_{\!\!\!\! \rm{g}}} + {\sigma _{\rm B, a}} \\& {A_1} = 1 - \sigma '{n^2}, \quad \;\, {A_2} = 1 + \sigma '{n^2} \\& {B_1} = ({n^2} + 1)k, \quad {B_3} = (2k' + k)\sigma ' \\& {B_2} = ({n^2} + 1)(2k' + k)\sigma ' \\& {C_1} = \sigma ' + 1, \quad \;\;\;\;\;{C_2} = \sigma ' + \frac{1}{{{n^2} + 1}} \\ \end{aligned} \right\}.$

(28)

3 Multiple equilibrium solutions and their stabilities

In this section, we will show the equilibrium solutions (i.e., stationary solutions) and their stabilities of the simple coupled land–atmosphere system Eqs. (19)–(27).

We set all of the time derivatives and wave components to zero in Eqs. (19)–(27), and then obtain a specific equilibrium state:

$\left. \begin{aligned}& {{\bar \theta }_1} = \frac{{{D_2}}}{{2k'\sigma ' - {D_1}}} \\&{{\bar \psi }_1} = {{\bar \theta }_1} \\& {{\bar T}_{{\rm{g}}, 1}} = \frac{{{d_4}{{\bar \theta }_1} + {{C'}_{\rm{\!\!\!\!g}}}}}{{{d_3}}} \end{aligned} \right\}, $

(29)

where

${D_1} = \frac{{{d_2}{d_4}}}{{{d_3}}} - {d_1}, \quad {D_2} = \frac{{{d_2}{{C'}_{\rm{\!\!\!\!g}}}}}{{{d_3}}} + {C'_{\rm{a}}}.$

(30)

This equilibrium state is referred to as “Hadley circulation” in CS. Note that ${\bar \psi _1} = {\bar \theta _1}$ indicates that there is no lower layer zonal flow, i.e., horizontally motionless in the lower layer, while strong westerlies without any meridional perturbations in the upper layer. Note also that the Hadley solution does not interact with the topography.

The method to obtain the general equilibrium solutions of Eqs. (19)–(27) and to determine the stabilities of the equilibrium solutions are shown in Appendix B.

Next, we show the results of calculations of the equilibrium solutions and their stabilities. Similar to the “demonstration case” in RP, we preferentially choose planetary zonal wavenumber $m = 3.7$ ( $n = 1.3$ ) in this study. Wavenumber $m = 6$ is also used for comparison purposes. We set $2k = 0.02$ and $k' = 0.005$ (same as Yoden, 1983), and thus, the dimensional surface and internal frictional dissipation times are 5.6 and 22.4 days, respectively. The values of other dimensional parameters are listed in Table 1.

3.1 Equilibrium solutions

The results of the equilibrium solutions and their stabilities for $m = 3.7$ are shown in Table 2. We will focus on the stable equilibrium states, and the unstable equilibrium states are rarely described.

In Table 2, for a given realistic value of C_g, there may be one (C_g $ \leqslant $ 45 W m^–2) or three (C_g $ > $ 45 W m^–2) equilibrium states, and some of them are stable. Some of the stable states are high-index equilibria, others are low-index equilibria. The criteria of low-index equilibria are that there is at least one closed streamline for low or high pressure center in the upper layer and in addition that the magnitude of streamfunction must be no less than 10⁷ m² s^–1 in the upper layer and 10⁶ m² s^–1 in the lower layer. Those that do not meet the above criteria belong to high-index equilibria. The Hadley solution is a specific high-index equilibria.

For C_g = 20 W m^–2, the only one equilibrium state is stable (see Table 2). All of the wave components of this equilibrium state are zero, so this is Hadley solution. For C_g = 30, 40, and $45$ W m^–2, the results are the same as forC_g = 20 W m^–2.

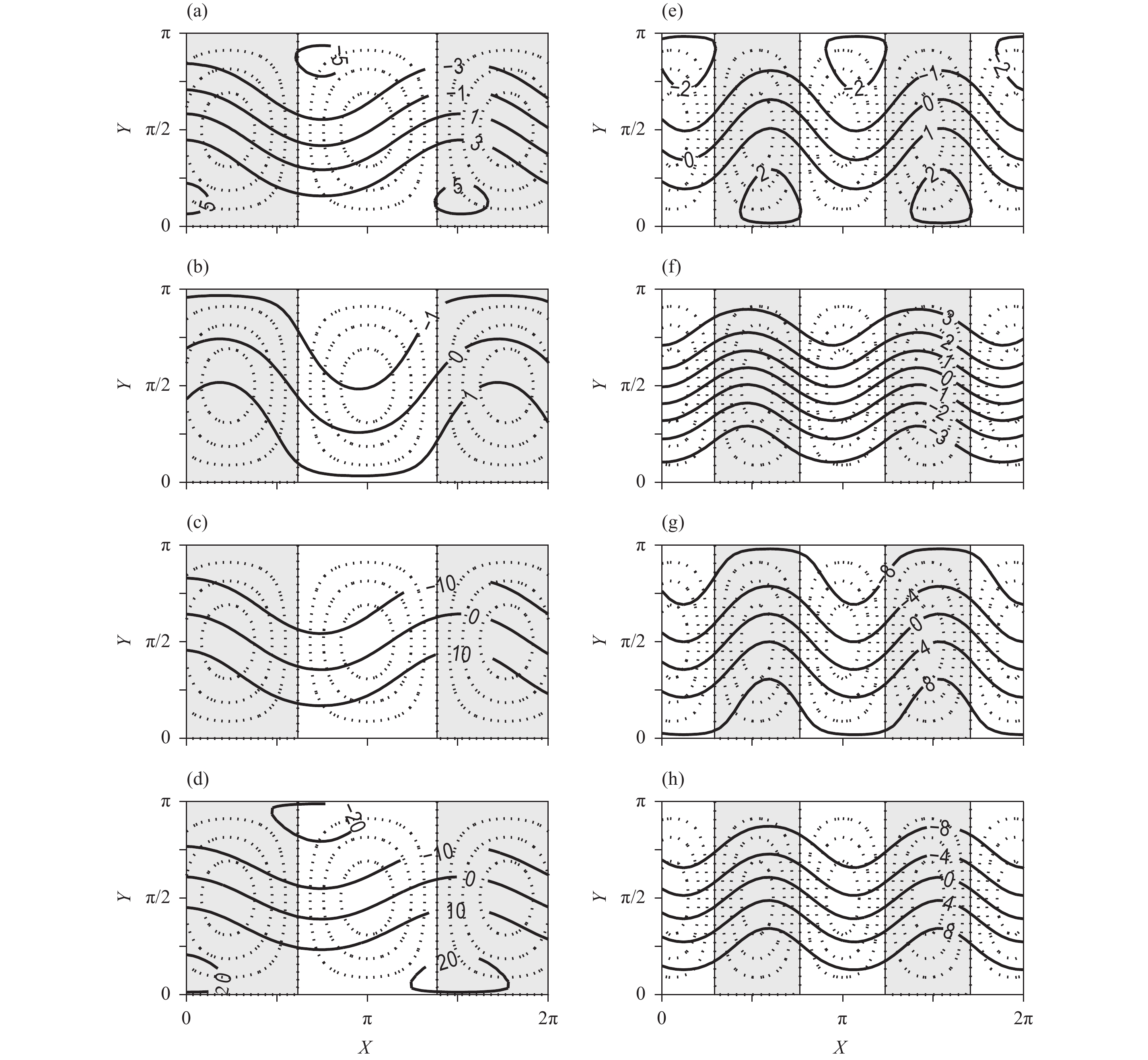
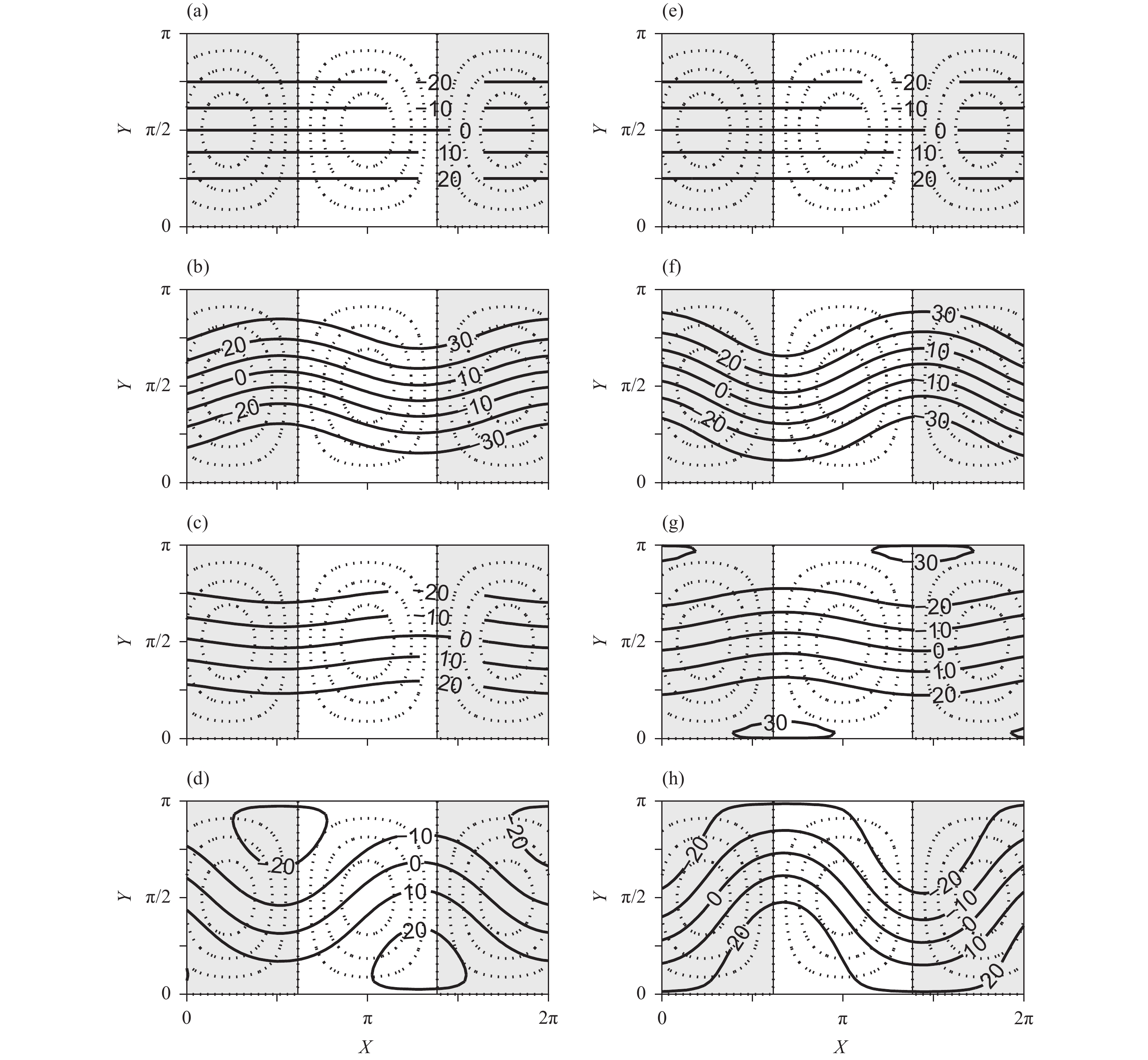
For C_g = 50 W m^–2, there are three equilibrium states and only the last two are stable. Note that the first equilibrium state is the Hadley solution, and now it becomes unstable. The streamfunction and temperature fields of the second and third equilibrium states are illustrated in Fig. 1.

The second and third equilibrium states are both high-index, due to both strong zonal westerlies with weak meridional perturbations in the upper layer (Figs. 1a, e). However, there are wavy easterlies in the lower layer for the second equilibrium state (Fig. 1b) and wavy westerlies in the lower layer for the third equilibrium state (Fig. 1f). The isotherms in both atmospheric and land temperature fields of the two equilibrium states are all quite flat (Figs. 1c, d, g, h) and almost in phase with each upper layer streamfunction field. Both of the two equilibrium states have a characteristic baroclinic structure, i.e., the waves of streamfunction fields displayed westward phase shifts with height. However, they have different wave phases relative to the topography. For the second equilibrium state, its lower layer streamfunction is nearly in phase with the topography, the lower layer converse ridges (anticyclonic flow) are over the mountains (positive topographic heights), lying slightly west of the mountain crests (Fig. 1b), and the upper layer ridges are located to the west side of the mountains (Fig. 1a). We call this a “ridge-type” equilibrium. By contrast, for the third equilibrium state, the lower layer streamfunction is nearly out of phase with the topography, the lower layer low-pressure centers and troughs are over the mountains, also lying slightly west of the mountain crests (Fig. 1f), and the upper layer troughs are located to the west side of the mountains (Fig. 1e). We call this a “trough-type” equilibrium. For simplicity, we refer to the characters of the two equilibrium states as “High 2” and “High 1”, respectively. Here, “High” denotes “high-index”, “2” denotes “ridge-type”, and “1” denotes “trough-type”.

ForC_g = 55 W m^–2, the last two of the three equilibrium states are also stable. The first one is still “High 2” high-index equilibrium, and the second one becomes low-index equilibrium (Table 2). The streamfunction and temperature fields of this low-index equilibrium are illustrated in Fig. 2 (left panel). There are relatively weak westerlies with strong meridional flow in both the upper and lower layer streamfunction fields (Figs. 2a, b), particularly closed streamlines in the former. Note that the magnitude of the streamfunction in Fig. 2b is 10⁶ m² s^–1 and larger than that in Fig. 1b (10⁵ m² s^–1), indicating that the amplitude of meridional perturbations in Fig. 2b are larger than those in Fig. 1b. There are also relatively large meridional perturbations in both the atmospheric and land temperature fields (Figs. 2c, d) and even closed isotherms in the latter. In this low-index equilibrium, the lower layer streamfunction is nearly out of phase with the topography, the lower layer troughs are over the mountains and the upper layer troughs are located on the west side of the mountains, so this is a trough-type equilibrium. We refer to the character of this equilibrium state as “Low 1”, where “Low” denotes “low-index”.

AtC_g = 60 W m^–2, only the third one of the three equilibrium states is stable, and it is “Low 1” equilibrium. The second one becomes unstable. For C_g = 70 and 80 W m^–2, the results are the same as for C_g = 60 W m^–2.

For comparison purposes, we have calculated the equilibrium solutions for wavenumber 6. We find there may be one, three, or five equilibrium states for a given value of C_g (figure omitted). Some of them are stable. Besides the stable “High 1”, “High 2”, and “Low 1” equilibrium, a new stable low-index equilibrium may exist. As illustrated in Fig. 2 (right panel), it has strong meridional perturbations in both the upper layer streamfunction field (Fig. 2e) and temperature fields (Figs. 2g, h). However, there are wavy easterlies in the lower layer streamfunction field (Fig. 2f). Note that the lower layer streamfunction is nearly in phase with the topography, the lower layer converse ridges are over the mountains, and the upper layer ridges are located to the west side of the mountains, so this is a ridge-type equilibrium. We refer to the character of this equilibrium state as “Low 2”.

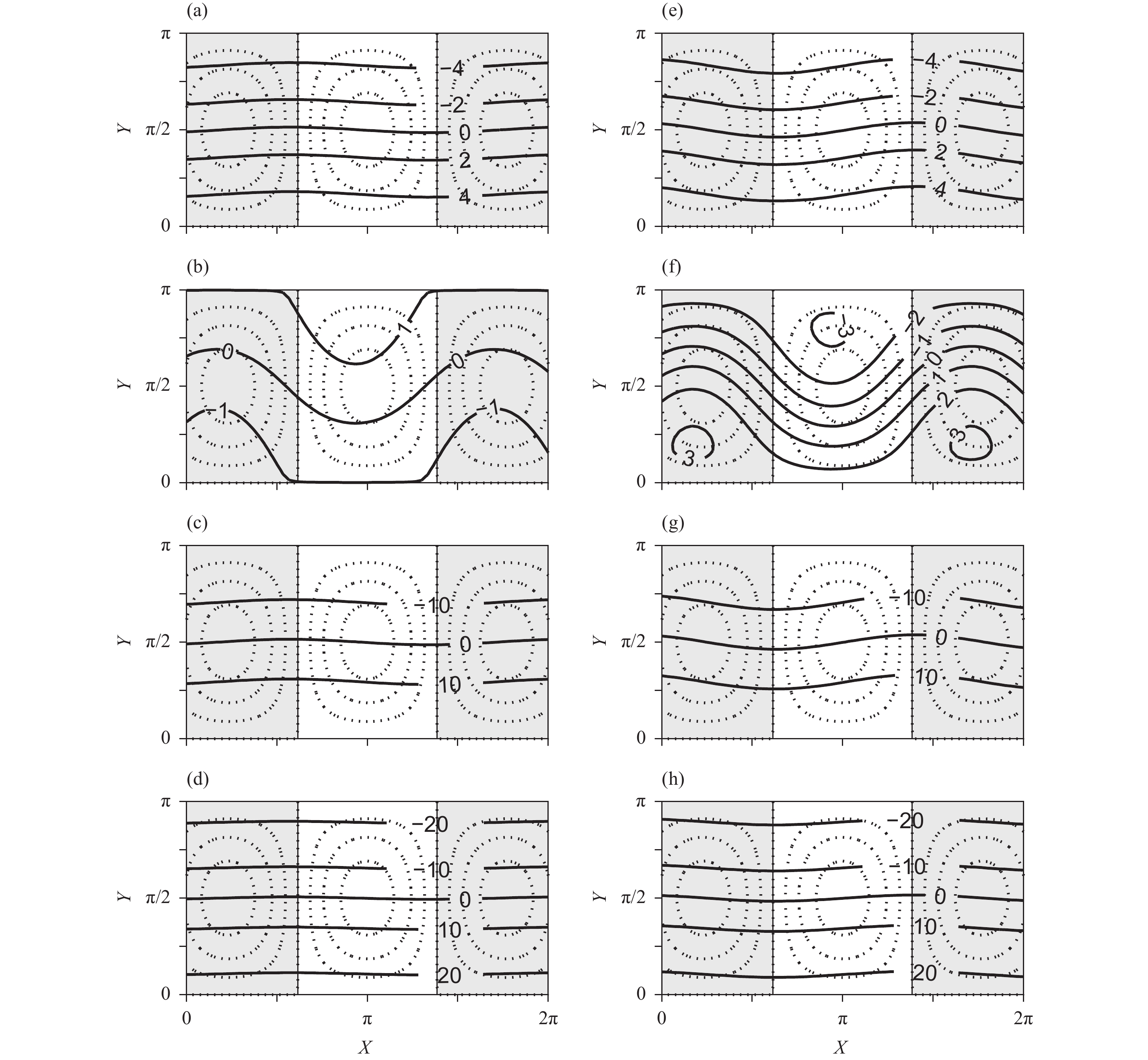
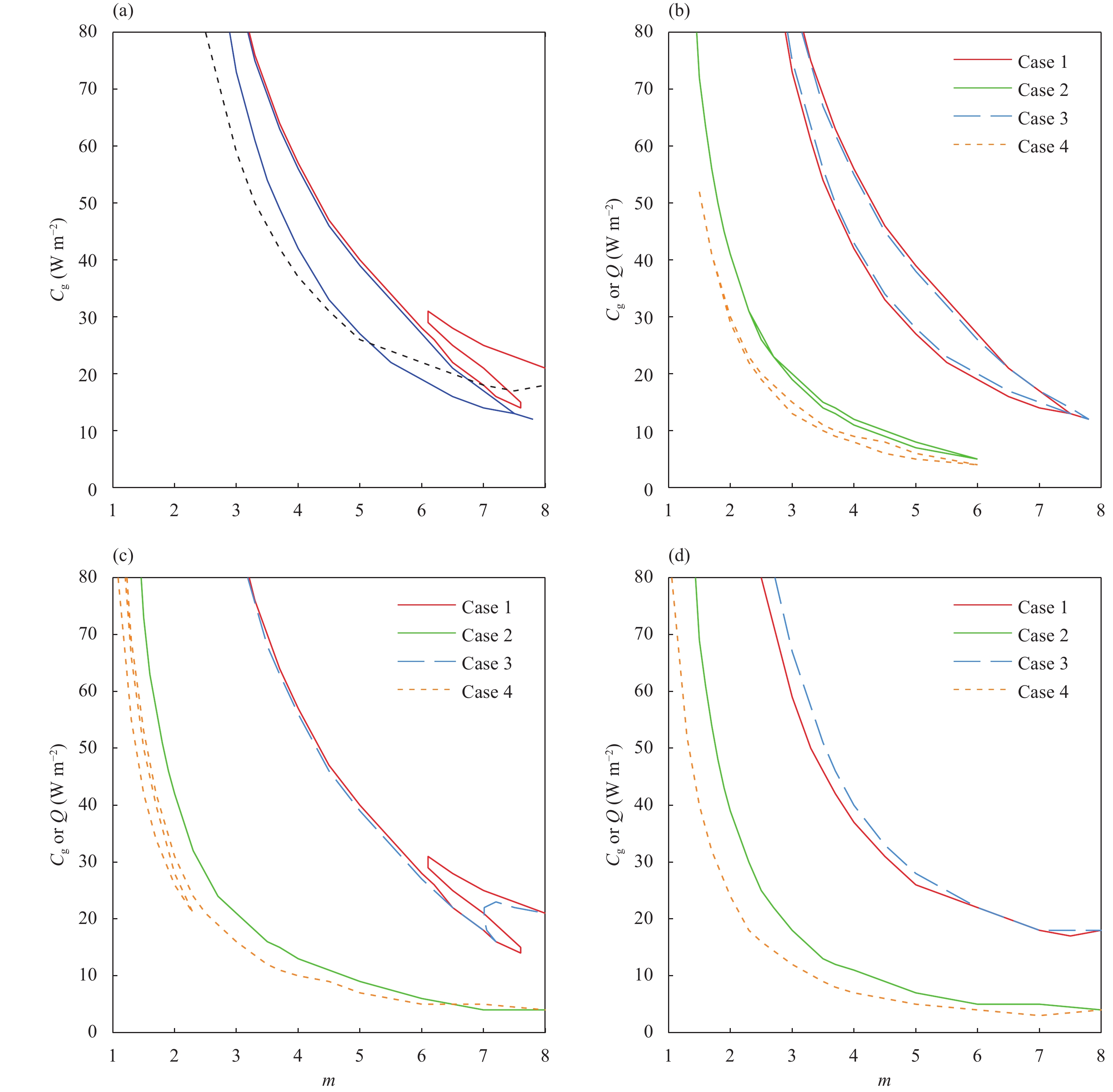
3.2 Bifurcation diagrams

To further demonstrate the multiple equilibrium states and their stabilities for wavenumbers 3.7 and 6, simple bifurcation diagrams are shown in Fig. 3. The zonal component $\psi _1^1$ , the wave component $\psi _2^1$ , and $\psi _3^1$ of the upper layer streamfunction ${\psi ^1}$ are given by $\psi _1^1 = {\psi _1} + {\theta _1}$ , $\psi _2^1 = {\psi _2} + {\theta _2}$ , and $\psi _3^1 = {\psi _3} + {\theta _3}$ , respectively. The equilibr-ium solutions are shown by the 2-W m^–2 interval of the parameterC_g.

For wavenumber 3.7, there are four equilibrium branches (Fig. 3, left panel). For small values of C_g, the Hadley circulation (black) is the only equilibrium and it is stable. As C_g is gradually increased, around C_g = 50 W m^–2, the Hadley circulation loses its stability, and two new equilibria (blue and red) appear. The blue branch represents a trough-type equilibrium, and it is always stable. It includes “High 1” (50 $ \leqslant {C_{\rm{g}}} \leqslant $ 52 W m^–2) and “Low 1” equilibrium ( ${C_{\rm{g}}} \geqslant $ 54 W m^–2). The red branch represents a ridge-type equilibrium, and it includes stable “High 2” equilibrium (50 $ \leqslant {C_{\rm{g}}} \leqslant $ 54 W m^–2). It becomes unstable around C_g = 56 W m^–2, then it disappears and a new equilibria (green) appears when ${C_{\rm{g}}} > $ 56 W m^–2. This green branch equilibrium is always unstable.

For wavenumber 6, there are five equilibrium branches (Fig. 3, right panel). For small values of C_g, the stable Hadley circulation (black) is still the only equilibrium. As C_g is increased to around C_g = 20 W m^–2, the Hadley circulation becomes unstable, and two new equilibria (blue and red) appear. The blue branch represents a trough-type equilibrium and it is always stable. It includes “High 1” (C_g = 20 W m^–2) and “Low 1” equilibrium ( ${C_{\rm{g}}} \geqslant $ 22 W m^–2). The red branch represents a ridge-type equilibrium, and it includes stable “High 2” equilibrium (20 $ \leqslant {C_{\rm{g}}} \leqslant $ 24 W m^–2) and stable “Low 2” equilibrium (26 $ \leqslant {C_{\rm{g}}} \leqslant $ 30 W m^–2). The ridge-type equilibrium is unstable within 32 $ \leqslant {C_{\rm{g}}} \leqslant $ 36 W m^–2 and it disappears when ${C_{\rm{g}}} > $ 36 W m^–2. At around C_g = 26 W m^–2, two more equilibria (green and magenta) appear, and they are both always unstable. The magenta branch disappears when ${C_{\rm{g}}} > $ 36 W m^–2.

The above results indicate that there are multiple equilibrium states with different wave phases and wave amplitudes in the coupled model. For a considerable range of C_g values (50 $ \leqslant {C_{\rm{g}}} \leqslant $ 54 W m^–2 for $m = 3.7$ and 20 $ \leqslant {C_{\rm{g}}} \leqslant $ 30 W m^–2 for $m = 6$ ), two stable equilibria with distinct wave phase relative to the topography, i.e., ridge- and trough-type equilibria, may simultaneously exist (Fig. 3). However, only for a small range of C_g values (C_g = 54 W m^–2 for $m = 3.7$ and 22 $ \leqslant {C_{\rm{g}}} \leqslant $ 24 W m^–2 for $m = 6$ ), two stable equilibria with distinct wave amplitude, i.e., high- and low-index equilibria, may coexist (Fig. 3). Therefore, the multiple wave phase equilibria associated with ridge- and trough-types are more prominent than the multiple wave amplitude equilibria associated with high- and low-index types.

3.3 The origin of the multiple equilibria

The multiple wavelike stationary equilibrium states exist in the model when the topography is present. This is proved in Appendix B.

Figure 5a shows the stability curves of the Hadley circulation in the coupled model. The blue lines enclose the orographically unstable region. In crossing the blue lines from the stable to unstable sides, the variable $\alpha $ (see Appendix B) changes from a negative real value to a positive real value (pitch-fork bifurcation). The red (black dashed) lines separate the baroclinically stable and unstable regions in the presence (absence) of topography. In crossing these lines from the stable to unstable sides, the real part of the complex $\alpha $ changes from negative to positive while the imaginary part is not zero (Hopf bifurcation). The orographic instability of the Hadley circulation is only present when the topography is present. Moreover, there is no overlap between the orographic instability and baroclinic instability in the presence of topography. Besides, just compared the baroclinic stability curves with/without topography, it is seen that the presence of topography stabilizes the Hadley circulation for most wavenumbers.

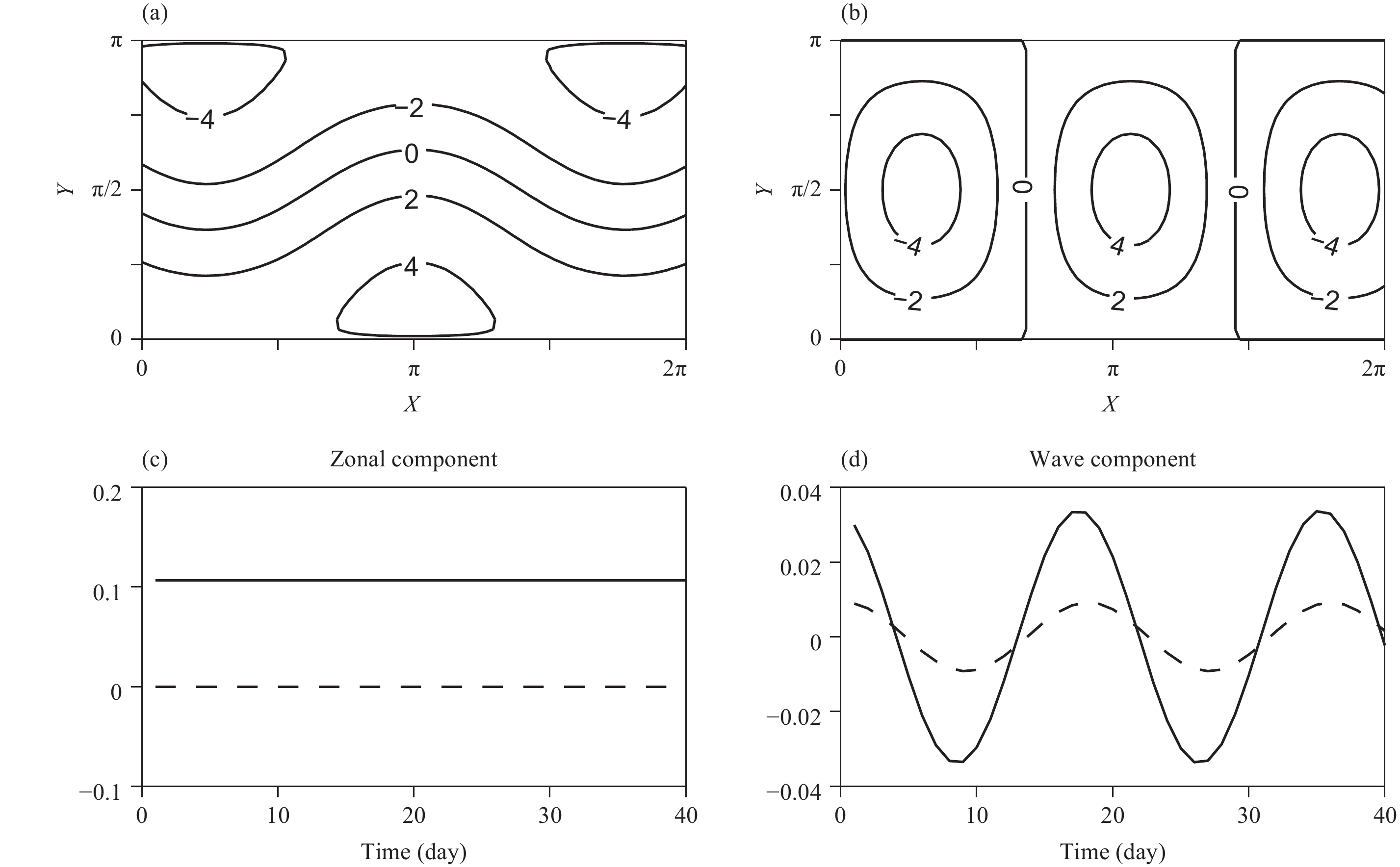
In the absence of topography, there is only the Hadley circulation (see Appendix B) or the traveling wave due to the baroclinic instability of the Hadley circulation (which is a Hopf bifurcation). For example, for $m = 3.7$ at C_g = 50 W m^–2 without the topography, numerical integration starting at arbitrary initial conditions converges to a periodic solution of period 18 days (Fig. 4). Here, the zonal component $\psi _1^3$ and the wave component $\psi _3^3$ of the lower layer streamfunction ${\psi ^3}$ are given by $\psi _1^3 = {\psi _1} - {\theta _1}$ and $\psi _3^3 = {\psi _3} - {\theta _3}$ , respectively. In this periodic solution, there is blocking-like flow in the upper layer streamfunction (Fig. 4a); however, there is no zonal flow (the zonal component $\psi _1^3$ remains zero in Fig. 4c) but wave train in the lower layer streamfunction (Fig. 4b). It is seen that the zonal components of upper and lower layer streamfunction ( $\psi _1^1$ , $\psi _1^3$ ) remain constant (Fig. 4c), whereas the wave components of upper and lower layer streamfunction ( $\psi _3^1$ , $\psi _3^3$ ) evolve periodically with time (Fig. 4d). Particularly, this traveling wave moves westward.

In the presence of topography, there may exist multiple equilibrium states. Compared Fig. 1 with Fig. 4, it seems that due to the presence of topography, the traveling wave becomes two types of stationary waves. In fact, the first bifurcation (Fig. 3, around C_g = 50 W m^–2 for $m = 3.7$ and around C_g = 20 W m^–2 for $m = 6$ ) results from the orographic instability of the Hadley circulation (Fig. 5a, around C_g = 50 W m^–2 for $m = 3.7$ and around C_g = 20 W m^–2 for $m = 6$ ), and it is a (supercritical) pitch-fork bifurcation. This bifurcation is important, because it determines the occurrence and coexistence of the trough- and ridge-type equilibria. Note that the disappearance of the ridge-type equilibria (Fig. 3, around C_g = 56 W m^–2 for $m = 3.7$ and around C_g = 36 W m^–2 for $m = 6$ ) is not related to the occurrence of baroclinic instability of the Hadley circulation (Fig. 5a, around C_g = 64 W m^–2 for $m = 3.7$ and around C_g = 28 W m^–2 for $m = 6$ ).

Therefore, the multiple wave phase equilibria associated with the ridge- and trough-types originate from the orographic instability of the Hadley circulation, which is a pitch-fork bifurcation.

4 The role of the land–atmosphere coupling

In this section, we explore the role of the land–atmosphere coupling in the existence and properties of the equilibrium states.

Four experiments are designed with different diabatic heating terms (see Table 3). For simplicity, here we refer to the coupled land–atmosphere model as Case 1. Equations (6) and (7) indicate that the diabatic heating terms in Case 1 include three terms: heat flux, longwave radiation, and shortwave radiation. For Case 2, we replace all the terms on the right side of Eq. (6) by specified heating ${Q^*}$ ( ${Q^*} = \sqrt 2 Q\cos ({y/L})$ , where $Q$ is a heating parameter that is similar to C_g) and delete the Eq. (7). Thus, Case 2 is just the classic uncoupled model. For Case 3, we delete the heat flux terms (the first terms on the right side) of both Eqs. (6) and (7) (or set $\lambda = 0$ W m^–2 technically). For Case 4, we delete the longwave radiation terms (the second and third terms on the right side) of both Eqs. (6) and (7) (or set ${\sigma _{\rm{B}}} = 0$ W m^–2 K^–4 technically).

4.1 Comparing the stability of the Hadley circulation

Figure 5b compares the orographic instability of the Hadley circulation in the four experiments. Clearly, compared with Case 1, the thresholds of orographic instability in Cases 2 and 4 are both greatly reduced for wavenumbers 1–6. Moreover, the orographically unstable regions in Cases 2 and 4 are both very narrow. Unexpectedly, the orographically unstable regions in Cases 3 and 1 almost completely overlap. Figures 5c and 5d compare the baroclinic instability of the Hadley circulation with and without topography, respectively. Similarly, compared with Case 1, the thresholds of baroclinic instability in Cases 2 and 4 are both greatly reduced for wavenumbers 1–8. However, the baroclinic stability curves in Cases 3 and 1 roughly overlap. The results indicate that compared with the uncoupled model (Case 2), the land–atmosphere coupling (Case 1) greatly stabilizes the Hadley circulation, and this stabilizing effect is primarily attributed to the presence of longwave radiation fluxes, but not the heat fluxes.

In addition, compared with Case 2, Case 4 has lower thresholds for all of the orographic instability and baroclinic instability with and without topography (Figs. 5b–d). It suggests that the presence of heat fluxes extremely destabilizes the Hadley circulation, no matter with or without topography. Nevertheless, in Case 1, which presents both the heat fluxes and the longwave radiation fluxes, the destabilizing effect of the heat fluxes on Hadley circulation is nearly entirely suppressed.

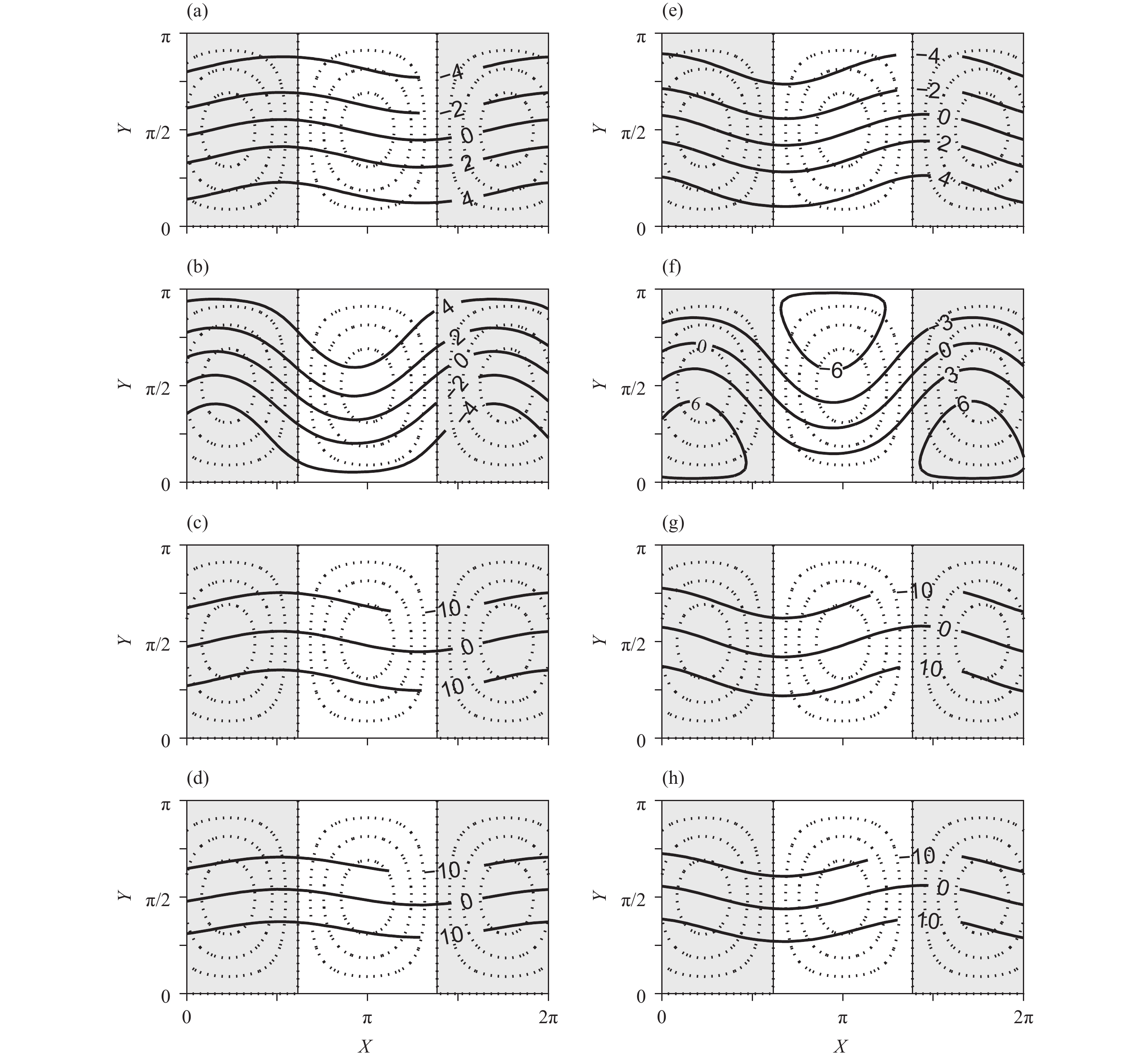
4.2 Comparing the bifurcation

Next, we compare the equilibrium bifurcation in the four experiments. Figures 6a and 6b show the bifurcation diagrams in Case 3 for wavenumber $m = 3.7$ and $m = 6$ , respectively. The equilibrium solutions are shown by the 2-W m^–2 interval of C_g. Even though Cases 3 and 1 have almost overlapped orographic and baroclinic instability curves (Fig. 5), they still have non-negligible difference in the equilibrium bifurcation. Compared with Fig. 3c, it is seen that almost all the ridge-type equilibrium states become unstable for $m = 3.7$ in Case 3 in which the heat flux is absent (Fig. 6a). However, the equilibrium bifurcation has little change for $m = 6$ in Case 3 (Fig. 6b). These suggest that the presence (absence) of heat fluxes more or less stabilizes (destabilizes) the ridge-type equilibrium.

Figures 6c and 6d show the bifurcation diagrams in Cases 2 and 4 for wavenumber $m = 3.7$ , respectively. The equilibrium solutions are shown by the 0.5-W m^–2 interval of $Q$ or C_g. Due to the low thresholds of orographic instability of the Hadley circulation in Cases 2 and 4 (Fig. 5b), the first bifurcation of the Hadley circulation occurs at considerably small heating parameter values (around $Q = 12.5$ W m^–2 in Fig. 6c and around C_g = 9 W m^–2 in Fig. 6d). However, the ridge-type equilibrium subsequently disappears at small heating parameter values (around $Q = 14.0$ W m^–2 in Fig. 6c and around C_g = 10 W m^–2 in Fig. 6d), mainly because the orographically unstable regions in Cases 2 and 4 are very narrow (Fig. 5b). In this case, only for a very small range of heating parameter values, the ridge- and trough-type equilibria may coexist (Figs. 6c, d). By contrast, for a considerable range of heating parameter values, the ridge- and trough-type equilibria may coexist in Case 1 (Fig. 3), mainly due to the fairly wide orographically unstable region (Figs. 5a, b). Therefore, compared with the uncoupled model (Case 2), the multiple wave phase equilibria associated with the ridge- and trough-types in the coupled model (Case 1) is more remarkable.

4.3 Comparing the streamfunction and temperature fields

Here, we compare the streamfunction and temperature fields of equilibrium states in the four experiments for $m = 3.7$ at the same heating parameter values: $Q = 50$ or C_g = 50 W m^–2. In Case 2, there is only one stable equilibrium state (Fig. 6c), with blocking-like large amplitude perturbations in both streamfunction and temperature fields (Fig. 7, left panel). Compared with the two equilibrium states in Case 1 (Fig. 1), it is obvious that the meridional perturbations in streamfunction and temperature fields of the equilibrium state in Case 2 are much stronger. To some extent, this result is attributed to the very low threshold of orographic instability of the Hadley circulation in Case 2 (Fig. 5b). In Case 3, the streamfunction and temperature fields of the two stable equilibrium states (Fig. 8) are very similar to those in Case 1 (Fig. 1), while the meridional perturbations of the lower layer streamfunction (Figs. 8b, f) are apparently weaker than those in Case 1 (Figs. 1b, f), whereas the meridional gradients of land temperature (Figs. 8d, h) are moderately greater than those in Case 1 (Figs. 1d, h). In Case 4, the result is similar to Case 2, but the meridional perturbations in streamfunction and temperature fields (Fig. 7, right panel) are stronger than those in Case 2 (Fig. 7, left panel), probably due to the lower threshold of orographic instability of the Hadley circulation in Case 4 than that in Case 2 (Fig. 5b)

These results indicate that compared with the uncoupled model (Case 2), the land–atmosphere coupling may weaken the atmospheric response to the thermal and topographic forcing, and this weakening effect is mainly contributed by the presence of longwave radiation fluxes. The presence of heat fluxes greatly strengthens the atmospheric response to the thermal and topographic forcing, but in the coupled model which combined the heat fluxes and longwave radiation fluxes, the heat fluxes just strengthen the response of the lower layer flow, and moderately reduce the meridional gradient of the land temperature.

4.4 Comparing the heating fields

To further understand the reason of the different results in the four experiments, we should compare the heating fields in the four experiments.

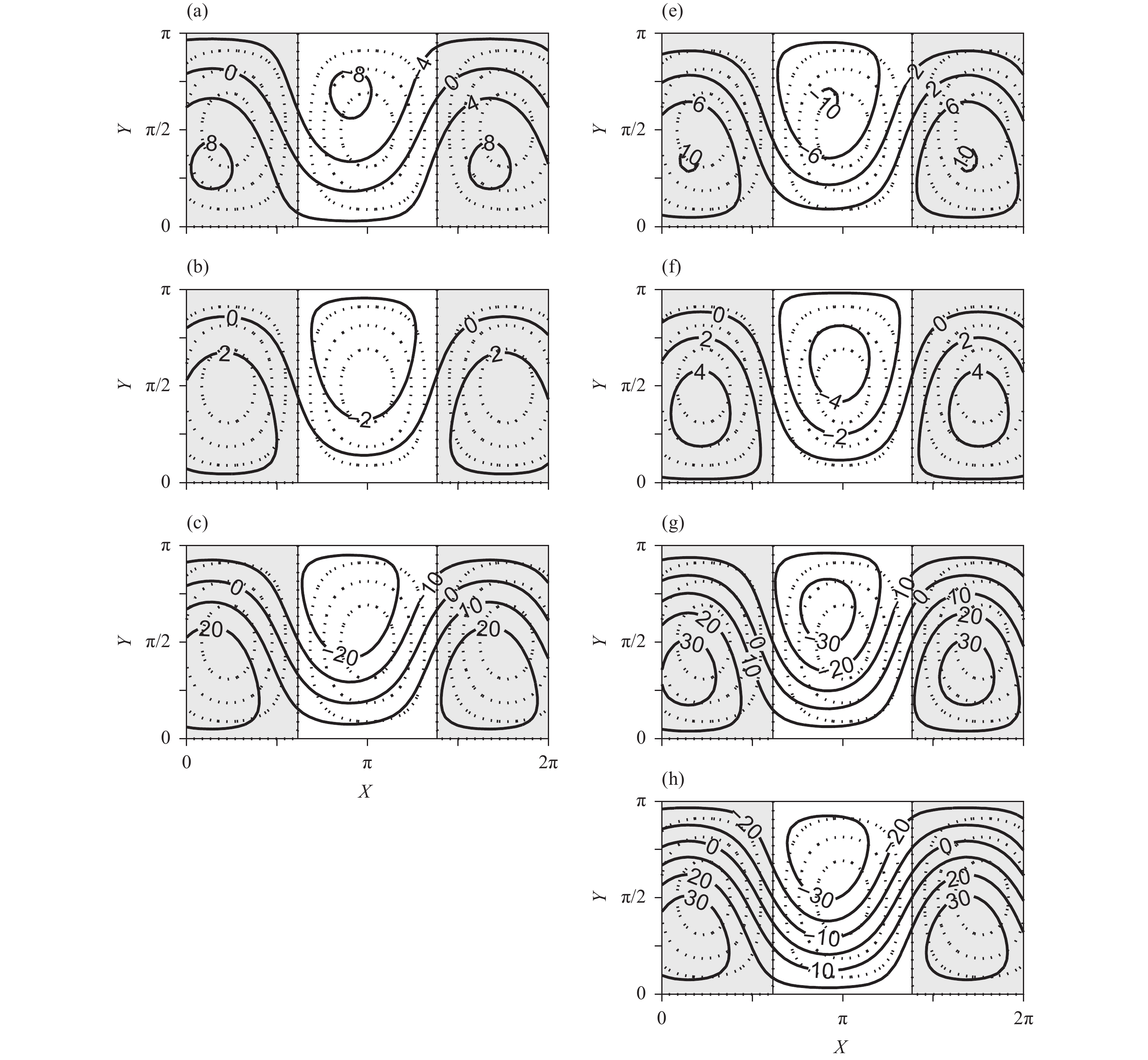
Figure 9 demonstrates the heating fields of the “High 2” and “High 1” equilibrium states shown in Fig. 1 (left and right panels), respectively. The zonally symmetric shortwave radiation fields for the two equilibrium states are identical (Figs. 9a, e). The isolines in all of the longwave radiation fields, the heat flux fields, and the net diabatic heating fields are wave-like, while the wave phases relative to the topography are different. For the “High 2” equilibrium state (Fig. 1, left panel), the “heating ridges” are located on the east side of the mountains (Fig. 9b–d); By contrary, for the “High 1” equilibrium state (Fig. 1, right panel), the “heating ridges” are located on the west side of the mountains (Figs. 9f–h). Note that for the lower layer streamfunction of the two equilibrium states, the ridges (high pressure) are always generated on west side of the “heating ridge”, and the troughs (low pressure) are always generated on east side of the “heat ridges”. Furthermore, it is noteworthy that the longwave radiation fluxes increase from low to high latitudes (Figs. 9b, f); thus, the presence of longwave radiation fluxes reduce the meridional gradient of the net diabatic heating field, resulting in a more stable atmosphere flow. On the contrary, the heat fluxes decrease from low to high latitudes (Figs. 9c, g); thus, the presence of heat fluxes increase the meridional gradient of the net diabatic heating field, resulting in a less stable atmosphere flow.

The net diabatic heating field in Case 2 is zonally symmetric (Fig. 10a). Particularly, the meridional gradient of the net diabatic heating is much greater than that in Case 1 (Figs. 9d, h). The net diabatic heating fields for the “High 2” and “High 1” equilibrium states in Case 3 (Figs. 10c, d) are similar to those in Case 1 (Figs. 9d, h), while the meridional gradients of the net diabatic heating are smaller than those in Case 1. The net diabatic heating field in Case 4 (Fig. 10b) is almost the same as that in Case 2 (Fig. 10a); however, the meridional gradient of the net diabatic heating is greater than that in Case 2. It suggests that compared with the uncoupled model (Case 2), the land–atmosphere coupling reduces the meridional gradient of the net diabatic heating, and this effect is mainly attributed to the presence of longwave radiation fluxes. The presence of heat fluxes greatly increase the meridional gradient of the net diabatic heating. However, in the coupled model that combines the heat fluxes and longwave radiation fluxes, the heat fluxes just moderately increase the meridional gradient of the net diabatic heating.

To sum up, compared with the uncoupled model, the multiple wave phase equilibria associated with the ridge- and trough-types in the coupled model is more remarkable, mainly because the land–atmosphere coupling expands the region of orographic instability of the Hadley circulation. Besides, the land–atmosphere coupling greatly stabilizes the Hadley circulation and weakens the atmospheric response to the thermal and topographic forcing. Particularly, these effects of the land–atmosphere coupling are primarily attributed to the presence of longwave radiation fluxes, which increase from low to high latitudes, reducing the meridional gradient of the net diabatic heating. The presence of heat fluxes more or less modify the effects of longwave radiation fluxes.

5 Ridge- and trough-type equilibria and wave phase

Next, we investigate the wave phases of ridge- and trough-type equilibria relative to the topography. It is clear that the wave components $\psi _2^1$ of the ridge- and trough-type equilibria are both negative (Figs. 3b, e). The negative sign of $\psi _2^1$ denotes that this wave component of upper layer streamfunction of the two types of equilibrium is out of phase with the topography. The wave components $\psi _3^1$ of the ridge- and trough-type equilibria are negative and positive, respectively (Figs. 3c, f). The negative (positive) sign of $\psi _3^1$ denotes that this wave component of upper layer streamfunction of the ridge-type (trough-type) equilibria has a lag (lead) in phase by 90° relative to the mountain crests. Therefore, the upper layer ridges (troughs) of ridge-type (trough-type) equilibria are located to the west side of the mountains.

We have calculated the wave phase of the streamfunction relative to the mountains for wavenumbers 3.7 and 6, and the results are shown in Table 5. The ridge-type (High 2 and Low 2) equilibrium states have lower layer ridges over the mountains, their upper layer ridges are located to the west side of the mountain crests, and they have lower layer easterlies (Mean_U³ is negative, see Table 4 for definition of Mean_U³). The trough-type (High 1 and Low 1) equilibrium states have lower layer troughs over the mountains, their upper layer troughs are located to the west side of the mountain crests, and they have lower layer westerlies (Mean_U³ is positive). Figures 11b and 12b also show that the ridge-type (trough-type) equilibria has lower layer easterlies (westerlies). Therefore, the distinct characters of ridge- and trough-type equilibria are robust.

The above phenomena can roughly be explained by the forced topographic Rossby wave theory. The forced topographic Rossby wave solution based on the barotropic potential vorticity equation (Smith, 1979; Nigam and DeWeaver, 2003; Holton and Hakim, 2012) is given by

$\Psi (x, y) = {\mathop{\rm Re}\nolimits} \left[\frac{{{f_0}h/{H_0}}}{{{{\tilde k}^2} + {{\tilde l}^2} - \beta /\bar u - i\varepsilon ({{\tilde k}^2} + {{\tilde l}^2})/(\bar u\tilde k)}}\right], $

(31)

where $\operatorname{Re} []$ denotes the real part; $\tilde k$ and $\tilde l$ are zonal and meridional wavenumbers, respectively; $h$ is the boundary topography; ${H_0}$ is the height of the homogeneous atmosphere; $\bar u$ is the mean zonal wind speed; $\varepsilon $ is the dissipation factor. In our model, $\tilde k = {n/L}$ , $\tilde l = {1/L}$ , $h = 2H{h_2}\cos (nx/L)\sin (y/L)$ , $\varepsilon = {k_d}$ and we might set ${H_0} = H$ . The boundary topography has little effect on the upper layer flow; therefore, we choose the mean lower layer zonal wind speed, i.e., Mean_U³, as $\bar u$ .

We might write the boundary topography as

$h = {\mathop{\rm Re}\nolimits} \{ 2H{h_2}[\cos (nx/L) + i\sin (nx/L)]\sin (y/L)\}, $

(32)

and set

$\,\,{g_1} = {\tilde k^2} + {\tilde l^2} - {\beta /{\bar u}}, \quad$

(33)

${{{g_2} = \varepsilon ({{\tilde k}^2} + {{\tilde l}^2})}/{(\bar u\tilde k)}}, $

(34)

then Eq. (31) becomes the following:

$\begin{split}&\Psi (x, y) = \operatorname{Re} \{ \frac{{2{f_0}{h_2}}}{{{g_1} - i{g_2}}}[\cos ({{nx}/L}) + i\sin ({{nx}/L})]\sin ({y/L})\} \\ &= \operatorname{Re} \{ \frac{{2{f_0}{h_2}({g_1} + i{g_2})}}{{({g_1} - i{g_2})({g_1} + i{g_2})}}[\cos ({{nx}/L}) + i\sin ({{nx}/L})]\sin ({y/L})\}\\& = \frac{{2{f_0}{h_2}}}{{g_1^2 + g_2^2}}[{g_1}\cos ({{nx}/L}) - {g_2}\sin ({{nx}/L})]\sin ({y/L}).\end{split}$

(35)

Examples of calculated values of g₁ and g₂ are shown in Table 5. The absolute values of g₁ are always much greater than that of g₂. Thus, the wave phase of the streamfunction $\Psi $ relative to the topography mainly depends on the sign of g₁. If g₁ is a positive (negative) value, the streamfunction $\Psi $ should be nearly in (out of) phase with the topography, in other words, ridges (troughs) should be over the mountains. Obviously, the wave phase of the lower layer streamfunction of these equilibrium states is exactly consistent with the wave phase predicted by this rough theory.

In fact, the wave phase of equilibrium states depends on the direction of zonal wind and horizontal scale of the topography. Due to the conservation of potential vorticity, the absolute vorticity is decreased over the mountains. For westerly flow ( $\bar u > 0$ ), in the case ${g_1} > 0$ , i.e., ${\tilde k^2} + {\tilde l^2} > {\beta /{\bar u}}$ [also called “long waves” case (Smith, 1979)], the decrease in absolute vorticity is primarily caused by the generation of negative relative vorticity, then ridges are generated over the mountains; by contrast, in the case ${g_1} < 0$ , i.e., ${\tilde k^2} + {\tilde l^2} < {\beta /{\bar u}}$ [also called “ultralong waves” case (Smith, 1979)], the decrease in absolute vorticity is primarily caused by the decrease in planetary vorticity, which is associated with the southward movement of air parcels, and then troughs are generated over the mountains. However, for easterly flow ( $\bar u < 0$ ), there is always ${\tilde k^2} + {\tilde l^2} > {\beta /{\bar u}}$ , but the decrease in absolute vorticity arises both from the development of negative relative vorticity and from the decrease in planetary vorticity due to the southward motion (Holton and Hakim, 2012); then converse ridges are generated over the mountains. In our model, the occurrence of “long wave” case associated with ridge-type equilibria is purely due to the lower layer easterlies (Table 5 and Figs. 11b, 12b).

In a word, the ridge-type (trough-type) equilibrium states have lower layer ridges (trough) over the mountains and have lower layer easterlies (westerlies). The wave phases of equilibrium states relative to the topography depends on the direction of lower layer zonal wind and horizontal scale of the topography. Further discussion is presented in Section 7.

6 High- and low-index equilibria and wave amplitude

Next, we investigate the high- and low-index equilibria and wave amplitude. To further examine the differences between these two types of equilibrium, we define some dimensional physical variables, as shown in the Table 4. All of the variables are defined over the domain ( $0 \leqslant x \leqslant 2\pi L$ , $0 \leqslant y \leqslant \pi L$ ) of the channel. The amplitude of wave component of the upper layer geopotential height field is defined as

${\rm AH} = \frac{{{L^2}f_0^2}}{{{g_0}}}\sqrt {{{(\psi _2^1)}^2} + {{(\psi _3^1)}^2}} .$

(36)

The amplitude of wave components of the atmospheric and the land temperature fields are defined as

$\!\!\!\!\!\!\!{\rm A}{{\rm T}_{\rm{a}}} = \frac{{2{L^2}f_0^2}}{R}\sqrt {{{({\theta _2})}^2} + {{({\theta _3})}^2}}, $

(37)

${\rm A}{{\rm T}_{\rm{g}}} = \frac{{{L^2}f_0^2}}{R}\sqrt {{{({T_{{\rm g}, 2}})}^2} + {{({T_{{\rm g}, 3}})}^2}}, $

(38)

respectively. These two variables represent the zonal asymmetry of atmospheric and land temperature fields.

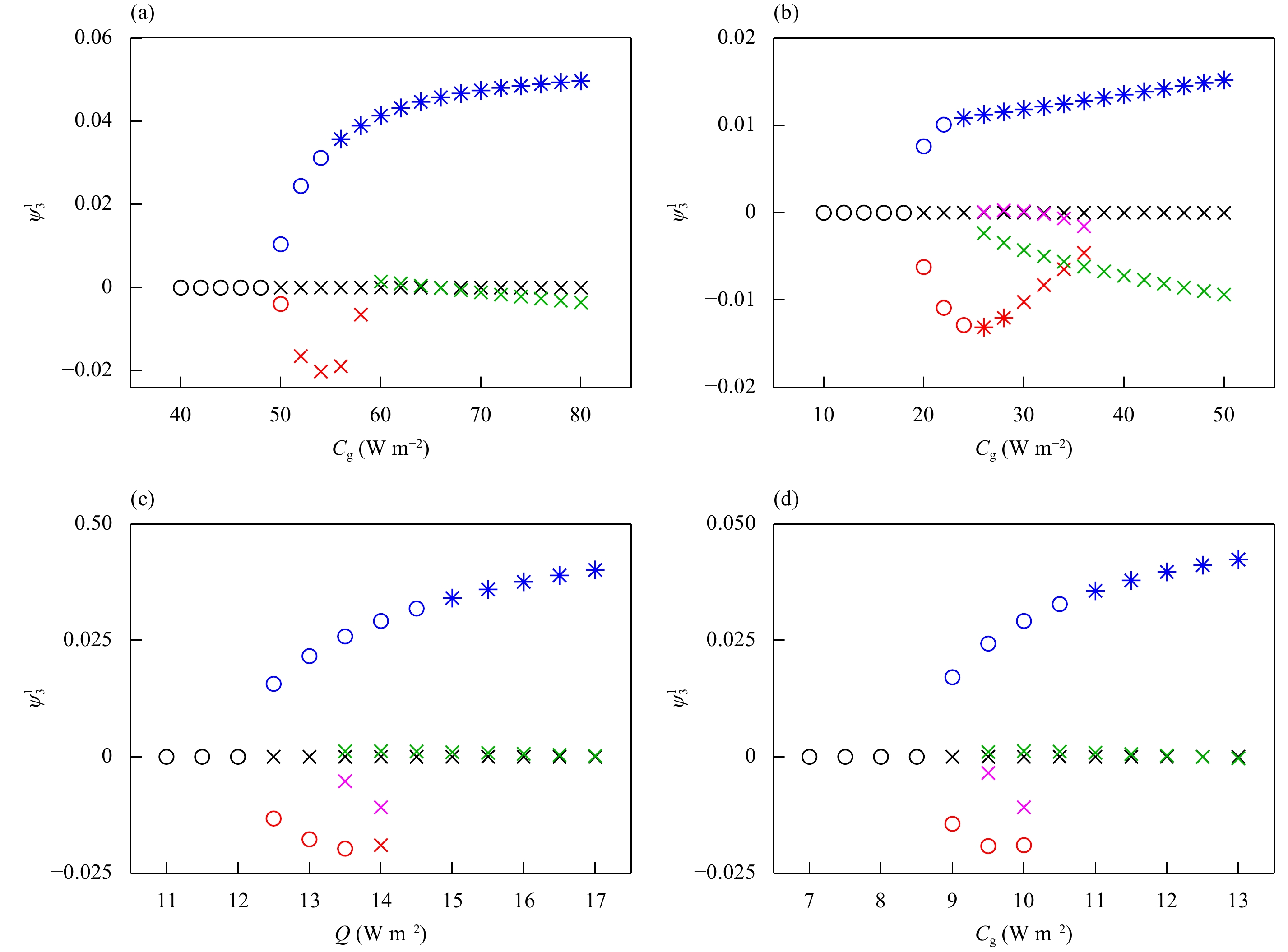
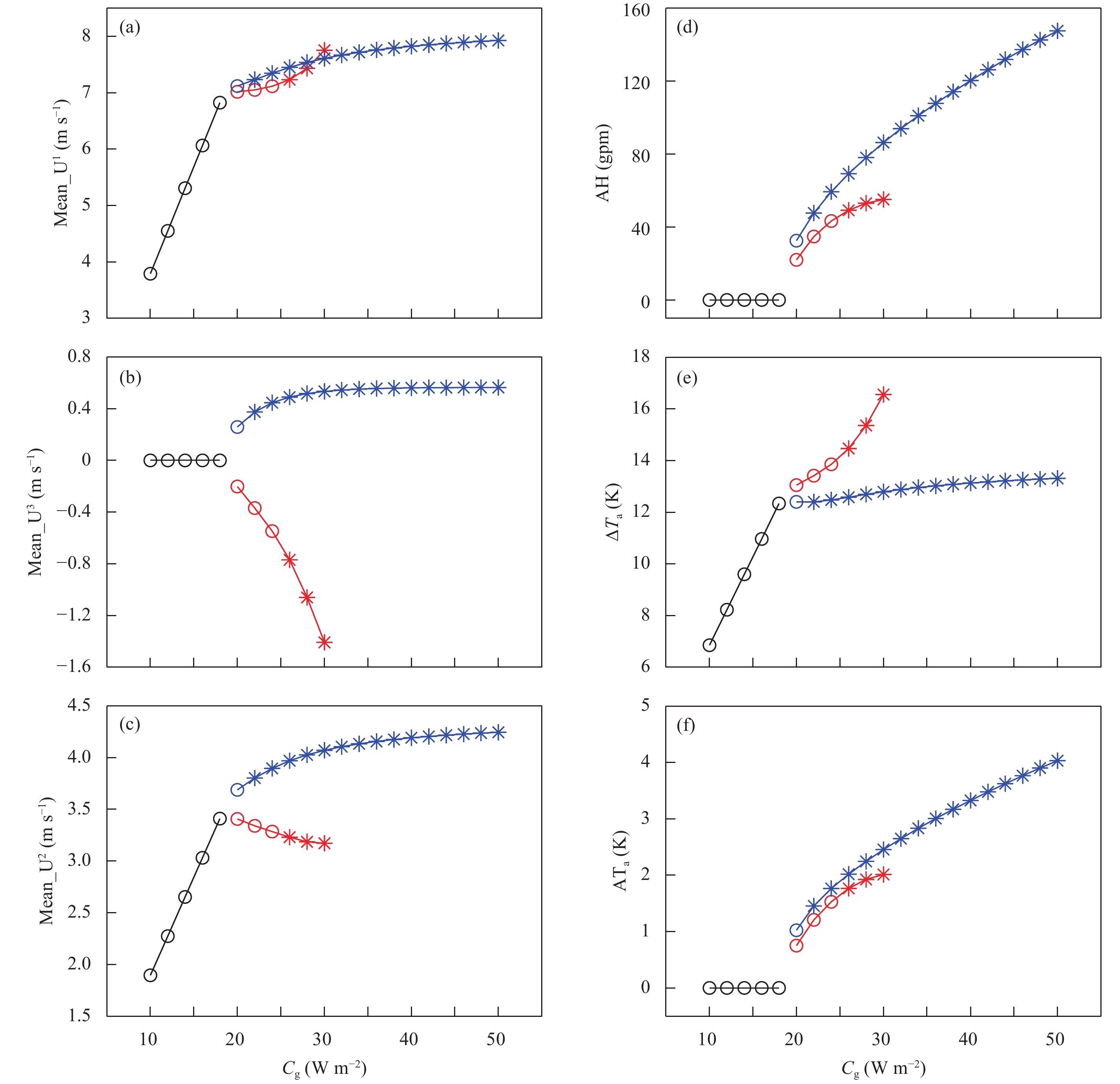
As expected, the wave amplitude AH of low-index equilibrium state is always greater than that of high-index equilibrium state at the same value of C_g (Figs. 11d, 12d). This phenomenon also occurs in wave amplitude of the atmospheric temperature field AT_a (Figs. 11f, 12f). However, the meridional atmospheric temperature gradient ΔT_a of low-index equilibrium state is always smaller than that of high-index equilibrium state at the same value of C_g (Figs. 11e, 12e).

These two types of equilibrium have no robust differences in the mean upper layer zonal wind speed: the Mean_U¹ of the low-index equilibrium state is smaller than that of the high-index equilibrium state at C_g = 54 W m^–2 for $m = 3.7$ (Fig. 11a). By contrast, the former is greater than the latter at C_g = 22, 24 W m^–2 for $m = 6$ (Fig. 12a). Besides, the differences in value of Mean_U¹ between high- and low-index equilibrium states are no more than 0.5 m s^–1. In addition, these two types of equilibrium also show no marked differences in nondimensional zonal component $\psi _1^1$ (see the overlap of the red circles and blue asterisks in Figs. 3a, d), which implies that the high- and low-index equilibria have no marked differences in upper layer zonal wind speed. Focusing on the middle-level zonal wind speed (Figs. 11c, 12c), the low-index equilibria always has a greater Mean_U² than the high-index equilibria at the same value of C_g; however, their differences are also no more than 1.0 m s^–1.

It is notable that our results regarding the differences between the high- and low-index equilibria in zonal wind differ from previous studies based on the barotropic models, in which the zonal component ${\psi _A}$ (i.e., zonal wind) of high-index equilibria was much greater than that of low-index equilibria (see Fig. 1 in CD; Figs. 13a, 14a in Huang et al., 2017a). One may argue that the upper layer zonal component $\psi _1^1$ of the magenta branch equilibrium states, which are characterized by small wave amplitude (Figs. 3e, f), are greater than that of the low-index equilibrium states of trough-type (Fig. 3d) in the baroclinic model, which can be an analogue of the results based on the barotropic model. However, the magenta branch equilibrium states are always unstable in the baroclinic model in this paper as well as in CS and RP. It should be noted that the results based on barotropic models disagree with the observations, e.g., some studies have shown that the probability density distribution of the zonal wind is unimodal (Benzi et al., 1986; Sutera, 1986). They are also inconsistent with the results of numerical experiments based on the general circulation model (Lindzen, 1986).

In fact, as emphasized in CS, the wavelike equilibrium is maintained not by the conversion of mean flow kinetic energy, but by the mean flow potential energy in the baroclinic atmosphere. Therefore, in our baroclinic model, as the low-index equilibria has larger wave amplitudes (Figs. 11d, 12d), there is indeed a reduction in meridional atmospheric temperature gradient of low-index equilibria (Figs. 11e, 12e) due to the consumption of mean flow potential energy. The low- and high-index equilibria certainly have no marked differences in zonal wind speed in our baroclinic model (Figs. 11a, 12a). However, in the barotropic model, the wavelike equilibria could only obtain energy from the mean flow kinetic energy. Therefore, in the barotropic model, the low-index equilibria with large wave amplitude undoubtedly has a lower zonal wind speed than the high-index equilibria with small wave amplitude.

The relationships between wave amplitude AH and meridional temperature gradient ΔT_a and ΔT_g are directly shown in Figs. 13a, b, e, f. As the wave amplitude of trough-type equilibria rapidly increases, the meridional atmospheric temperature gradient ΔT_a remarkably decreases for $m = 3.7$ (Fig. 13a, blue branch) and slowly increases for $m = 6$ (Fig. 13e, blue branch). This is because more and more potential energy is consumed to maintain the trough-type equilibria with rapidly increasing wave amplitude. The wave amplitude of ridge-type equilibria increases much slowly, so the meridional atmospheric temperature gradient ΔT_a increases rapidly for both $m = 3.7$ and $m = 6$ (Figs. 13a, e, red branch). Of course, the wavelike equilibria hardly draws energy from the land directly, so there is no marked reduction of meridional land temperature gradient ΔT_g for the equilibria with large wave amplitude (Figs. 13b, f).

In addition, regardless of ridge- or trough-type as well as high- or low-index equilibria, the wave amplitudes of both atmospheric and land temperature fields (AT_a and AT_g) are highly positively correlated with wave amplitude AH (Figs. 13c, d, g, h). In fact, the atmospheric and land temperature fields of equilibrium states are always nearly in phase with each upper layer streamfunction field (Figs. 1, 2). If the atmospheric temperature field showed a lag or lead to the streamfunction field in phase, the meridional perturbations of streamfunction field would continue to grow or decay due to the temperature advection. Thus, there would be no stationary waves, i.e., equilibrium states. As we have only obtained equilibrium solutions from Eqs. (19)–(27), the atmospheric temperature field is surely in phase with the streamfunction field. The formation of the zonal asymmetric structure of the land temperature field should be attributed to the interactions between the land and atmospheric temperature fields through radiative and heat exchange. Therefore, the changes in wave amplitude of both atmospheric and land temperature fields are highly consistent with that of the upper layer streamfunction field (Figs. 11d, f; 12d, f; 13c, d g, h). This result also suggests that the wavelike equilibrium is maintained by the conversion of the mean flow potential energy.

The results in this section show that the low-index (high-index) equilibrium states have a larger (smaller) wave amplitude and smaller (larger) meridional atmospheric temperature gradient; however, the two types equilibrium states have no marked differences in zonal wind speed. These results are attributed to the wavelike equilibrium that is maintained by the conversion of the mean flow potential energy in the baroclinic atmosphere.

7 Conclusions and discussion

To overcome the shortcoming of the classic Charney’s model that the thermal forcing is always artificially specified, we use a coupled land–atmosphere model. We find that there are still multiple equilibrium states in the presence of topography for a given realistic uneven solar heating. Therefore, this study again verifies the multiple flow equilibria theory. However, in addition to the multiple wave amplitude equilibria associated with high- and low-index types, multiple wave phase equilibria associated with ridge- and trough-types are more prominent in our coupled baroclinic model (Fig. 3). The multiple wave phase equilibria associated with ridge- and trough-types originate from the orographic instability of the Hadley circulation in the presence of topography, which is a pitch-fork bifurcation. Thus, the ridge- and trough-type equilibria can also coexist in the uncoupled model as long as the topography is present (Fig. 6c). But the multiple wave phase equilibria in the uncoupled model is unremarkable, mainly due to the very narrow orographically unstable region (Fig. 5b, Case 2). The land–atmosphere coupling considerably expands the orographically unstable region (Fig. 5b, Case 1), and thus, the multiple wave phase equilibria in the coupled model is prominent (Fig. 3). In other words, the land–atmosphere coupling generates more ridge-type equilibria in the coupled model (Fig. 3, red branch). We have demonstrated that the effect of the land–atmosphere coupling is primarily contributed by the longwave radiation fluxes, and the heat fluxes more or less modify the effect of longwave radiation fluxes. In the longwave radiation fields, the longwave radiation fluxes increase from low to high latitudes (Figs. 9b, f), which reduces the meridional gradient of the net diabatic heating. As a result, compared with the uncoupled model, the Hadley circulation in the coupled model is much more stable; besides, the atmospheric response to the thermal and topographic forcing is much weaker in the coupled model. In a word, the land–atmosphere coupling greatly stabilizes the atmospheric flow.

We have investigated the ridge- and trough-type equilibria and wave phase in details in this paper. The results show that the ridge-type (trough-type) equilibrium states have lower layer ridges (troughs) over the mountains and have lower layer easterlies (westerlies). We explain that the wave phase of equilibrium states relative to the topography depends on the direction of lower layer zonal wind and horizontal scale of the topography. However, why does the same solar forcing would yield two opposite directions of the lower layer zonal wind? In the absence of topography, we have demonstrated that there is no zonal flow in the lower layer for both the Hadley circulation [Eq. (29)] and the traveling wave (Figs. 4b, c). In the presence of topography, the first bifurcation of the Hadley circulation yields two branches of equilibrium states with opposite directions of the lower layer zonal wind (Figs. 11b, 12b): the trough-type equilibrium has lower layer westerlies, by contrary, the ridge-type equilibrium has lower layer easterlies. Therefore, the generation of two opposite directions of the lower layer zonal wind is still attributed to the presence of topography.

We have also investigated the high- and low-index equilibria and wave amplitude. The results show that the low-index (high-index) equilibrium states have a larger (smaller) wave amplitude and smaller (larger) meridional atmospheric temperature gradient. However, the high- and low-index equilibrium states have no marked differences in zonal wind speed in our coupled baroclinic model, and this result is qualitatively consistent with the observations (e.g., Benzi et al., 1986; Sutera, 1986). These results can be explained that the wavelike equilibrium is maintained by the conversion of the mean flow potential energy in the baroclinic atmosphere. Therefore, the previous conclusion that the high-index (low-index) equilibria has relative stronger (weaker) zonal flow in the barotropic model (e.g., CD) should be carefully reconsidered.

However, the low-order model that we used is oversimplified and has some limitations. For example, the vertical resolution of our two-layer model is still poor; The land–sea thermal contrast is not taken into account in our model; the flow patterns of the equilibrium states are sensitive to the horizontal resolution of the model (e.g., the flow patterns of the equilibrium states in 9-, 18-, and 24-component systems are different from each other (see Supplementary Figs. S1, S2), which implies that the eddy feedback is important). Therefore, the low-order model is only heuristic and this study is just preliminary. Nevertheless, our results on multiple wave phase equilibria are enlightening to the further study of some large-scale atmospheric phenomena, such as the recurrence of quasi-stationary planetary wave trough and planetary wave ridge over some regions, e.g., the Ural (Dole and Gordon, 1983; Li and Ji, 2001; Molteni, 2003; Ren et al., 2006; Pan et al., 2009; Tan et al., 2017; or see Supplementary Fig. S3). Further studies are needed that examine the extent to which our results agree with the observations. More realistic model should also be used to study the multiple wave phase equilibria in the future.

 

Acknowledgments. The authors appreciate Professor Ming Cai and also two anonymous reviewers whose detailed comments and constructive suggestions helped to improve the paper. We thank Professor Ruixin Huang of the Woods Hole Oceanographic Institution for his several helpful suggestions. We also thank Stéphane Vannitsem of the Institut Royal Météorologique de Belgique for providing the code of his low-order coupled atmos-phere–ocean model, which is helpful for us to design the low-order coupled land–atmosphere model.

Appendix

A. Linearization of the quartic terms in the radiative fluxes

We assume that

${T_{\rm{a}}}(x, y, t) = {T_{{\rm a}, 0}}(t) + \delta {T_{\rm{a}}}(x, y, t),$

(A1)

${T_{\rm{g}}}(x, y, t) = {T_{{\rm g}, 0}}(t) + \delta {T_{\rm{g}}}(x, y, t),$

(A2)

where ${T_{{\rm a}, 0}}$ and ${T_{{\rm g}, 0}}$ are spatially uniform averaged temperatures, $\delta {T_{\rm{a}}}$ and $\delta {T_{\rm{g}}}$ are temperature anomalies of the atmosphere and the land, respectively.

We assume that the shortwave solar radiation absorbed by the atmosphere and the land are just the function of latitude and time, i.e., ${R_{\rm{a}}}(y, t)$ and ${R_{\rm{g}}}(y, t)$ , and we set

${R_{\rm{a}}}(y, t) = {R_{{\rm a}, 0}}(t) + \delta {R_{\rm{a}}}(y, t),$

(A3)

${R_{\rm{g}}}(y, t) = {R_{{\rm g}, 0}}(t) + \delta {R_{\rm{g}}}(y, t),$

(A4)

where ${R_{{\rm a}, 0}}$ and ${R_{{\rm g}, 0}}$ are time dependent spatially uniform shortwave solar radiation, and $\delta {R_{\rm{a}}}$ and $\delta {R_{\rm{g}}}$ are spatially varying counterparts.

Neglecting the high-order terms in $\delta T$ and separating the averaged temperatures and perturbations, i.e., the zeroth-order terms in the expansion from the first-order ones, the atmospheric temperature equation [Eq. (6)] becomes

${\gamma _{\rm{a}}}\frac{{\partial {T_{{\rm a}, 0}}}}{{\partial t}} = - \lambda ({T_{{\rm a}, 0}} - {T_{{\rm g}, 0}}) + {\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{{\rm g}, 0}^4 - 2{\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{{\rm a}, 0}^4 + {R_{{\rm a}, 0}},$

(A5)

$\!\!\begin{split}& {\gamma _{\rm{a}}}(\frac{{\partial \delta {T_{\rm{a}}}}}{{\partial t}} + J(\psi, \delta {T_{\rm{a}}}) - \sigma \omega \frac{p}{R}) = - \lambda (\delta {T_{\rm{a}}} - \delta {T_{\rm{g}}}) \\ &\quad\quad + 4{\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{{\rm g}, 0}^3\delta {T_{\rm{g}}} - 8{\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{{\rm a}, 0}^3\delta {T_{\rm{a}}} + \delta {R_{\rm{a}}},\end{split}\qquad$

(A6)

and the land temperature equation [Eq. (7)] becomes

${\gamma _{\rm{g}}}\frac{{\partial {T_{{\rm g}, 0}}}}{{\partial t}} = - \lambda ({T_{{\rm g}, 0}} - {T_{{\rm a}, 0}}) - {\sigma _{\rm{B}}}T_{{\rm g}, 0}^4 + {\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{{\rm a}, 0}^4 + {R_{{\rm g}, 0}},$

(A7)

${\gamma _{\rm{g}}}\frac{{\partial \delta {T_{\rm{g}}}}}{{\partial t}} = - \lambda (\delta {T_{\rm{g}}} - \delta {T_{\rm{a}}}) - 4{\sigma _{\rm{B}}}T_{{\rm g}, 0}^3\delta {T_{\rm{g}}} + 4{\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{{\rm a}, 0}^3\delta {T_{\rm{a}}} + \delta {R_{\rm{g}}}.$

(A8)

Note that Eqs. (A5) and (A7) for the averaged temperatures are independent of the perturbations, and thus, stationary solutions can be obtained by solving

$ - \lambda ({T_{{\rm a}, 0}} - {T_{{\rm g}, 0}}) + {\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{{\rm g}, 0}^4 - 2{\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{{\rm a}, 0}^4 + {R_{{\rm a}, 0}} = 0,$

(A9)

$ - \lambda ({T_{{\rm g}, 0}} - {T_{{\rm a}, 0}}) - {\sigma _{\rm{B}}}T_{{\rm g}, 0}^4 + {\varepsilon _{\rm{a}}}{\sigma _{\rm{B}}}T_{{\rm a}, 0}^4 + {R_{{\rm g}, 0}} = 0.\quad$

(A10)

According to the parameter values listed in Table 1, particularly, $\lambda = 10$ W m^–2 K^–1, we get ${T_{{\rm a}, 0}} = 270.22$ K, ${T_{{\rm g}, 0}} = 280.40$ K, and they are the default values in the main body. For $\lambda = 0$ W m^–2 K^–1 in the experiment Case 3, we get ${T_{{\rm a}, 0}} = 264.16$ K, ${T_{{\rm g}, 0}} = 295.71$ K, and they are only used in Section 4 of the main body. Since stationary solutions are obtained, Eqs. (A5) and (A7) need not be considered any more, and we just focus on Eqs. (A6), (A8), (4), (5), and (8).

B. Equilibrium solutions and their stabilities

To obtain the general equilibrium solutions of Eqs. (19)–(27), we set all of the time derivatives to zero. We obtain

$- k({\psi _1} - {\theta _1}) - c\tilde h({\theta _3} - {\psi _3}) = 0, \qquad\qquad\qquad\quad\quad $

(A11)

$ - c{n^2}({\psi _1}{\psi _3} + {\theta _1}{\theta _3}) + \beta n{\psi _3} - {B_1}({\psi _2} - {\theta _2}) = 0,\qquad$

(A12)

$c[{n^2}({\psi _1}{\psi _2} + {\theta _1}{\theta _2}) + \tilde h({\theta _1} - {\psi _1})] - \beta n{\psi _2} - {B_1}({\psi _3} - {\theta _3}) = 0, $

(A13)

$c[{\psi _2}{\theta _3} - {\psi _3}{\theta _2} - \sigma '\tilde h({\psi _3} - {\theta _3})] - {B_3}{\theta _1} + k\sigma '{\psi _1} + {D_1}{\theta _1} + {D_2} = 0,$

(A14)

$c({A_1}{\psi _3}{\theta _1} - {A_2}{\psi _1}{\theta _3}) + \beta n\sigma '{\theta _3} - {B_2}{\theta _2} + {B_1}\sigma '{\psi _2} + {D_1}{\theta _2} = 0,\quad$

(A15)

$\begin{split} & c[{A_2}{\psi _1}{\theta _2} - {A_1}{\psi _2}{\theta _1} + \sigma '\tilde h({\psi _1} - {\theta _1})] - \beta n\sigma '{\theta _2}\\ &\quad\quad - {B_2}{\theta _3} + {B_1}\sigma '{\psi _3} + {D_1}{\theta _3} = 0,\end{split}\qquad\qquad\qquad\quad$

(A16)

and

$\left. \begin{aligned}& {T_{{\rm g}, 1}} = \frac{{{d_4}}}{{{d_3}}}{\theta _1} + \frac{{{{C'}_{\rm{\!\!\!\!g}}}}}{{{d_3}}} \\& {T_{{\rm g}, 2}} = \frac{{{d_4}}}{{{d_3}}}{\theta _2} \\& {T_{{\rm g}, 3}} = \frac{{{d_4}}}{{{d_3}}}{\theta _3}\end{aligned} \right\}.$

(A17)

In view of Eq. (A11), Eq. (A14) may be written as

$c[{\psi _2}{\theta _3} - {\psi _3}{\theta _2}] + ({D_1} - 2k'\sigma '){\theta _1} + {D_2} = 0,$

(A18)

and Eqs. (A12), (A13), (A15), and (A16) become

$\left[ {\begin{array}{*{20}{c}} { - {B_1}}&{{B_1}}&{ - c{n^2}{\psi _1} + \beta n}&{ - c{n^2}{\theta _1}} \\ {c{n^2}{\psi _1} - \beta n}&{c{n^2}{\theta _1}}&{ - {B_1}}&{{B_1}} \\ {{B_1}\sigma '}&{{D_1} - {B_2}}&{c{A_1}{\theta _1}}&{ - c{A_2}{\psi _1} + \beta n\sigma '} \\ { - c{A_1}{\theta _1}}&{c{A_2}{\psi _1} - \beta n\sigma '}&{{B_1}\sigma '}&{{D_1} - {B_2}} \end{array}} \right]\left[ {\begin{array}{*{20}{c}} {{\psi _2}} \\[7.5pt] {{\theta _2}} \\[7.5pt] {{\psi _3}} \\[7.5pt] {{\theta _3}} \end{array}} \right] = \left[ {\begin{array}{*{20}{c}} 0 \\ {c\tilde h({\psi _1} - {\theta _1})} \\ 0 \\ { - c\sigma '\tilde h({\psi _1} - {\theta _1})} \end{array}} \right].$

(A19)

Equation (A19) constitutes a linear system for the variables $({\psi _2},\;{\theta _2}, \;{\psi _3}, \;{\theta _3})$ if the zonal variables $({\psi _1}, \;{\theta _1})$ are specified. The general solution of Eq. (A19) is described in detail in Supplementary Section 1. If we set $\tilde h = 0$ , then the right side of Eq. (A19) becomes a zero matrix. In general, the value of the coefficient determinant on the left side is not equal to zero. Thus, in this case, the solutions of wave components $({\psi _2}, \;{\theta _2}, \;{\psi _3}, \;{\theta _3})$ are all equal to zero. Therefore, the wavelike equilibria (wave components are not zero, i.e., stationary wave solution) cannot exist without the topography in this system.

The stability of the equilibrium solution obtained from Eqs. (19)–(27) is determined from the characteristic values of the linear perturbation equations coefficient matrix, which is a nine homogeneous linear equations governing $({\psi _1}, \;{\psi _2}, \;{\psi _3}, \;{\theta _1}, \;{\theta _2}, \;{\theta _3}, \;{T_{g, 1}}, \;{T_{g, 2}}, \;{T_{g, 3}})$ (see Supplementary Section 1). Set all perturbation quantities be proportional to ${e^{ + \alpha t}}$ , we obtain a nine-order equation in the variable $\alpha $ . If the maximum real part of $\alpha $ is greater than zero, the equilibrium is unstable, otherwise it is stable.