木材压缩技术是将木材进行软化处理后压缩变形，以减少木材细胞的空腔体积，增加木材的密度，并对压缩变形进行永久固定的木材改性处理技术(Kollmann et al., 1975；Norimoto, 1993)。木材经压缩后，不仅解剖构造发生了很大变化，而且物理、力学性质也完全不同于素材，密度、硬度和耐磨性显著提高，强度和模量增大，性能得到显著改善(Tsunematsu et al., 2006；Yoshihara et al., 2008; Kitamori et al., 2010；李坚等，2011)。

木材压缩技术最早出现于20世纪30年代的美国和德国(Walsh et al., 1923；Olesheimer, 1929)，最初的压缩木在军用飞机上使用，用来替代金属材料，目的是防止被雷达发现。直到20世纪90年代，以改善软质木材性能、拓宽人工林木材应用范围为目标的木材压缩技术研究才受到世界各国学者和产业界的重视。经过20多年的发展，木材压缩技术，特别是湿热软化的木材压缩技术在木材软化、塑性变形、压缩木材的性能变化、压缩变形固定及其机制以及压缩方式和压缩工艺等方面逐步得到完善(Norimoto, 1993；Liu et al., 1993；Inoue et al., 1993；1998；2000；2008；Udaka et al., 1998；2000；2003；Matsumoto et al., 2012)，并形成了湿热软化处理后的原木整形压缩、锯材整体压缩、单板压缩、锯材表层压缩以及高频加热软化和变形固定等压缩木加工技术体系，而且已经在木材工业界推广应用(足立幸司等，2006；李坚等，2011)。

本文针对湿热软化下的木材压缩问题，从木材软化机制、软化特性、软化点的确定、热板加热下的传热传质特性、层状压缩的形成和压缩变形固定等方面分析木材压缩技术的研究现状、进展以及存在的问题。

木材之所以能够被压缩，主要取决于2方面原因：一是构成木材的各类细胞都是具有孔隙的空腔结构，存在压缩的空间；二是构成木材多孔结构骨架的细胞壁成分具有可塑性，在遇到水、氨、低分子醇、酚等极性无机或有机型气体或液体时会发生膨胀，使木材的弹性模量降低，软化温度下降，成为塑性材料(Morisato et al., 1999；李坚，2009)。虽然干燥木材是一种缺乏塑性的材料，在常温下压缩很容易被破坏，但在高温高含水率下，由于木材细胞壁被软化而成为塑性材料，从垂直于纤维方向压缩木材(横纹压缩)，可以发生细胞腔完全消失的大变形(Norimoto, 1993；Liu et al., 1993)，即使从平行于纤维方向压缩木材(顺纹压缩)，在很大的压缩变形下木材也不会被破坏。可见，木材软化是木材塑性压缩变形的必要条件。

木材是一种具有弹塑性的天然高聚物，其主要成分纤维素、半纤维素和木质素的特性及所占比例直接影响木材的可塑性(Takamura, 1968；Furuta et al., 2010；Yokoyama et al., 2000)。早在20世纪60年代，Goring(1963)就对木材三大组分在干、湿状态下的软化特性进行了研究，气干状态下，纤维素、半纤维素和木质素的玻璃化转变温度分别在231~253 ℃、167~217 ℃和134~235 ℃之间；湿润状态下，半纤维素和木质素的玻璃化转变温度分别降低到54~142 ℃和77~128 ℃之间，但纤维素对水分不敏感，其玻璃化转变温度几乎不发生变化。这一研究结果作为木材软化的重要依据，被多部论著引用(城代進等，1996；李坚，2009；李坚等，2011；刘一星等，2012)。

Takamura等(1968)进一步详细分析了含水率对木材三大组分的影响，结果发现，在240 ℃以下时，纤维素的软化温度几乎不受含水率影响，而半纤维素和木质素的软化温度随着含水率的增加显著降低。在绝干状态下，半纤维素和木质素的软化温度分别为200 ℃左右和150 ℃左右，在含水率约60%时，半纤维素的软化温度降低到20 ℃，而木质素在含水率约20%时，软化温度降低到80 ℃左右，之后随着含水率增加软化温度几乎不会降低。可见，水分和热量都能对木材组分起到增塑作用，特别是在湿热共同作用下增塑作用更加显著。

从木材组分的分子结构看，纤维素的非结晶区、半纤维素和木质素对水分都有很强的亲和性，对木材的吸湿膨胀起很大作用，其中木质素的软化点与是否存在水分关系密切，而且木质素的含量和软化特性是影响木材软化的主要因素(Furuta et al., 2010；Yokoyama et al., 2000)。纤维素的软化温度在240 ℃左右，远远高于木材压缩软化的温度范围，而且其玻璃化转变温度不受含水率影响，因此对木材软化的影响很小(Higashihara et al., 2003)。

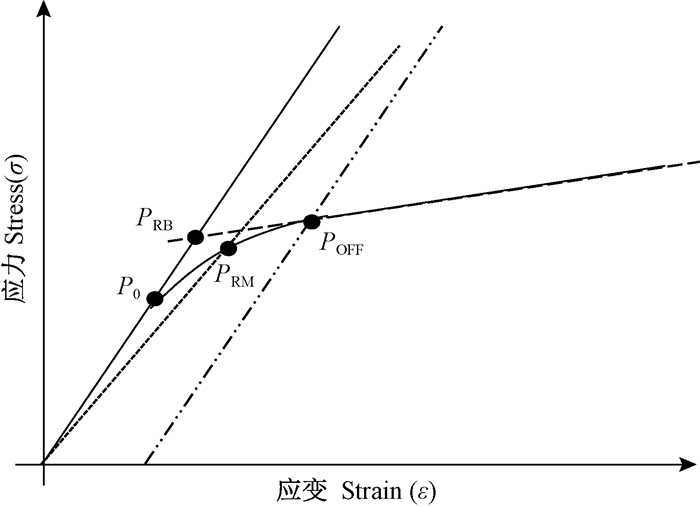
软化点是评价木材软化的重要指标，玻璃化转变温度和应力屈服点是表征木材软化最常用的参数，也可以用弹性模量随温度升高的急剧变化点表征木材的软化点(李坚，2009；2014)。在木材弹塑性分析中，应力屈服点控制了材料在塑性区域的应力-应变关系，同时也决定了塑性变形潜能(Yoshihara et al., 1994)。但由于木材成分和结构非常复杂，应力-应变关系的拐点并不明显(图 1)，在应力-应变曲线中很难确定屈服应力，也无法得到准确的应力屈服点；而且木材的应力-应变关系因树种、木材组织构造、压缩方向、木材含水率和温度的不同而差异非常显著(Tabarsa et al., 2000；2001；Chui et al., 2007；Liu et al., 1993)。因此，应力屈服点和屈服应力的确定是木材塑性变形表征的关键点，也是一个难点。目前，木材压缩应力-应变关系的研究主要以干燥木材和饱水木材为对象，分析水分对应力-应变关系和屈服应力的影响(Norimoto, 1993；Liu et al., 1993；Yoshihara et al., 1994；Chui et al., 2007)。

图 1 应力-应变曲线 Figure 1 Cure of stress-strain P0、PRB、POFF和PRM分别是“非补偿法”、“Reily-Burstein法”、“应变补偿法”和“缩减弹性模量法”得到的屈服点。 P0, PRB, POFF and PRM were the yield points, measuring by the "none-offset method", "Reily-Burstein method", "strain offset method" and "reduced modulus method".

高分子聚合材料应力屈服点的确定方法有4种(Yoshihara et al., 1997)。第1种方法是以应力-应变曲线中弹性阶段和塑性阶段的交叉点作为屈服点(图 1中P₀)，这种方法又称“非补偿法”。第2种方法是以应力-应变曲线中屈服前后弹性阶段2条直线延长线的交点为屈服点(图 1中P_RB)，也称“Reily-Burstein法”(Reily et al., 1975)。这2种方法的缺点是屈服点的确定取决于试验者的观察，不同试验者得到的结果有偏差。第3种方法是以应力-应变曲线和应变补偿直线的交点为屈服点(图 1中P_OFF)，又称“应变补偿法”(Raghava et al., 1973)，其补偿值一般为0.2%~3%。第4种方法是以应力-应变曲线和缩减一定弹性模量后应力-应变直线的交点为屈服点(图 1中P_RM)，也称“缩减弹性模量法”，一般弹性模量缩减值为3%~5%，该种方法也是日本工业标准规定的方法。这4种方法得到的屈服点的屈服应力有很大差异，其中“非补偿”与其他3种方法得到的屈服点的屈服应力相差1.2~2.5倍之多(Yoshihara et al., 1997)。

水分和热量是影响木材软化和压缩变形的2个重要因素，因此热板加热下木材的传热传质是木材压缩变形过程中必须考虑的问题，特别是在研究木材层状控制压缩时尤为重要。

木材是一种天然有机多孔材料，无论是其固相骨架细胞壁还是细胞腔、细胞间的间隙等孔隙结构以及水分存在形式都比较复杂，因而影响木材传热传质规律因素很多，在建立模型时涉及参数也多，如木材密度、导热系数、水分扩散系数、比热容、加热温度、初始含水率、纤维饱和点等(俞昌铭，2011)。木材的传热传质一直是木材干燥研究的核心问题，其中，水分扩散规律是干燥过程数字模拟的理论基础，因此关于干燥过程中水分扩散规律以及水分扩散的非稳态模型方面的研究报道很多(Schmidt, 1967；Bramhall, 1979；李延军等，2007；李梁等，2009)。热板加热下的传热传质问题最早也是作为木材干燥研究中的一个科学问题被提出的(Crank, 1956)。单板干燥主要考虑湿热的水平分布问题，多用二维非稳态模型分析干燥过程中的传热传质规律。在锯材干燥研究过程中，由于上下热板的加热条件对称而均匀地施于木材上下表面，使平板内水平方向的热传递很微弱，可以忽略不计，只需计算厚度方向的热传递和气体的渗流，因此传热传质过程可以简化为一维非稳态传热传质问题，建立数学模型，分析传热传质规律(俞昌铭，2011)。

对既有自由水又有吸着水的木材加热后，作为含水率分界线的纤维饱和点是一个临界值，以此为界限构成的干湿界面是木材传热传质模型的重要参数。Tang等(1994)假设加热界面上的温度保持水的沸点温度(约100 ℃)，并在满足一维非稳态的假设和边界条件下建立了热板加热下的干湿界面退却模型以及一维非稳态传热模型和传质模型(图 2)，分析了热量和水分的传递规律以及干湿界面的退却规律。汪佑宏等(2005；2008)参考该模型的假设条件和边界条件，分别建立了马尾松(Pinus massoniana)锯材在热压干燥过程中的传热传质模型，对理论值和实测值偏差的分析结果表明，二者之间的温度偏差小于5%，含水率偏差在干区小于10%，但湿区的含水率偏差较大。

图 2 热压过程中热量传递和界面退却模型 Figure 2 Model for heat transfer and retreat of interface in the press-drying process 根据Tang等(1994)图绘制。Modified following an original drawing by Tang et al. (1994).

整体压缩是将木材软化后在一定压缩率下对木材进行压缩密实，软化过程一般只强调达到软化温度并保持一定的时间，因此，多数研究都是在饱水或饱和水蒸气软化条件下展开的(Norimoto, 1993；Inoue et al., 1993；1998；2000；Udaka et al., 1998；2000；李坚，2014)。

整体压缩能够显著提高软质木材的物理力学性能，而且压缩处理后的木材密度更均匀。但由于软质木材密度非常低，只有通过提高压缩率才能得到密度高、硬度大的压缩木，因此这种压缩方式会带来很大的木材材积损失，提高了压缩木的制造成本。为了减少压缩带来的木材材积损失，很多学者开展了表层压缩研究。有采用200 ℃以上的高温热板加热软化进行表层压缩的研究报道(涂登云等，2012)，但这种方式形成的压缩层厚度非常有限，而且密度梯度大，刨光加工后压缩层有可能消失，实际上这种压缩方式更接近于整体压缩。通过表层浸渍酚醛树脂、乙二醛树脂和三聚氰胺树脂等化学聚合物的方式进行木材表层压缩的研究报道很多(Inoue et al., 1990；1991；Tokuda et al., 2003；Adachi et al., 2005；刘君良等，2002)。表层树脂浸渍虽然能获得表面性能显著改善的表层压缩木材，但树脂浸渍深度的控制比较复杂，通过湿热处理不能解决树脂浸渍下的表层压缩厚度控制问题，而且采用化学聚合物浸渍处理的压缩木存在害物质释放问题，不容易被使用者接受。

层状压缩是将木材的表层或者中间层进行压缩密实，形成压缩层和未压缩层同时存在的压缩木(Huang et al., 2012)。这种压缩方式要在木材内部不同层面上形成屈服应力差，因此需要进行分层软化处理。

水热控制下的层状压缩是一种新型压缩方式(Huang et al., 2012)，是利用木材多孔性和各向异性的特点通过工艺过程设计实现的。由于木材3个方向上的细胞及组织的排列方式不同，因此木材3个断面的水分渗透性差异极其显著。木材轴向水分的渗透速度是弦向的5 000~5万倍，是径向的500~8 000倍(高橋徹等，1995)。利用木材3个断面水分渗透性的显著差异，对木材横截面进行封闭处理，阻断水分从横截面进入木材内部，通过浸水、放置、热板加热等处理，可使木材内部各个层面上形成含水率差异显著的梯度分布(李贤军等，2010)，压缩后能够获得如图 3所示的层状压缩木材，压缩层的密度可达0.8 g·cm^-3以上，未压缩层仍然保持原有的密度，而且压缩层的形成部位是可控的(Huang et al., 2012；王艳伟等，2012；夏捷等，2013；Gao et al., 2016)。这种压缩方式不仅克服了单纯高温热软化表层压缩存在的压缩层厚度小、压缩断面密度呈线性梯度分布(涂登云等，2012)的缺点，而且由于压缩层形成的位置和厚度具有可控性，能够依据结构材料要求，通过木材内部湿热分布的调控形成“内强外弱”的效果，增加材料刚度。层状压缩木材的这些特性在一定程度上可以解决整体压缩木材损失大的问题，处理过程中不使用任何化学药剂，具有环保性。

图 3 不同预热时间下形成的压缩木材横截面照片及压缩木材的密度分布 Figure 3 The photograph and density profiles of transverses sections of compressed wood at different pre-heating times 10 s, 40 s, 240 s and 420 s表示预热时间，箭头表示压缩方向。10 s, 40 s, 240 s and 420 s, represent the wood which was compressed after pre-heating for 10 s, 40 s, 240 s and 420 s, respectively, and arrow indicates the direction of compression.

但目前湿热软化、压缩研究多数都是基于木材整体实施的整体压缩，木材软化和屈服应力的湿热响应规律以及木材湿热梯度分布形成和调控方面的研究报道很少。但要实现层状压缩的可控性，还需要在木材软化、屈服应力、热板加热下的传热传质规律等方面开展深入研究。

木材的弹性性质决定了木材软化后压缩可以形成很大的塑性变形，但如果在压缩应力没有释放的情况下解除载荷，压缩变形会基本全部恢复(Norimoto, 1993；李坚等，2011；Zhao et al., 2015)。因此，如何使压缩后的变形不回弹、实现压缩变形的永久固定是木材压缩技术必须解决的另一个关键科学问题。

木材细胞壁具有复杂的层状构造，主要由纤维素、半纤维素和木质素组成，各层的微纤丝群间充填了以木质素为主体的基质。微纤丝和基质在湿热软化压缩变形状态下干燥后，微纤丝表面的纤维素和半纤维素分子吸着的水分子脱水形成氢键，温度降低后可以使压缩变形固定。采用压缩-卸载-再压缩的多次循环或长时间高温保压的处理方式，可使压缩应力部分释放并形成变定，将压缩变形固定，但这种以变定方式实现的压缩变形固定在湿热条件下依然会因吸湿而全部回弹(Norimoto, 1993；Inoue et al., 1993；Matsumoto et al., 2012)。

在压缩状态下进行高温热处理或高温水蒸气处理，可使木材成分水解、微纤丝和基质成分的分子链断裂以及再聚合，使木材内部应力释放，降低变形恢复力，使压缩变形永久固定(Norimoto, 1993)。虽然高温热处理能够有效降低压缩变形恢复率，但要实现压缩变形的永久固定，还需进行高压水蒸气处理，180 ℃以上的饱和水蒸气处理能使压缩变形永久固定(Inoue et al., 1993；2000；Dwianto et al., 1998；2000；Higashihara et al., 2000；2003；2004)。基于高压水蒸气对压缩变形固定的效果，采用密闭条件下的加热处理和高频加热处理等能产生高压水蒸气环境的方式进行压缩变形固定，效果也非常显著(Udaka et al., 2000；2005；Inoue et al., 1998)。

综上所述，20多年来，世界各国学者围绕木材湿热软化压缩问题，从木材软化、压缩变形固定等处理方法到形成机制开展了深入广泛的研究，而且已经取得了大量有价值的研究成果。在木材整体压缩情况下，形成了原木整形压缩、锯材整体压缩、单板压缩等比较完整的技术体系，利用高频加热技术开发了加热软化和变形固定的一体化技术，并且实现了工业化应用。但由于整体压缩方式会带来很大的木材材积损失，压缩木制造成本高，限制了压缩木的市场化应用。

水热控制下的层状压缩技术可以解决整体压缩材积木材损失大的问题，但目前木材压缩变形机制的研究都是围绕木材的整体压缩开展的，缺乏木材软化点和屈服应力随含水率变化规律以及热板加热下木材内部屈服应力差变化规律的基础研究，因此，要实现层状压缩的可控性，还需要在热板加热下的传热传质规律及木材湿热梯度分布的形成与调控等方面开展深入研究。