Numerical Analysis of Stress Concentration in Non-uniformly Corroded Small-Scale Specimens
https://doi.org/10.1007/s11804-020-00154-2
-
Abstract
A numerical evaluation of stress concentrations of corroded plate surfaces of small-scale corroded steel specimens is compared with the experimentally estimated ones. Eleven specimens were cut from a steel box girder, which was initially corroded in real seawater conditions. The surface of all corroded specimens was analysed applying photogrammetry techniques, and a statistical description of an idealised corroded surface of each specimen was established. Fatigue lives of specimens are determined from the fatigue tests. Based on experimentally obtained fatigue lives, the stress concentration factors are calculated concerning the ideally smooth specimens. The correlation between the statistical parameters of the corroded specimen surfaces and the estimated stress concentration factors is analysed. Idealised corroded surfaces, converted in graphical format, are then used for the finite element modelling in ABAQUS software, and stress concentration factors are estimated from the finite element results. A convergence study is performed to determine the appropriate finite element mesh density. Comparison between experimentally obtained and numerically estimated stress concentration factors is performed as well as correlation analysis between actual and finite element predicted crack locations.-
Keywords:
- Fatigue life ·
- Fatigue tests ·
- Corrosion ·
- Stress concentration factors ·
- Finite element method
Article Highlights• Fatigue lives of specimens are defined based on fatigue tests.• Photogrammetry techniques are used to analyse the corroded surface of specimens.• Comparison between experimentally and numerically estimated stress concentration factors is performed as well as correlation analysis is performed.• A numerical evaluation of stress concentrations of corroded steel specimen surfaces is analysed and compared with the experimentally estimated ones. -
1 Introduction
Corrosion and fatigue cracking may be the two most important types of damage in ageing ship structures, which lead to surface roughness, reduction of the plate thickness and strength and eventually to leakage and structural failure.
Corrosion in ship structures has an essential role in long-term structural integrity. Under conditions of high temperature, improper ventilation, high stress concentration, high stress cycling, very high rates of corrosion can be achieved in spaces such as ballast tanks and at specific structural details such as horizontal stringers or connections of longitudinal stiffeners and web frames. This has been a source of concern by ship operators, and Classification Societies that have collected much service data (TSCF 1992, 1997; Wang et al. 2003; Yamamoto and Ikagaki 1998). Corrosion has clear consequences in degrading the ultimate strength of ship structures (Jiang and Guedes Soares 2012; Nakai et al. 2006; Paik et al. 2003; Silva et al. 2013) and also in affecting the fatigue strength by increasing the stress levels (Garbatov et al. 2002; Guedes Soares and Garbatov 1998; Moan and Ayala-Uraga 2008; Tran Nguyen et al. 2012, 2013) and also by a direct degradation of fatigue strength (Garbatov 2016; Garbatov et al. 2014a).
Fatigue is an important design criterion for locally welded structural components and global structures. The fatigue damage may even further reduce the strength because of the presence of different imperfections, which can lead to a local increase of stresses and fatigue crack initiation and propagation. For fatigue life assessments, different procedures have been developed based on databases of the fatigue behaviour of welded structural components as a result of both tests and theoretical investigations (Fricke and Petershagen 1992).
The main steps in fatigue analysis are based on direct calculations that involve the description of the wave-induced loading (Guedes Soares and Moan 1991) the stress distribution in the structure (Guedes Soares et al. 2003), the model of fatigue damage (S-N approach) or fracture mechanics approach (Paris and Erdogan 1963) and the probabilistic evaluation of the different steps to arrive at a safety index or time-dependent reliability as has been developed in Guedes Soares and Garbatov (1999). The analysis of stresses is a difficult task due to the complexity of the ship structures. Nowadays, the methods that are mostly accepted and spread for analysing complex welded structures are the hot spot stress approach (Petershagen et al. 1991) and the effective notch stress approach (Radaj 1990; Radaj et al. 2009). Recommendations for fatigue stress assessment can be found in guidelines (Niemi 1992, 1995; Niemi et al. 2004).
The work presented here is analysing small-scale specimens of a transversely stiffened welded plate, which were firstly corroded and then fatigue tested. The surfaces of 11 corroded specimens are analysed applying photogrammetry techniques to obtain the 3D geometrical description of the corroded surfaces. The description of fatigue tests, together with results and statistics of the corroded surfaces, is given in Garbatov et al. (2014a) and Garbatov et al. (2014b).
In the present study, the relations between the statistical properties of the corroded surfaces of the specimens and the experimentally estimated stress concentration factors (SCFs) are investigated. Finite element (FE) analyses, using very fine 3D elements, have been performed on the idealised finite element models of corroded specimens to estimate the SCFs. To determine an appropriate mesh density, the convergence study is performed. SCFs calculated by the finite element method (FEM) are then compared with the ones estimated experimentally, and their dependency on statistical properties of corroded surfaces is investigated. The correlation between actual and predicted crack locations is also calculated and discussed.
2 Fatigue Test Setup
The fatigue specimens have been cut from box girders that have been subjected to corrosion, in a real corrosive environment in direct contact with seawater. The dimensions of the box girder specimen were 1400 × 800 × 600 mm. The box girder was made of standard shipbuilding steel with a yield stress of 235 MPa. The box girder specimen was exposed to the Baltic seawater and tested in hot water. The box girder was placed in a large tank, and seawater was pumped into the tank continuously. The temperature of seawater was increased and additionally, oxygen depolarisation sub process rate was increased by the agitation of seawater, which resulted in a corrosion rate increase.
The test duration was 90 days without polarisation. More detailed information about the corrosion set up may be seen in Domzalicki et al. (2009). The total weight loss observed was 56 kg (23% of initial weight). The average value of the electrolyte flow rate was 308 dm3/h; water temperature was 45.2 ℃ and pH = 7. 93.
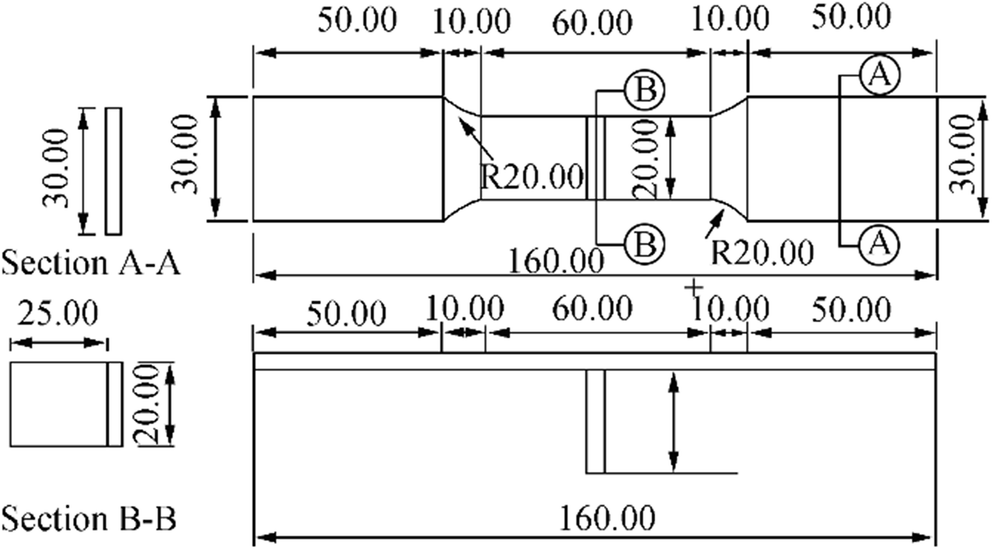
After the test was completed, the box girder was covered with iron corrosion products. This box girder has been subjected to ultimate strength tests as described in Saad-Eldeen et al. (2011, 2013a, b). Eleven fatigue test specimens were cut from the box girder in a shape that can be seen in Figure 1 (Garbatov et al. 2014a).
The non-corroded thickness of specimens was 4.5 mm. To accurately characterise the test specimens, an analysis of their thickness has been made employing photogrammetry techniques. The corroded specimens were scanned with an optical 3D scanner (Atos III Triple Scan, GOM mbH, Germany). The geometries were reconstructed using Geomagic Spark (3D Systems, USA) software, and the thickness of the specimens was determined using GOM Inspect (GOM mbH, Germany) software packages (see Figure 2). The minimum, maximum, mean value and standard deviation of corroded specimen thicknesses are given in Table 1 (Garbatov et al. 2014a).
Table 1 Corroded specimen thickness descriptors (Garbatov et al. 2014a)Thickness (mm) Max Min Mean value St. Dev. A6 3.08 1.5 2.44 0.39 A7 3.07 1.52 2.39 0.28 A8 3.23 1.62 2.53 0.32 B9 3.22 2.13 2.72 0.19 A10 3.07 1.48 2.4 0.36 B14 3.23 2.1 2.69 0.11 B17 3.14 2.09 2.6 0.17 C4 3.28 2.04 2.53 0.19 C11 3.29 1.83 2.57 0.23 C12 3.09 1.96 2.61 0.22 C13 2.98 2.02 2.52 0.16 The corroded specimens (see Figure 1) were fatigue tested to axial cyclic load (Garbatov et al. 2014a). A transverse stiffener is welded to one side of the specimen. The specimens are not symmetrical about the load axis. The design of the specimen assumes that if the specimen is intact, without corrosion, the failure occurs along the toe of the transverse fillet weld.
Eleven specimens with different corrosion degradation levels are used to analyse the effects of the degradation and to evaluate the fatigue performance. Figure 1 shows the dimensions of specimens. All specimens were 180 mm in length and had a plate thickness of intact non-corroded specimen of 4.5 mm. The stiffener of intact non-corroded specimen was also 4.5 mm thick. The stiffener width was 17.6 mm. The specimens were welded based on regular shipyard practice and were machined to smooth the edges.
All specimens were tested under cyclic loading at a nominal stress range, Δσ as shown in Table 2. The stress range is the difference between the minimum and maximum nominal stresses. The stress range across the net section of the plate, which varies from specimen to specimen as can be seen in Table 2, is modelled as 120, 155 and 200 MPa, respectively. These nominal stresses were calculated by dividing the applied axial load on the specimen by the mean cross-sectional area of the plate. The lower stress limits correspond to 6, 8 and 11 MPa, respectively, whereas the upper-stress limit was 126, 163 and 210 MPa, respectively. It should be noted that the three groups of loading have been simulated A, B and C. The first group consists of specimens 6, 7, 8 and 10, the second group covers the specimens 9, 14 and 17 and the third one includes 4, 11, 12 and 13 (Figure 3).
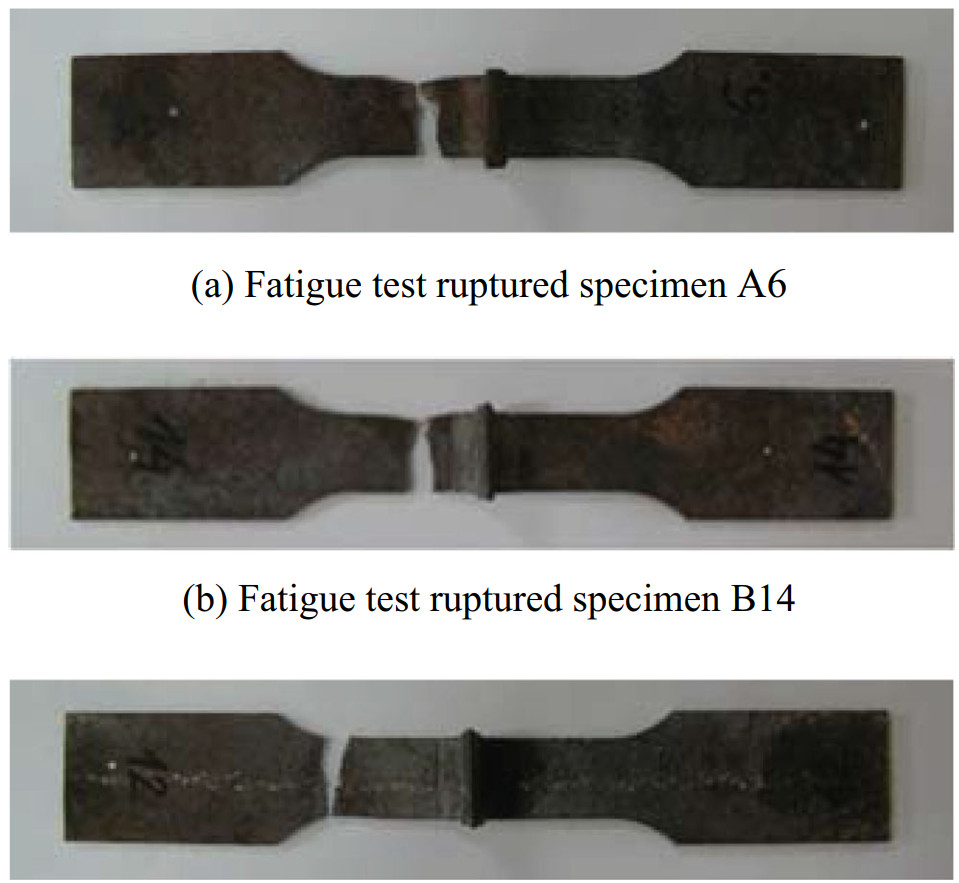
Table 2 Principal characteristics of corroded specimens (Garbatov et al. 2014a)Load group Specimen R Δσ, MPa Frequency (Hz) Number of cycles A 6 0.05 120 10 552 360 7 0.05 120 10 169 023 8 0.05 120 10 915 974 10 0.05 120 10 212 719 B 9 0.05 155 10 368 200 14 0.05 155 10 842 368 17 0.05 155 10 315 611 C 4 0.05 200 10 41 143 11 0.05 200 10 69 252 12 0.05 200 10 98 715 13 0.05 200 10 119 801 All samples were tested to failure, and typical crack locations are shown in Figure 3. It has been observed that all of the failures initiated at a specific corrosion pit at the narrowed plate away from the fillet weld due to severe corrosion in the specimens. None of the specimens failed at the weld toe, although this was expected before performing experiments.
3 Fatigue Strength and SCF Analysis
The fatigue life, or several loading cycles to failure, was recorded and presented for each specimen in Table 2. A very large scatter of fatigue life can be observed. Fatigue life data exhibit widely scattered results because of inherent microstructural inhomogeneity in the material properties, differences in the surface and material properties due to corrosion.
3.1 Experimentally Estimated SCFs
To estimate the SCF experimentally, the S-N curve is defined as
(Δσ)mN=K50 (1) where Δσ is the stress range, N the number of cycles to failure while m and K50 are the constants depending on the type of welded connection. The index "50" denotes the mean S-N curve, i.e. for the stress range level Δσ, the specimen fails with a probability of 50%. The stress range, for a given number of cycles to failure N, can be calculated using Eq. (1) as
Δσ=(K50N)1m. (2) The S-N curve used in the present study for the assessment of the specimens, excluding any stress concentration, is the category "B" curve as defined by the UK Department of Energy (BV 1996). The curve is valid for non-tubular joints in as-rolled condition with no flame-cut edges, and the constants m = 3 and K50 = 1.342 × 1013 characterises it. The stress range calculated by Eq. (2) is to be multiplied by factor 1.45 representing the ratio of fatigue strength of a smooth specimen to that of the plates in the as-rolled condition (BV 1996):
ΔσSS=1.45ΔσAR (3) where ΔσAR represents a stress range of as-rolled specimen given by Eq. (2). The factor 1.45 is taken as stipulated in BV (1996) as a ratio of the fatigue strength of a smooth specimen to as-rolled one. The B-class refers to as-built specimen, while the strength of the smooth specimen is 1.45 times higher.
The SCF of each corroded specimen may then be experimentally defined as a ratio of stress range, ΔσSS calculated by Eq. (3), for the number of cycles of the fatigue life of the corroded specimens as is given in Table 3, to the stress range, Δσ, which represents the nominal stress range used during the fatigue test of the corroded specimen as specified in Table 3:
SCF=ΔσSSΔσ=Notch tress rangeNominal stress range (4) Table 3 Experimental SCFsLoad group Specimen Number of cycles Δσ (Table 2) (MPa) ΔσSS (Eq. 3) (MPa) SCF (Eq. 4) A 6 552, 360 120 420 3.50 7 169, 023 120 623 5.19 8 915, 974 120 355 2.96 10 212, 719 120 577 4.81 B 9 368, 200 155 481 3.11 14 842, 368 155 365 2.36 17 315, 611 155 506 3.27 C 4 41, 143 200 998 5.00 11 69, 252 200 839 4.21 12 98, 715 200 746 3.74 13 119, 801 200 699 3.50 Thus, the SCFs, as calculated by the described procedure, are given in Table 3. As a consequence of highly scattered fatigue lives, the SCFs presented in Table 3 also exhibit widely scattered results.
3.2 SCF Dependency
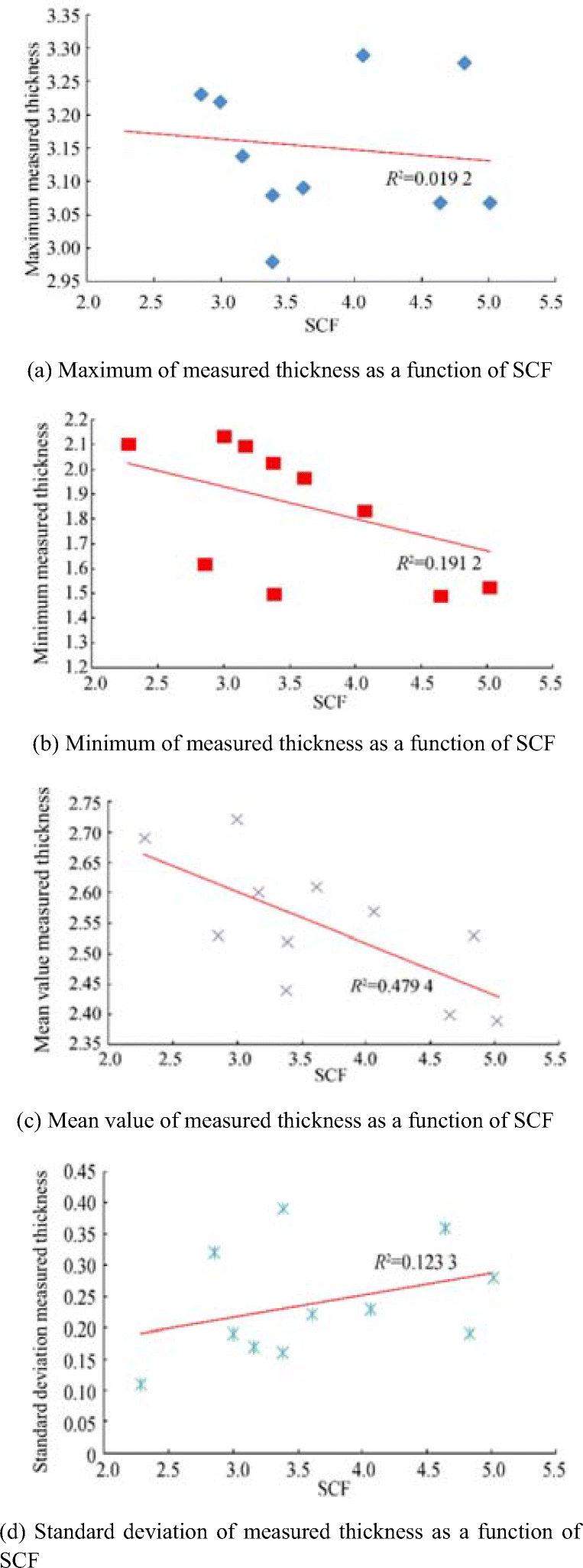
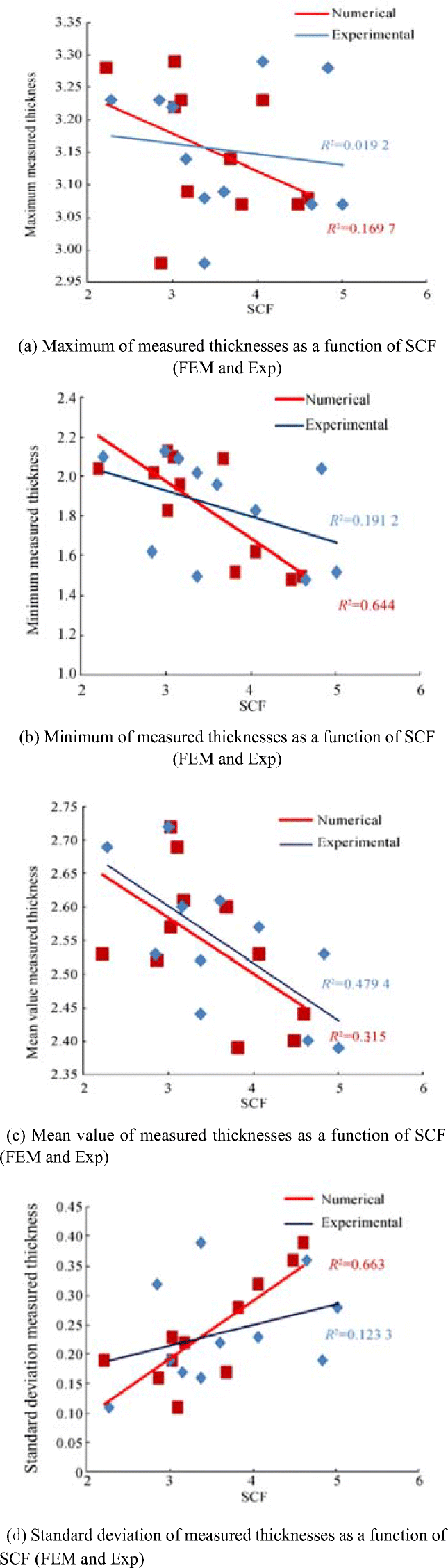
The dependence of the experimentally estimated SCFs (see Table 3) on several relevant statistical parameters of the corroded specimens is investigated here. Thus, a linear regression analysis concerning the maximum, minimum, mean and standard deviation of the measured thickness is performed. The results of the dependency analysis are presented in Figure 4.
The adequacy of the linear regression is estimated by the coefficient of determination R2, which is approaching 1 in the case of a perfect correlation between the regression model and measured values. As can be seen from Figure 4, a large scatter around the trend is observed and the only acceptable dependency is the one between the mean value of the thickness of the corroded specimen and the SCFs as R2, in that case, is 0.480. For all analysed parameters, except for the standard deviation, as the thickness goes down, the SCF grows, which may be explained by the fact that the corrosion pits become more in-depth and sharper leading to shorter fatigue lives. In the case of the standard deviation, as the corrosion degradation develops the standard deviations grow much faster than the mean value of the corrosion depth indicating a bigger scatter around the trend. For the much more corroded plates with a small resulting mean value of the corroded thickness, the observed standard deviation is more significant than for the one with lower corrosion degradation. This fact is also confirmed by Figure 4, where for a smaller mean value and more significant standard deviation of the corroded thickness, the SCF is bigger.
It must be clarified that the average thickness is the most relevant parameter because it is associated with the stress levels in the specimen. However, the average thickness was estimated from measurements before the experiments were performed, and the imposed force is adjusted to have approximately the target average stress level in the specimen (see Table 2). Increasing SCF with decreasing mean thickness is, therefore, a consequence of the fact that higher corrosion wastage is correlated with more frequent, more in-depth and sharper pits.
4 Finite Element Analysis of SCF
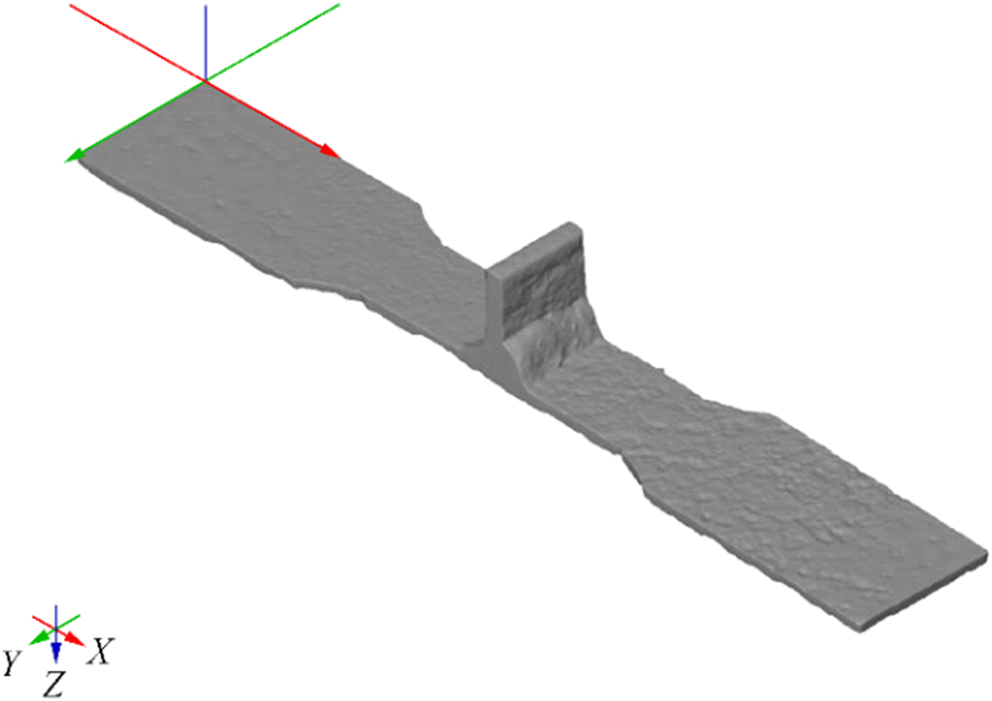
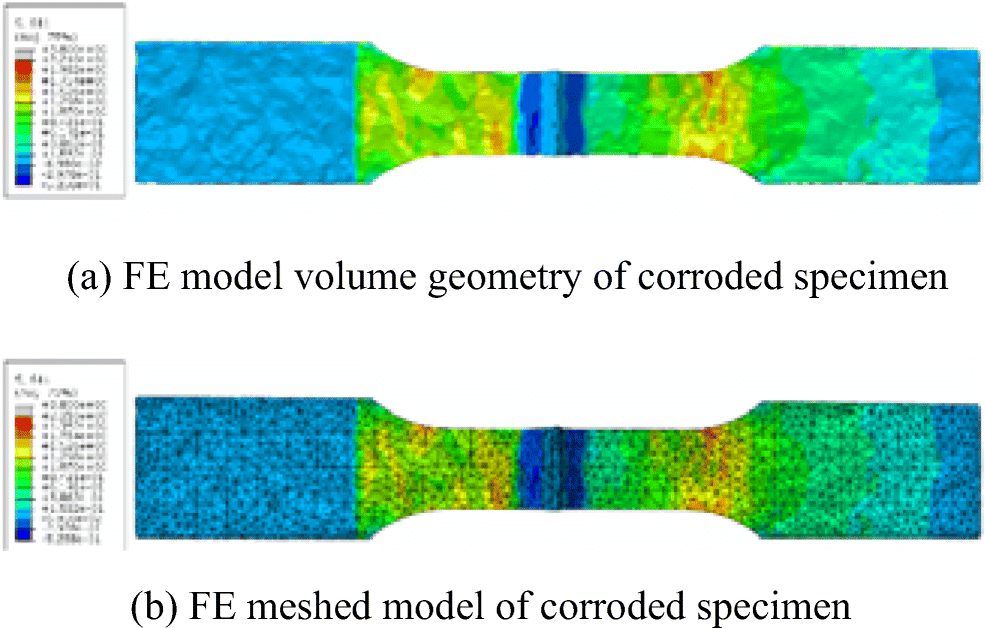
To investigate the SCFs of the corroded specimens, an FE analysis of the idealised geometries of the corroded specimens is performed using ABAQUS software. FE models are built based on the scanned geometries obtained in STL format. Surfaces are reconstructed using GeoMagic Spark software with the support of ProEngineer software. Finally, STP files are generated by closed volumes. The typical STP geometry of a specimen is presented in Figure 5.
The boundary conditions of the FE model are imposed to represent actual constraints of the specimen during the fatigue testing. One end of the specimen is completely fixed, while axial forces are imposed on the other end of the model. The magnitude of the imposed nominal stress in the cross-sectional area of the narrow part of the specimen is 1 MPa. The estimated average cross-sectional area of each specimen is taken into account, so the axial force is adjusted to reflect differences in the average corrosion wastage between individual specimens.
In the shipbuilding industry practice, the total stress at the anticipated crack location of the weld toe is calculated as (Parunov et al. 2013)
σnotch=KwKsΔσnom (5) where σnom is the nominal stress, calculated either by simple beam theory either by the coarse-mesh finite element analysis. The hot spot stress concentration factor, Ks, takes into account the effect of structural discontinuities and Kw is a notch factor. The product of the nominal stress σnom and stress concentration factor Ks (Δσhs = KsΔσnom) is known as the hot spot σhs or the structural stress. Ship classification societies establish a strict procedure on how to calculate this stress that includes standardisation of mesh size, finite element types and stress extrapolation (DnV 2010). The non-linear stress increase at the local notch is taken into account by the notch stress concentration factor Kw. In the fatigue analysis of ship structural details, Kw is usually proposed as a pre-defined factor, rather than requiring a very detailed finite element analysis. A difficulty raised with the calculation of the notch stress is that a 1 mm fictitious notch rounding should be modelled to estimate the stress increase at the weld toe. Consequently, a very fine mesh with an element size of 0.25 mm would be required.
In the present case of analysis of corroded specimens, the resulting SCF includes both types of stress concentrations. Pitting holes, irregularly distributed along the specimen surface, produce a structural discontinuity that causes stress concentrations. Although those places of stress concentration are away from the sharp corners, they have a similar notching effect as has a weld toe of intact non-corroded structural detail. Therefore, the calculation method employed should be capable of taking into account both types of stress concentration. Presently, there is not an approach standardised by either classification societies or other bodies interested in fatigue assessment of corroded structures (e.g. Haagensen and Maddox 2013; Hobbacher 2009). The present study aims to check the results of applying a 3D solid model with very fine mesh to model the corroded specimens with pits. This is possible nowadays due to the highly developed computing techniques and corresponding FE software solutions.
The analysis is subdivided into three tasks—firstly, a convergence study is performed on one of the specimens to identify the trends and the accuracy of results vs element size. The second step of the analysis is to estimate the SCFs of each specimen numerically and to compare with experiment, while the third task is to perform a comparative study of the regions of high stress concentrations from FE analysis and locations where the crack propagation was observed during the experimental fatigue test. The crack initiation starts in different locations for the fatigue tested specimens. This is explained by the fact that the surface roughness and pit penetrations are non-uniformly distributed and in all of the cases the crack initiation is not colliding with the weld toe hotspot, which usually occurs for the intact non-corroded specimens.
Principal stresses are recommended by ship classification societies for fatigue assessment (BV 1996; DnV 2010); therefore, the significant principal stresses are employed in the present study. However, the choice of some other stress components may be discussed as well. Tetrahedral elements of the second order with ten nodes (C3D10) are used in the modelling. Although hexahedral elements are the preferred element type regarding the accuracy of results, because of the slightly irregular shape of the surface, tetrahedral meshing could not be avoided.
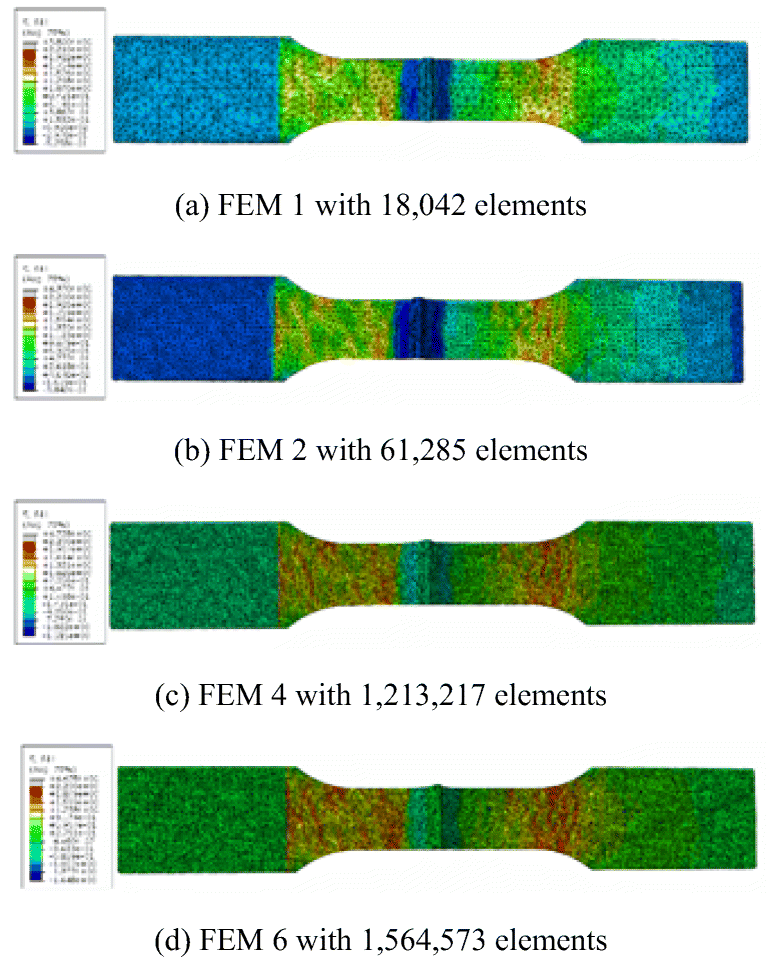
The convergence study is performed for the specimen C4 (Table 1). Six models with a progressively increasing number of elements are used, while the measure of the convergence is the displacement of the free edge of the specimen and the maximum SCF. Finite element models 1 (18, 042 elements), 2 (61, 285 elements), 4 (1, 213, 217 elements), and 6 (1, 564, 573 elements) are presented in Figure 6, where the colours represent principal stresses.
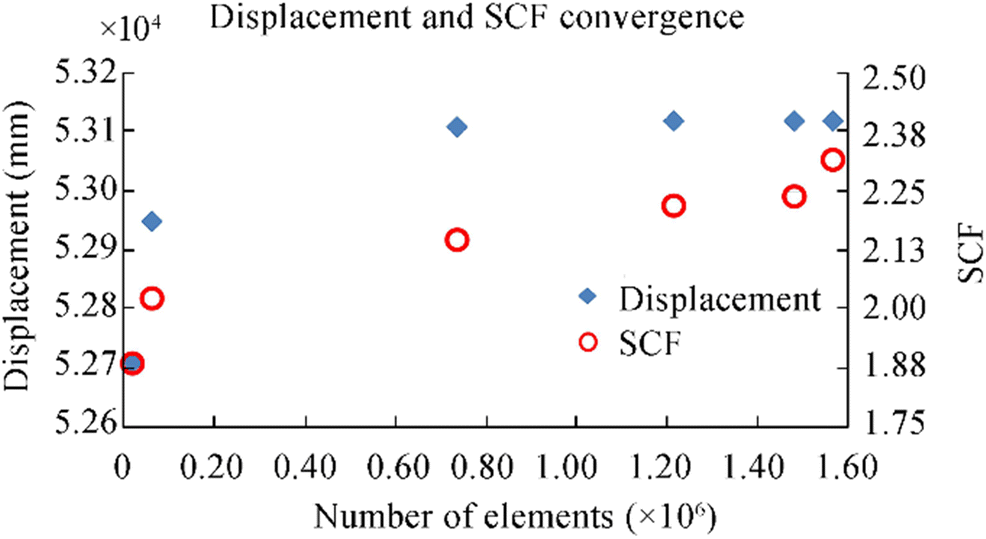
The number of finite elements used, several nodes, the typical length of the element edge and estimated maximum displacements and maximum SCF are given in Table 4 and Figure 7.
Table 4 Estimated displacements and maximum SCFs as a function of the FE mesh densityFEM Elements Nodes Size (mm) Displ (× 10−4) (mm) SCF 1 18, 042 32, 200 3.0 5.271 1.89 2 61, 285 99, 954 1.5 5.295 2.02 3 734, 931 1, 074, 280 0.5 5.311 2.15 4 1, 213, 217 1, 751, 270 0.4 5.312 2.22 5 1, 479, 414 2, 125, 439 0.37 5.312 2.24 6 1, 564, 573 2, 244, 825 0.36 5.312 2.32 As can be seen from Table 4 and Figure 7, the displacement convergence is achieved for the model 3, (734, 931 elements and 1, 074, 280 nodes). The convergence concerning the stresses is quite more complicated. Refining the element size, the maximum stresses are increasing, and they also appeared in different locations. As may be seen from Table 4 and Figure 7, the convergence of the maximum SCFs is also achieved for model 3 if the precision of the SCF is kept to one decimal place, with differences of about 5% in model 6. Such a level of accuracy may be assumed to be acceptable for the present study.
Accounting for the fact that the displacement and SCF convergence was well achieved for the FE model 3, FE model 4 (1, 213, 217 elements and 1, 751, 270 nodes) is considered as a reasonable and safe choice for the SCF analysis of the remaining corroded specimens. Finer models are not considered to be practical as the computational cost is increasing without providing substantially different results. Therefore, in the analysis of SCFs, the typical length of the element used in the modelling reads 0.4 mm (Table 4).
5 Comparative Analysis of SCF of Corroded Specimens
The experimental SCF is defined as a fictitious one, based on the number of cycle of the experimental fatigue life and the nominal stress range of the average corroded plate thickness. The stress range and number of cycles to failure relationship are defined as
ΔσmnotchN=K50 (6) where Δσnotchm is the notch stress estimated as
Δσnotch=1.45 SCF Δσnom (7) where the nominal stress range, Δσnom is as input data for the experiments (Table 2). K50 and m are given for the B S-N curve from the UK Department of Energy, where 1.45 is a factor taking into account that the B-curve includes "as-rolled", instead of a smooth specimen. The smooth specimen could have 1.45 higher fatigue strength (BV 1996).
The finite element analysis of the remaining specimens is performed using an FE model corresponding to the length of elements of 0.4 mm. The results of the FE analyses are presented in Table 5 and Figure 8, together with the experimentally estimated SCFs.
Table 5 SCFs estimated experimentally and by FEALoad group Specimen No. of elements No. of nodes SCFFEA SCFExp A 6 1, 317, 488 1, 898, 434 4.61 3.50 7 1, 350, 330 1, 939, 769 3.83 5.19 8 1, 318, 766 1, 900, 942 4.06 2.96 10 1, 356, 700 1, 954, 709 4.49 4.81 B 9 1, 212, 565 1, 752, 218 3.03 3.11 14 1, 221, 527 1, 761, 867 3.11 2.36 17 1, 207, 846 1, 744, 149 3.68 3.27 C 4 1, 213, 651 1, 751, 270 2.22 5.00 11 1, 170, 467 1, 694, 064 3.03 4.21 12 1, 254, 478 1, 823, 896 3.18 3.74 13 1, 219, 449 1, 759, 695 2.87 3.50 If the modelling error is defined as Φ = SCFFEM/SCFExp, then the mean value of Φ reads 1.00, while its standard deviation is 0.30. It may be stated that on average, the numerically estimated SCFs calculated by FEA predict the experimental SCFs accurately, while the dispersion of the predictions measured by standard deviation is rather large. However, it has to be pointed out that the experimentally estimated SCFs also have dispersion, which is of a comparable order of magnitude as the dispersion of Φ, then one may conclude that the numerical predictions of SCFs are reasonably accurate.
To further analyse the SCF estimates, the dependency of numerical SCFs on statistical properties of the corroded surface is determined and presented in Figure 9.
It can be observed in Figure 9 that the coefficients of determination R2 are generally much higher for the SCF estimated by FEA than for the ones experimentally estimated. The only regression trend line that shows somewhat lower R2 for SCFFEM than the one for SCFExp is on the mean value of corroded plate thickness. However, those two trend lines are almost identical, and they both present quite a clear trend of increasing SCFs with a reducing of the mean value of corroded plate thickness.
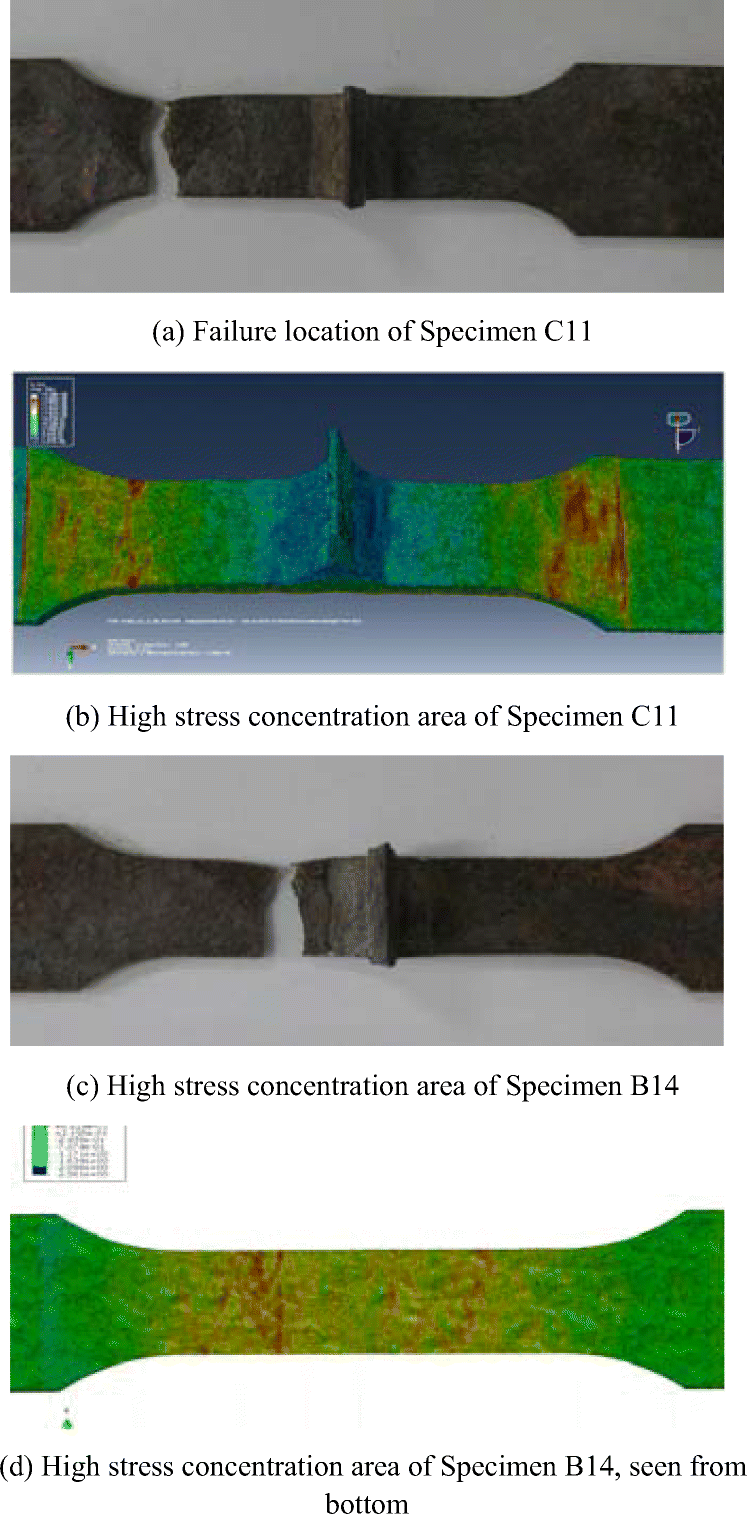
It is interesting to compare the actual failure location with areas of high stress concentrations calculated by FEA. As already elaborated, it is not always an easy task to establish such relationship as increased stress concentration in FE models is obtained in different locations, very often also on opposite sides of the model. Furthermore, SCFs observed at different locations are often reasonably close indicating various potential positions of the crack initiation and propagation. Therefore, careful analysis of stresses is necessary to establish a correlation with failure from the experiments. Typical situations are shown in Figure 10 for specimens C11 and B14. In the case of specimen C11, the stress concentration is visible in several locations along with the specimen. However, the stresses at the left upper transition from narrow to the wide part of the specimen are responsible for the failure. The FEM quite well predicts failure location. For the specimen B14, the predicted failure location is at the bottom of the specimen, not far away from the weld toe. Although there are other areas of elevated stresses in the FEM, the failure location is well predicted also in this case.
6 Conclusion
Analysis and comparative study of experimental and FE calculated SCFs of small-scaled corroded specimens was performed. The experimental SCFs are derived from experimentally estimated fatigue lives, by comparing it to the expected fatigue lives defined for perfectly smooth specimens. The SCFs were analysed by FEM using very fine 3D finite elements. The nodal plate thicknesses of FE models were identified by the photogrammetry method. A convergence study was performed to determine the element size and the accuracy of the estimated SCFs.
It was shown that the prediction of the experimental SCFs by the use of the FE analysis is unbiased, i.e. on an average, the calculated SCFs agree well with the experimental ones. However, the dispersion observed in the predictions is rather large, as measured by the standard deviation.
Linear regression trend lines, showing the dependency of SCFs on statistical properties of corroded surfaces, were generally better for calculated than for the experimentally estimated SCFs. It is interesting to notice that the trend lines presenting SCFs vs the mean value of the corroded specimen surface are almost identical for both SCFFEM and SCFExp.
Similar SCFs are observed at several locations along with the specimen, making it difficult to predict precisely the failure location by FE analysis. However, in most of the cases analysed, one of the areas with the increased stresses identified from FE results coincides with the actual failure position obtained from experiments.
-
Table 1 Corroded specimen thickness descriptors (Garbatov et al. 2014a)
Thickness (mm) Max Min Mean value St. Dev. A6 3.08 1.5 2.44 0.39 A7 3.07 1.52 2.39 0.28 A8 3.23 1.62 2.53 0.32 B9 3.22 2.13 2.72 0.19 A10 3.07 1.48 2.4 0.36 B14 3.23 2.1 2.69 0.11 B17 3.14 2.09 2.6 0.17 C4 3.28 2.04 2.53 0.19 C11 3.29 1.83 2.57 0.23 C12 3.09 1.96 2.61 0.22 C13 2.98 2.02 2.52 0.16 Table 2 Principal characteristics of corroded specimens (Garbatov et al. 2014a)
Load group Specimen R Δσ, MPa Frequency (Hz) Number of cycles A 6 0.05 120 10 552 360 7 0.05 120 10 169 023 8 0.05 120 10 915 974 10 0.05 120 10 212 719 B 9 0.05 155 10 368 200 14 0.05 155 10 842 368 17 0.05 155 10 315 611 C 4 0.05 200 10 41 143 11 0.05 200 10 69 252 12 0.05 200 10 98 715 13 0.05 200 10 119 801 Table 3 Experimental SCFs
Load group Specimen Number of cycles Δσ (Table 2) (MPa) ΔσSS (Eq. 3) (MPa) SCF (Eq. 4) A 6 552, 360 120 420 3.50 7 169, 023 120 623 5.19 8 915, 974 120 355 2.96 10 212, 719 120 577 4.81 B 9 368, 200 155 481 3.11 14 842, 368 155 365 2.36 17 315, 611 155 506 3.27 C 4 41, 143 200 998 5.00 11 69, 252 200 839 4.21 12 98, 715 200 746 3.74 13 119, 801 200 699 3.50 Table 4 Estimated displacements and maximum SCFs as a function of the FE mesh density
FEM Elements Nodes Size (mm) Displ (× 10−4) (mm) SCF 1 18, 042 32, 200 3.0 5.271 1.89 2 61, 285 99, 954 1.5 5.295 2.02 3 734, 931 1, 074, 280 0.5 5.311 2.15 4 1, 213, 217 1, 751, 270 0.4 5.312 2.22 5 1, 479, 414 2, 125, 439 0.37 5.312 2.24 6 1, 564, 573 2, 244, 825 0.36 5.312 2.32 Table 5 SCFs estimated experimentally and by FEA
Load group Specimen No. of elements No. of nodes SCFFEA SCFExp A 6 1, 317, 488 1, 898, 434 4.61 3.50 7 1, 350, 330 1, 939, 769 3.83 5.19 8 1, 318, 766 1, 900, 942 4.06 2.96 10 1, 356, 700 1, 954, 709 4.49 4.81 B 9 1, 212, 565 1, 752, 218 3.03 3.11 14 1, 221, 527 1, 761, 867 3.11 2.36 17 1, 207, 846 1, 744, 149 3.68 3.27 C 4 1, 213, 651 1, 751, 270 2.22 5.00 11 1, 170, 467 1, 694, 064 3.03 4.21 12 1, 254, 478 1, 823, 896 3.18 3.74 13 1, 219, 449 1, 759, 695 2.87 3.50 -
BV (1996) Fatigue strength of welded ship structures, Bureau Veritas DnV (2010) Fatigue assessment of ship structures, Classification Notes No 30.7 Domzalicki P, Skalski I, Guedes Soares C, Garbatov Y (2009) Large scale corrosion tests. In: Guedes Soares C, Das PK (eds) Analysis and Design of Marine Structures. London, Taylor & Francis Group, pp 193–198 Fricke W, Petershagen H (1992, 1992) Detail design of welded ship structures based on hot-spot stresses. In: Caldwell JB, Ward G (eds) Proceedings of the Practical Design of Ships and Mobile Units. Elsevier Science Limited, pp 1087–1100 Garbatov Y (2016) Fatigue strength assessment of ship structures accounting for a coating life and corrosion degradation. Int J Struct Integr 7: 305–322 Garbatov Y, Rudan S, Guedes Soares C (2002) Fatigue damage of structural joints accounting for nonlinear corrosion. J Ship Res 46: 289–298 https://doi.org/10.5957/jsr.2002.46.4.289 Garbatov Y, Guedes Soares C, Parunov J (2014a) Fatigue strength experiments of corroded small scale steel specimens. Int J Fatigue 59: 137–144 https://doi.org/10.1016/j.ijfatigue.2013.09.005 Garbatov Y, Guedes Soares C, Parunov J, Kodvanj J (2014b) Tensile strength assessment of corroded small scale specimens. Corros Sci 85: 296–303 https://doi.org/10.1016/j.corsci.2014.04.031 Guedes Soares C, Garbatov Y (1998) Reliability of maintained ship hull girders subjected to corrosion and fatigue. Struct Saf 20: 201–219 https://doi.org/10.1016/S0167-4730(98)00005-8 Guedes Soares C, Garbatov Y (1999) Reliability of corrosion protected and maintained ship hulls subjected to corrosion and fatigue. J Ship Res 43: 65–78 https://doi.org/10.5957/jsr.1999.43.2.65 Guedes Soares C, Moan T (1991) Model uncertainty in the long-term distribution of wave-induced bending moment for fatigue design of ship structure. Mar Struct 4: 295–315 https://doi.org/10.1016/0951-8339(91)90008-Y Guedes Soares C, Garbatov Y, von Selle H (2003) Fatigue damage assessment of ship structural components based on the long-term distribution of local stresses. Int Shipbuild Prog 50: 35–56 Haagensen PJ, Maddox SJ (2013) IIW Recommendations on methods for improving the fatigue strength of welded joints: doc. IIW-2142-110. International Institute of Welding, Woodhead Publishing, Paris Hobbacher A (2009) Recommendations for fatigue design of welded joints and components, IIW doc. 1823-07, Welding Research Council Bulletin 520. International Institute of Welding, New York Jiang X, Guedes Soares C (2012) A closed form formula to predict the ultimate capacity of pitted mild steel plate under biaxial compression. Thin-Walled Struct 59: 27–34 https://doi.org/10.1016/j.tws.2012.04.007 Moan T, Ayala-Uraga E (2008) Reliability-based assessment of deteriorating ship structures operating in multiple sea loading climates. Reliab Eng Syst Saf 93: 433–446 https://doi.org/10.1016/j.ress.2006.12.008 Nakai T, Matsushita H, Yamamoto N (2006) Effect of pitting corrosion on the ultimate strength of steel plates subjected to in-plane compression and bending. J Mar Sci Technol 11: 52–64 https://doi.org/10.1007/s00773-005-0203-4 Niemi E (1992) Determination of stresses for fatigue analysis of welded components. Proceedings of the IIW, Conference on Design in Welded Construction. 57–74 Niemi E (1995) Recommendation concerning stress determination for fatigue analysis of weld components. Abington Publishing Niemi E, Fricke W, Maddox S (2004) Structural stress approach to fatigue analysis of welded components - designer's guide. IIW Doc. XIII-1819-00/XV-1090-01 Paik JK, Lee JM, Ko MJ (2003) Ultimate compressive strength of plate element with pit corrosion wastage. J Eng Maritime Environ 217: 185–200 Paris P, Erdogan F (1963) A critical analysis of crack propagation laws. J Basic Eng 85: 528–534 https://doi.org/10.1115/1.3656900 Parunov J, Ćorak M, Gilja I (2013) Calculated and prescribed stress concentration factors of ship side longitudinal connections. Eng Struct 52: 629–641 https://doi.org/10.1016/j.engstruct.2013.03.027 Petershagen H, Fricke W, Massel T (1991) Application of the local approach to the fatigue strength assessment of welded structures in ships. Int Inst Weld Radaj D (1990) Design and analysis of fatigue-resistant welded structures. Abington Publishing, Cambridge Radaj D, Sonsino CM, Fricke W (2009) Recent developments in local concepts of fatigue assessment of welded joints. Int J Fatigue 31: 2–11 https://doi.org/10.1016/j.ijfatigue.2008.05.019 Saad-Eldeen S, Garbatov Y, Guedes Soares C (2011) Corrosion-dependent ultimate strength assessment of aged box girders based on experimental results. J Ship Res 55: 289–300 https://doi.org/10.5957/jsr.2011.55.4.289 Saad-Eldeen S, Garbatov Y, Guedes Soares C (2013a) Effect of corrosion severity on the ultimate strength of a steel box girder. Eng Struct 49: 560–571 https://doi.org/10.1016/j.engstruct.2012.11.017 Saad-Eldeen S, Garbatov Y, Guedes Soares C (2013b) Effect of corrosion degradation on ultimate strength of steel box girders. Corros Eng Sci Technol 47: 272–283 Silva JE, Garbatov Y, Guedes Soares C (2013) Ultimate strength assessment of rectangular steel plates subjected to a random localised corrosion degradation. Eng Struct 52: 295–305 https://doi.org/10.1016/j.engstruct.2013.02.013 Tran Nguyen K, Garbatov Y, Guedes Soares C (2012) Fatigue damage assessment of corroded oil tanker details based on global and local stress approaches. Int J Fatigue 43: 197–206 https://doi.org/10.1016/j.ijfatigue.2012.04.004 Tran Nguyen K, Garbatov Y, Guedes Soares C (2013) Spectral fatigue damage assessment of tanker deck structural detail subjected to time-dependent corrosion. Int J Fatigue 48: 147–155 https://doi.org/10.1016/j.ijfatigue.2012.10.014 TSCF (1992) Condition evaluation and maintenance of tanker structures, tanker structure co-operative forum. Witherby & Co, London TSCF (1997) Guidance Manual for Tanker Structures, Tanker Structure Cooperative Forum Wang G, Spencer J, Elsayed T (2003) Estimation of corrosion rates of structural members in oil tankers. Proceeding of 22nd International Conference on Offshore Mechanics and Arctic Engineering, Paper OMAE2003–37361. ASME Yamamoto N, Ikagaki K (1998) A study on the degradation of coating and corrosion on ship's hull based on the probabilistic approach. J Offshore Mech Arctic Eng 120: 121–128 https://doi.org/10.1115/1.2829532