1 Introduction
Composite vertical breakwaters have various advantages in deep water conditions. They are less expensive, fast construction and minimum maintenance in comparison with rubble mound breakwaters(Takahashi, 2002). The rate of failure in these types of structures is lower than rubble mound breakwaters, but the renovation after failure is very difficult. Therefore, safe design and construction of this type of breakwaters is crucial. The forces exerted by wind-generated waves on breakwaters and allied structures have been the subject of various theoretical and experimental studies. Most of the efforts have been directed towards assessing the effects of waves on rubble-mound breakwaters and the forces exerted on the upright section of vertical breakwaters. The effects of dynamic response of vertical breakwaters subject to breaking wave loads have been investigated by many scholars, e.g., Oumeraci and Kortenhaus(1994), Goda(1994), Lamberti and Martinell(1998), Ulker et al.(2010), Cuomo et al.(2011). However, one of the most important failures of composite breakwaters is related to erosion of foundation(Oumeraci, 1994a) and apparently few researchers have focused on that and the systematic studies of the well-recognized formula for erosion of the composite vertical breakwater foundations have no consensus in the literature.
Experimental research on sliding of a caisson breakwater carried out by Ruol et al.(2014)indicates that the bearing capacity of rubble mound decreased by about 50% when affected by the horizontal seepage force in comparison with the condition without seepage flow. Besides, the interaction mechanism between breaking waves, seabed foundation and composite breakwater have been investigated numerically(Ye et al., 2014a). This research shows the effect of liquefaction in porous seabed foundations, which govern the dynamic behaviors of seabed foundation and composite breakwater under breaking wave loading. The nonlinear interaction mechanism between ocean wave, a composite breakwater and its loose elasto-plastic s and bed foundation is investigated by utilizing a semi-coupled numerical model FSSI-CAS 2D(Ye et al., 2014b). Moreover, Esteban et al., (2012)research regarding stability of rubble mound breakwaters against solitary waves and existing problems when attempting to apply the Van der Meer formula and the research on stability of armor units covering rubble mound of composite breakwaters against a steady overflow of tsunami was investigated(Mitsui et al., 2014).
De Best et al.(1971), Xie(1981; Xie1985), Irie and Nadaoka(1984), and Hughes and Fowler(1991)investigated the scour in front of a vertical-wall breakwater in the case of 2D. The key factor is the action of st and ing waves that lead to a steady streaming pattern in the vertical plane. This presumably results in a distinct scour and deposition pattern in front of the breakwater in the form of alternating scour and deposition areas lying parallel to the breakwater(Oumeraci et al., 1994b).
Brebner and Donnelly(1962)investigated the influence of sea waves on rubble mound foundation of vertical breakwaters, using a 2D wave flume and showed that a rubble unit will be subjected to disturbing forces with horizontal and vertical components. Horizontal inertia and horizontal drag force must be equal to the buoyant weight plus vertical inertia and vertical drag force with multiplying friction coefficient. Eventually, a formula linked with a graph based on experimental tests under regular wave condition was derived. Tanimoto et al.(1982)carried out a number of irregular wave tests that used Bretchneider spectrum on composite breakwater foundation and discussed the stability number defined by Hudson(1959). A formula for the stability number of foundation quarry stones was achieved. Afterwards, Kimura et al.(1994)promoted the mentioned formula using 3D model experiments under oblique waves because it was assumed that 3D effects of waves on scouring of rubble mound foundations was not negligible. Therefore, a comprehensive formula was proposed.
Reviewing the existing experimental research revealed that there is a significant scatter between the results of different formulas for the same sea state condition and structural parameters. The main intention of the present study is to investigate the influence of a number of parameters on the erosion of foundation in order to evaluate the proposed formulas in the literature and see if any of them is more reliable according to wave tests.
Therefore, a series of 2D model tests was established in a wave flume. The effects of several structural parameters such as berm width and stone diameter, sea state conditions such as wave height and period, and water depth on the foundation were investigated.
In the following sections, first the experimental set-up is described. Next, the range of the parameters variation is given in Section 3. Section 4 gives the procedure for deriving the proposed formula for estimation of the stability number. Lastly, the stability of a real project in one of the southern ports of Iran that is in phase of construction is discussed by employing different formulas.
2 Experimental set-up and structural designThe tests were carried out in a 1 m wide, 1 m deep and 16 m long wave flume at the Hydraulic Laboratory of Tarbiat Modares University where some other research have been already carried out, e.g. Shafieefar and Shekari(2014) and Moghim et al.(2011). All the length of the tank is equipped with transparent glass panels for easier observations and photography, see Fig. 1. The seaward and l and ward slope of the foundation was 1:1.5 in all experiments. The tests were managed with irregular waves to observe the behavior of the foundation, applying Joint North Sea Wave Project(JONSWAP)spectrum with a peak enhancement factor γ of 3.3. A series of four wave gauges was used along the channel according to the Mansard and Funke(1980)pattern. The first three sensors near the paddle were used for calculation of wave reflection coefficients and the fourth sensor was placed to determine the height of waves near the structure. Extracting the reflected waves from the incident waves figured out that the reflection coefficient is approximately in the range of(0.55-0.65).
To measure the eroded area of the foundation, a wave profiler system including a vertical point gauge was used in three lines manually and for each test and pictures were captured from each side of the foundation.
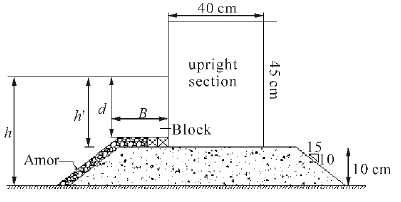
Fig. 2 shows the tested cross-section situated at the end of the channel that was tested for different conditions. Core height from the structure bottom was 10 cm in all tests. Forty-five tests were used to determine the influence of berm width, armor stone size, wave height and period on the stability of the foundation. Table 1 shows the test program for various hydrodynamic and structural parameters.

|
| Fig. 1 Wave flume longitudinal section |
|
| Fig. 2 Cross-section of the composite breakwater model |

|
| Fig. 3 Sample test, erosion less than 5% |
| Parameters | Structural parameters | ||||||
| Caisson dimensions | Berm width | Slope | Height of core | Stones size | Specific weight | ||
| Number of changes | Constant | 3 | 1 | 1 | 3 | 1 | |
| Parameters | Hydrodynamic parameters | ||||||
| Wave height | Wave period | Depth of water | |||||
| Number of changes | 3 | 3 | 3 | ||||
The stability of a foundation is traditionally expressed by stability number, Ns=Hs/(Δ×Dn50), where Hs is significant wave height, Δ =ρs/ρw−1 is relative buoyant density of armor, ρs is the mass density of armor unit, ρw is the water mass density, and Dn50 is the equivalent cube dimension exceeded by 50% of the armor stone units. Another parameter that denotes the armor damage level is expressed by S=Ae/(Dn50)2 similar to that used by Van der Meer(1987), where Ae is the eroded area of foundation. Coastal engineering manual(ENGINEERS U.A.C.O., 2006)defined the range of 2%-5% for the maximum allowable percentage of eroding in armor layer. Thus 5% is considered in all tests as the maximum acceptable value. Fig. 3 shows a case that erosion is less than 5%. A summary of material properties for all tests is given in Table 2 and the range of dimensional and non-dimensional parameters covered in the tests is shown in Tables 3 and 4.
| Core | Armor 3 | Armor 2 | Armor 1 | Item |
| 2700 | 2600 | 2600 | 2600 | Mass density |
| ρs /(kg∙m−3) | ||||
| 0.12 | 8.77 | 2.6 | 0.89 | Wn50 /gr |
| 0.0035 | 0.015 | 0.01 | 0.007 | Dn50 /m |
| Range | Parameter |
| 0.023-0.062 | Incident wave height Hs / m |
| 0.81-0.92 | Spectral peak wave period Tp / s |
| 0.08-0.15 | Berm width B /m |
| 0.14-0.18 | Water depth above core h' |
| 0.24-0.28 | Water depth h / m |
| Range | Parameter | |
| 1.70-6.65 | Ns | |
| 21.6-32.6 | To | |
| 3.20-28.6 | S | |
| 2.26-6.97 | h'/Hs | |
| 0.58-0.64 | h'/h | |
| 8.00-21.4 | Dn50/B | |
| 0.02-0.06 | Hs/L |
The effects of wave and structural parameters on the erosion are investigated according to the present experimental data. The composite breakwater model is considered as non-overtopped structure in all experiments.After performing each test and measuring the eroded area, the stability number is calculated for each test according to the generated wave height and stone diameter. To propose an appropriate formula for the stability number in terms of damage level, several algebraic functions were examined and ultimately the power function was selected as follows:

It is observed that, in this case the stability numbers of the performed tests have suitable trend to damage level with R-squared of 91%, see Fig. 4.
Rate of erosion: Due to the impact of waves in storm conditions, the seaside profile of the foundation is prone to be eroded. The eroded area would increase after a while until it reaches to a balance position. The rate of erosion can be divided into 3 parts. The first section is the acceptable rate of erosion, the second is the moderate part and the last section is the failure where the armor layer is completely eroded and there is no any protection for core particles. A sample test showing failure is given in Fig. 5.
Fig. 6 shows the percent of erosion versus damage parameter(S)for different Dn50/B. The maximum damage level for maximum erosion of 5% and for each dimensionless ratio of average diameter to berm, width(Dn50/B)is given in Table 5. Fig. 7 shows variation of damage level versus Dn50/B. According to this figure, following expression can be proposed to consider the effect of erosion on damage parameter:

| Dn/B | S |
| 0.125 | 6 |
| 0.083 | 10 |
| 0.058 | 17 |

|
| Fig. 4 Trend of damage in terms of stability number |

|
| Fig. 5 ample test, failure of foundation under an extreme wave condition |

|
| Fig. 6 Sketch of damage level in term of eroded for different dimension |

|
| Fig. 7 Interpolation of damage level for various dimensionless(Dn/B)in constant erosion of 5% |
As stated in the previous section, logical relationships can be established between the stability number and several parameters such as damage level and the rate of eroded area. According to Figs. 4 and 6, if the percentage of eroded area(less than 5%) and the ratio of stone diameter to berm width(Dn50/B)are known then the damage level can be evaluated and consequently, the stability number according to damage level is achievable and the stone diameter can be determined. This process of assuming and finding stone diameter should continue until they intersect to a nearly single point and ultimately the stability number will be gained. If the mentioned figures meet together in a same value of damage level, as it is shown in Fig. 8 then the process of trial and error is completed.
In order to find a more accurate stability number rather than graphical sketch, the equations from the previous sections can be employed as follow:
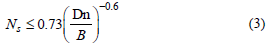
Eq. 3 is achieved through substituting Eq. 2 in Eq. 1, which shows the maximum stability number with the same limitations. The parameter of water depth is not presented in this formula because the range of h'/h mentioned in the Table 4 is limited to ranges(0.58-0.64), which is almost a practicable range for economical designing in real projects. Thus, apparently the formula is applicable in this range.
6 Comparison of the proposed formula with other referencesIn this section, the proposed formula for estimation of the stability number is compared with Kimura et al.(1994), which is given in the Overseas Coastal area Development Institute of Japan(OCDI). Fig. 9 reveals a comparison of observed and calculated stability number from Kimura formula. It can be comparatively seen that the present data are below the bisect line, which means that the results from Kimura formula has a greater stability number and smaller stone diameter for the same wave condition. Therefore, Kimura formula underestimates the stone size and should be used with caution. However, if stability number of Kimura formula multiplies an enhancement factor of 1.7 meters then the results would lead to a better correlation considering the present experimental data, see Fig. 10.
In order to compare application of the proposed formula and other formulas given in the literature, information of a caisson breakwater, which is under construction in Tombak at the northern coast of Persian Gulf is used. The design parameters of this breakwater are given in Table 6.
Designed cross section for the sub-structure of this breakwater is shown in Fig. 11. The weight of the core stone is in the range of(50-300 kg) and armor is in the range of(300-800 kg).

|
| Fig. 8 Relation between damage level and stability number for different Dn/B |

|
| Fig. 9 Comparison of Kimura formula's output and experiments |

|
| Fig. 10 Comparison of Kimura formula's results with safety factor of 1.7 meters and present data |
| Waves parameter | Geometrical parameter | ||||
| Hs / m | Hs / m | L / m | MHHW /(m∙CD) | Bed level /(m∙CD) | Water depth / m |
| 4.60 | 9 | 126.36 | 1.88 | −35 | 36.88 |
Firstly, redesigning has been done according to Kimura formula, which is based on some parameters such as water depth(h), distance between surface level and crown of core(h'), length of incident waves(L'), berm width(BM), height and period of waves and incident waves angle(β), as follows
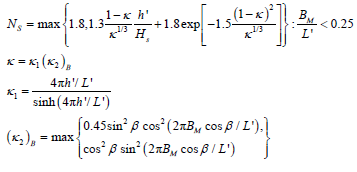

|
| Fig. 11 Designed cross-section for Tombak breakwater |
It is important to calculate and compare the results of different formulas in the same range of data inputs because the empirical tests were performed in some limited ranges and proposed formula is applicable in those ranges. In this caisson breakwater(Fig. 11)the ratio of(h'/Hs)is 2.97 and (h'/h)is 0.57 and the wave steepness is 0.05, which are in the range of present experimental tests. To determine the most critical phase the incident wave’s angle is assumed zero. The results are shown in Table 7.
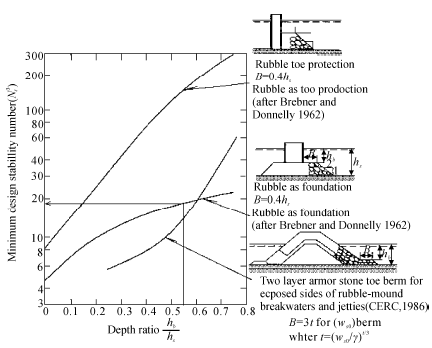
Second way to achieve stability number and stone diameter size for foundation is shown in the graph proposed in the CEM, see Fig. 12(Markle, 1989). By calculating the ratio of(h'/h), the stability number and consequently the stone diameter can be obtained, see Table 7.
|
| Fig. 12 Stability of toe berm tested in regular waves(Markle, 1989) |
Lastly, by assuming the stone diameter size the stability number can be achieved using proposed procedure. The ultimate stone diameter and stability number are gained with some trial and error. This process can be completed by using the proposed graph in Fig. 13.
Table 7 shows the comparison of different formulas for a real project carried out in Iran recently. The well-known Kimura formula indicates that the requisite stone diameter for this sea state is 0.67 m, while the proposed graph in CEM shows that the required diameter should be at least 1.3 meters which is a great difference.

|
| Fig. 13 Trial and error graph of different eroded for finding stone diameter in terms of damage level and stability number |
| Inputs | Kimura Eq. | CEM graph(Markle.1989) | Proposed Eq. | |||
| Ns | Dn50 / m | Ns | Dn50 / m | Ns | Dn50 / m | |
| h=23.96 m h'=13.7 m T=9 s B=11.2 m Hs=4.6 m | 5.25 | 0.67 | 2.66 | 1.3 | 2.65 | 1.3 |
Results from an experimental study with a total number of 45 tests are presented conjointly with a formula for estimation of the stability number as the most important parameter for designing the foundation of composite breakwaters. The results showed that the Kimura formula underestimates the stability number in the range of the present experimental data and need an enhancement factor of 1.7 meters to gain a proper estimation.
| Brebner A, Donnelly P (1962). Laboratory study of rubble foundations for vertical breakwaters. Coastal Engineering Proceedings, 1(8), 24. DOI: 10.9753/icce.v8.24 |
| Cuomo G, Lupoi G, Shimosako KI, Takahashi S (2011). Dynamic response and sliding distance of composite breakwaters under breaking and non-breaking wave attack. Coastal Engineering, 58(10), 953-969. DOI: 10.1016/j.coastaleng.2011.03.008 |
| De Best A, Bijker EW, Wichers JEW (1971). Scouring of sand in front of a vertical breakwater. Proc. Conference on Port and Ocean Engineering under Arctic Conditions Vol. 2, The Norwegian Institute of Technology, Trondheim, Norway, 1077-1086. |
| ENGINEERS U.A.C.O. (2006). Coastal engineering manual-Part VI.Publication Number EM 1110-2-1100, Proponent CECW-EW., Washington, DC. |
| Esteban M, Morikubo I, Shibayama T, Aranguiz-Muñoz R, Mikami T, Nguyen TD, Ohtani A (2012). Stability of rubble mound breakwaters against solitary waves. Coastal Engineering Proceedings, 1(33), Structures-9. DOI: 10.9753/icce.v33.structures.9 |
| Goda Y (1994). Dynamic response of upright breakwaters to impulsive breaking wave forces. Coastal Engineering, 22(1), 135-158. DOI: 10.1016/0378-3839(94)90051-5 |
| Hughes SA, Fowler JE (1991). Wave-induced scour prediction at vertical walls. ASCE Proc. Conf. Coastal Sediments 91, 1886-1899. |
| Hughes SA, Fowler JE (1995). Estimating wave-induced kinematics at sloping structures. Journal of Waterway, Port, Coastal, and Ocean Engineering, 121(4), 209-215. DOI: 10.1061/(ASCE)0733-950X(1995)121:4(209) |
| Hudson RY (1959). Laboratory investigations of rubble-mound breakwaters. American Society of Civil Engineers (ASCE). |
| Irie I, Nadaoka K (1984). Laboratory reproduction of seabed scour in front of breakwaters. Proc. 19th International Conference on Coastal Engineering, Houston, 2, 1715-1731.DOI: 10.9753/icce.v19 |
| Kimura K, Takahashi S, Tanimoto K (1994). Stability of rubble mound foundations of composite breakwaters under oblique wave attack. Coastal Engineering Proceedings, 1(24). DOI: 10.9753/icce.v24 |
| Lamberti A, Martinelli L (1998). Prototype measurements of the dynamic response of caisson breakwaters. Coastal Engineering Proceedings,1(26). DOI: 10.9753/icce.v26 |
| Mansard EP, Funke ER (1980). The measurement of incident and reflected spectra using a least squares method. Coastal Engineering Proceedings, 1(17). DOI: 10.9753/icce.v17 |
| Markle DG (1989). Stability of toe berm armor stone and Toe buttressing stone on Rubble-mound breakwaters and Jetties: physical model investigation. US Army Engineer Waterways Experiment Station. |
| Mitsui J, Matsumoto A, Hanzawa M, Nadaoka K (2014). Stability of armor units covering rubble mound of composite breakwaters against a steady overflow of tsunami. Coastal Engineering Proceedings, 1(34), Structures-34. DOI: 10.9753/icce.v34.structures.34 |
| Moghim MN, Shafieefar M, Tørum A, Chegini V (2011). A new formula for the sea state and structural parameters influencing the stability of homogeneous reshaping berm breakwaters. Coastal Engineering, 58(8), 706-721. DOI: 10.1016/j.coastaleng.2011.03.006 |
| Oumeraci H (1994a). Review and analysis of vertical breakwater failures—lessons learned. Coastal Engineering, 22(1), 3-29. DOI: 10.1016/0378-3839(94)90046-9 |
| Oumeraci H (1994b). Scour in front of vertical breakwaters—review of problems. Proc. International Workshop on Wave Barriers in Deep Water, Yokosuka, Japan, 281-307. |
| Oumeraci H, Kortenhaus A (1994). Analysis of the dynamic response of caisson breakwaters. Coastal Engineering, 22(1), 159-183. DOI: 10.1016/0378-3839(94)90052-3 |
| Ruol P, Martin P, Andersen TL, Martinelli L (2014). Experimental investigation on caisson breakwater sliding. Coastal Engineering Proceedings, 1(34), Structures-74. DOI: 10.9753/icce.v34.structures.74 |
| Shafieefar M, Shekari MR (2014). An experimental study on the parameterization of reshaped seaward profile of berm breakwaters. Coastal Engineering, 91, 123-139. DOI: 10.1016/j.coastaleng.2014.05.009 |
| Takahashi S (2002). Design of vertical breakwaters. Port and Airport Research Institute, Japan. |
| Tanimoto K, Yagyu T, Goda Y (1982). Irregular wave tests for composite breakwater foundations. Coastal Engineering Proceedings, 1(18). DOI: 10.9753/icce.v18.%p |
| Ulker MBC, Rahman MS, Guddati MN (2010). Wave-induced dynamic response and instability of seabed around caisson breakwater. Ocean Engineering, 37(17), 1522-1545. DOI: 10.1016/j.oceaneng.2010.09.004 |
| Van der Meer JW (1987). Stability of breakwater armour layers—design formulae. Coastal Engineering, 11(3), 219-239. DOI: 10.1016/0378-3839(87)90013-5 |
| Xie SL (1985). Scouring patterns in front of vertical breakwaters. Acta Oceanologica Sinica, 4(1), 153-164. |
| Xie SL (1981). Scouring patterns in front of vertical breakwaters and their influence on the stability of the foundations of the breakwaters. Report. Department of Civil Engineering, Delft University of Technology, Delft, The Netherlands, September, 61. |
| Ye JH, Jeng DS, Liu PF, Chan AHC, Wang R, Zhu CQ (2014a). Breaking wave-induced response of composite breakwater and liquefaction in seabed foundation. Coastal Engineering, 85, 72-86. DOI: 10.1016/j.coastaleng.2013.08.003 |
| Ye JH, Zhang Y, Wang R, Zhu CQ (2014b). Nonlinear interaction between wave, breakwater and its loose seabed foundation: A small-scale case. Ocean Engineering, 91, 300-315. DOI:10.1016/j.oceaneng.2014.09.003 |





