鱼雷在纵向平面内的运动方程具有明显非线性,一般来说,研究鱼雷非线性问题需要对运动方程进行不断地简化和限制。通常将非线性微分方程线性化,然后按线性方程进行运动分析和控制器设计,实践证明多数情况下线性方法可行[1]。但随着对鱼雷相关技术的研究不断深入,鱼雷运动中蕴涵的非线性因素越来越受到重视。如鱼雷纵向运动方程中所包含的流体动力系数都是通过试验测定,但随着作战使用环境以及鱼雷使用方式的不同,这些系数值会发生变化[2],甚至会导致鱼雷运动由稳态跳变到不稳态,现实中表现为跳水或沉底[3]。因此,研究鱼雷在流体动力参数存在扰动时的运动稳定性,有利于改善鱼雷的运动特性,匹配鱼雷机动性与稳定性。近年来,对水下航行体运动稳定性的分叉研究工作已经展开。文献[5]和文献[6]以横舵角为分叉参数,分别对鱼雷和潜艇纵向运动的跨临界分叉进行研究;文献[7]以控制器参数为分叉参数,对鱼雷爬潜运动的跨临界分叉进行研究;文献[8]和文献[9]分别以控制器参数和空化数为分叉参数,对水下高速运动体纵向运动的Hopf分叉进行研究;文献[10]以流体动力参数为分叉参数,对鱼雷在非控状态下纵向运动的跨临界分叉和Hopf分叉进行研究;文献[11]对水下航行体的纵倾航行稳定性进行了研究分析。上述工作在研究属静态分叉的跨临界分叉时,都给出了系统的分叉范式;而研究属动态分叉的Hopf分叉时,却未给出系统的解析表达式,而是通过数值仿真证明了Hopf分叉的存在。
本文以目前常见的采用比例微分控制的鱼雷为研究对象,将鱼雷定深运动模型中所包含的某一流体动力参数的扰动作为分叉参数,利用中心流形定理推导出鱼雷定深运动各状态参量的解析表达式,对系统的Hopf分叉进行研究,并进行仿真验证。可揭示比例微分控制下,鱼雷定深运动失稳的本质原因,并完善鱼雷航行稳定性分析理论体系。
1 鱼雷定深运动方程鱼雷定深运动方程由文献[1]给出:
| $ \left\{ {\begin{array}{*{20}{l}} {\dot y = v\sin (\theta -\alpha )}\text{,} \\ {m\dot v = (B-mg)\sin (\theta -\alpha ) + T-D}\text{,} \\ {mv(\dot \theta -\dot \alpha ) = (B-mg)\cos (\theta -\alpha ) + T\alpha + L}\text{,}\\ {{J_z}{{\dot \omega }_{zB}} = N-B({Y_b}\sin \theta -{X_b}\cos \theta )-T{Y_T}\text{,}\quad \;} \\ {\dot \theta = \omega\!\!\!\!\!\!{\quad _{zB}}} \text{;} \end{array}} \right. $ | (1) |
将其化为如下标准形式[1]:
| $ \left\{ {\begin{aligned} & {\dot y = v\sin (\theta -\alpha )}\text{,} \\ & {\dot v = {k_{11}}{v^2} + {k_{14}}\sin (\theta -\alpha ) + {k_{18}}}\text{,}\\ & \dot \alpha \! = 1/v({k_{21}}{v^2}\alpha + {k_{22}}v{\omega _{zB}} + {k_{23}}\alpha +\\ & \quad \quad \!\! {k_{24}}\alpha \sin (\theta -\alpha ) + {k_{25}}\cos (\theta -\alpha )+ \\ & \quad \quad \!\! {k_{26}}\cos \theta + {k_{27}}\sin \theta + {k_{28}} + {k_{29}}{v^2}{\delta _e})\text{,}\\ & {{\dot \omega }_{zB}} = {k_{31}}{v^2}\alpha + {k_{32}}v{\omega _{zB}} + {k_{34}}\alpha \sin (\theta -\alpha )+ \\ & \quad \quad \, \, \, \, {k_{35}}\cos (\theta -\alpha ) + {k_{36}}\cos \theta + \\ & \quad \quad \, \, \, \, {k_{37}}\sin \theta + {k_{38}} + {k_{39}}{v^2}{\delta _e}\text{,}\\ & {\dot \theta = {\omega _{zB}}} \text{。} \end{aligned}} \right. $ | (2) |
式中:y为鱼雷航深;v为鱼雷速度;α,θ和Θ分别为攻角、俯仰角及弹道倾角;
| $ {\delta _e} = {k_y}(y-{y_0}) + {k_\theta }(\theta -{\theta _0}) + {\delta _{e0}}\text{。} $ | (3) |
式中:y0为设定航深;θ0为平衡俯仰角;
令
| $ \dot x = G(x, \Delta m)\text{,} $ | (4) |
在平衡点处利用泰勒级数展开,我们可以得到新的系统:
| $ \dot x = A(\Delta m)x + F(x, \Delta m)\text{,} $ | (5) |
式中:F(x, ∆m)为系统的高阶项;A(∆m)由下式可得:
| $ A(\Delta m) = {\left. {\frac{{\partial {G_i}}}{{\partial {x_j}}}} \right|_{x = 0}}(i, j = 1, 2, 3, 4, 5)\text{,} $ | (6) |
以国外某型鱼雷为例,其总体和流体动力参数见文献[12],设鱼雷以航速30 kn进行定深直航,将以上参数值代入式(4)和式(6)可得:
| $ {\boldsymbol{A}} \!=\! \left[\!\! {\begin{array}{*{20}{c}} \! 0 &\! 0 &\! {-15.42} & \!0 & \!{15.42} \\ \! 0 &\! {-0.27} & \!{1.83} &\! 0 & \!{-1.83} \\ \!{-0.02} &\! {-0.01} & \!{-1.37} &\! {0.12-0.25\Delta m} &\! {-0.47} \\ \! {-0.40} & \!{0.01} & \!{11.16} &\! {-7.95 + 0.17\Delta m} & \!{-9.04} \\ \! 0 & \!0 &\! 0 & \!1 &\! 0 \end{array}} \!\!\right]\text{。} $ |
参考
作线性变换y=p-1x,p为
| $ \begin{gathered} \left[{\begin{array}{*{20}{c}} {{{\dot y}_1}} \\ {{{\dot y}_2}} \\ {{{\dot y}_3}} \\ {{{\dot y}_4}} \\ {{{\dot y}_5}} \end{array}} \right] = \left[{\begin{array}{*{20}{c}} {-0.27} & {} & {} & {} & {} \\ {} & {-9.02} & {} & {} & {} \\ {} & {} & {-1.23i} & {} & {} \\ {} & {} & {} & {-0.88} & {} \\ {} & {} & {} & {} & {1.23i} \end{array}} \right]\text{×}\\ \left[{\begin{array}{*{20}{c}} {{y_1}} \\ {{y_2}} \\ {{y_3}} \\ {{y_4}} \\ {{y_5}} \end{array}} \right] + F'\left( {y, \Delta m} \right) \text{,} \quad \quad \quad \quad \quad \\ \end{gathered} $ | (7) |
式中
| $ \left\{ \begin{gathered} \dot v = \boldsymbol{B}v + \boldsymbol{M}(v, w) \hfill\text{,}\\ \dot w = \boldsymbol{C}w + \boldsymbol{N}(v, w) \hfill \text{。}\\ \end{gathered} \right. $ | (8) |
式中:B为2 × 2矩阵;C为3 × 3矩阵。它们分别对应A(∆m)的零实部和负实部特征值,可将其表示为:
| $ w = h(v), \;h(0) = Dh\;(0) = 0 \text{。} $ |
令
| $ \begin{gathered} {a_{1, 2, 3}} = -0.008-0.006i, 0.013 + 0.009i, - 0.002-0.021i \hfill\text{,} \\ {b_{1, 2, 3}} = -0.008 + 0.006i, 0.013-0.009i, - 0.002 + 0.021i \hfill\text{,} \\ {c_{1, 2, 3}} = -0.064, 0.098, - 0.034\text{。} \hfill \\ \end{gathered} $ |
从而进一步得到:
| $ \begin{aligned} {{\dot y}_3} = & -1.234i{y_3} + (0.037 + 0.054i)y_3^2 + \\ & (0.007 + 0.003i)y_5^2 + (0.023 + 0.114i){y_3}{y_5}\text{,} \\ {{\dot y}_5} = & 1.234i{y_5} + (0.007-0.003i)y_3^2+ \\ & (0.037-0.054i)y_5^2 + (0.023-0.114i){y_3}{y_5}\text{。} \\ \end{aligned} $ | (9) |
经分析可知y3和y5是共轭复根,进行如下坐标变换:
| $ {y_3} = r(\cos o + i\sin o), \;{y_5} = r(\cos o-i\sin o)\text{,} $ | (10) |
将式(10)代入式(9)整理可得:
| $ \begin{aligned} \frac{1}{r} = & \frac{1}{{1.23}}(1 + 0.007\sin o{\cos ^2}o +\\ & 0.160{\sin ^2}o\cos o-0.021{\sin ^3}o + 0.171{\cos ^3}o)\text{,} \\ \end{aligned} $ | (11) |
相应地可求得x的表达式为:
| $ \left\{ \begin{gathered} {x_1} = -2.84{r^2}{\cos ^2}o-1.69{r^2}{\sin ^2}o-5.18r\sin o +\\ 0.86{r^2}\sin o\cos o + 28.03r\cos o \text{,}\\ {x_2} = -0.35{r^2}{\cos ^2}o-0.20{r^2}{\sin ^2}o + 1.28r\sin o +\\ 0.11{r^2}\sin t\cos o-3.04r\cos o \text{,}\\ {x_3} = -0.11{r^2}{\cos ^2}o-0.07{r^2}{\sin ^2}o-0.88r\sin o +\\ 0.08{r^2}\sin o\cos o-1.69r\cos o \text{,}\\ {x_4} = 0.12{r^2}{\cos ^2}o + 0.10{r^2}{\sin ^2}o + 2.60r\sin o -\\ 0.36{r^2}\sin o\cos o-1.68r\cos o \text{,}\\ {x_5} = 0.06{r^2}{\cos ^2}o + 0.03{r^2}{\sin ^2}o-1.36r\sin o +\\ 0.02{r^2}\sin o\cos o-2.11r\cos o\text{。} \\ \end{gathered} \right. $ | (12) |
分析式(11)和式(12)可知,此时x=[x1, x2, x3, x4, x5]T是关于参量O的周期函数,系统从平衡点处产生极限环即发生Hopf分叉。Hopf分叉是动态分叉的典型形式, 指参数∆m经过分叉点时,系统的平衡点由渐近稳定的焦点变成不稳定焦点,并产生孤立周期运动的突变现象[14]。
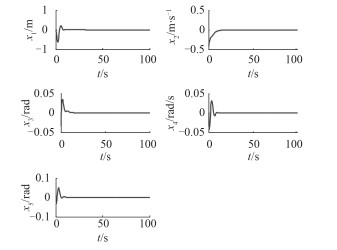
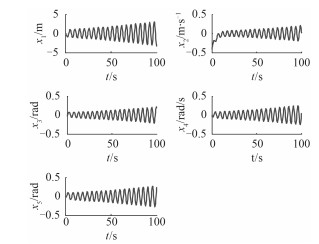
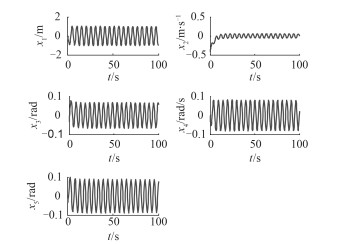
3 数值仿真为了验证分叉分析结果,进行数值仿真。设鱼雷的初始速度为15 m/s,发射深度与设定航深相同,其他状态参量初始值都为0,选择不同的流体动力系数干扰值∆m,进行计算机仿真,图 1~图 3为式(4)在∆m取不同值时的状态响应曲线。
|
图 1 ∆m=0时,系统响应曲线 Fig. 1 The curve of system response when ∆m=0 |
|
图 2 ∆m=-3.50时,系统响应曲线 Fig. 2 The curve of system response when ∆m=-3.50 |
|
图 3 ∆m=-3.40时,系统响应曲线 Fig. 3 The curve of system response when ∆m=-3.40 |
分析图 1~图 3可知,当
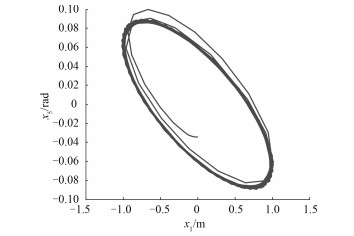
|
图 4 ∆m = -3.40 时,平衡点处极限环 Fig. 4 Limit cycle at equilibrium point when ∆m = -3.40 |
鱼雷定深运动方程中,含有各种非线性项和耦合项,为方便起见,在研究中经常对非线性的影响进行忽略和简化。分析非线性问题是鱼雷航行动力学与控制的研究分支之一,本文运用分叉理论处理鱼雷定深航行的非线性问题,该方法不需要过于简化方程,利用等价变换将高维系统约化到低维的包含了定深航行稳定性全部信息的中心流形上来进行研究。文中选择某一流体动力参数扰动值为分叉参数,推导出了状态变量的解析表达式,讨论鱼雷在定深航行中的Hopf分叉现象,并进行数值仿真验证。研究表明,流体动力系数变化会对鱼雷的定深航行稳定性产生影响,该结果可用来解释鱼雷发生跳水、沉底等现象的原因,同时也可为研究非线性因素影响下的鱼雷运动稳定性提供分析工具。
| [1] |
徐德民.
鱼雷自动控制系统[M]. 西安: 西北工业大学出版社, 2001 .
XU De-min. Autocontrol system of torpedo[M]. Xi'an: Northwestern Polytechnical University Press, 2001 . |
| [2] |
黄景泉, 张宇文.
鱼雷流体力学[M]. 西安: 西北工业大学出版社, 1989 .
HUANG Jing-quan, ZHANG Yu-wen. Torpedo hydrodynamics[M]. Xi'an: Northwestern Polytechnical University Press, 1989 . |
| [3] | CHEN G R, MOIOLA J L, WANG H O. Bifurcation control:theories, methods, and applications[J]. International Journal bifurcation and Chaos , 2000, 10 (3) :511–548. DOI:10.1142/S0218127400000360 |
| [4] |
朱新坚, 邵惠鹤, 张钟俊. 一类非线性系统Hopf分叉的控制[J]. 上海交通大学学报 , 1997, 31 (6) :52–55.
ZHU Xin-jian, SHAO Hui-he, ZHANG Zhong-jun. Control of Hopf bifurcation in a certain nonlinear system[J]. Journal of Shanghai Jiaotong University , 1997, 31 (6) :52–55. |
| [5] |
杨明, 王德石, 蒋兴舟. 鱼雷纵向运动的分叉特性分析[J]. 兵工学报 , 2001, 22 (3) :338–341.
YANG Ming, WANG De-shi, JIANG Xing-zhou. Bifurcation analysis for the nonlinear longitudinal motion dynamics of a torpedo[J]. Acta Armamentarii , 2001, 22 (3) :338–341. |
| [6] |
王晓玢, 孙尧, 莫宏伟. 潜艇垂直面运动突变分析[J]. 大连海事大学学报 , 2008, 34 (4) :55–58.
WANG Xiao-bin, SUN Yao, MO Hong-wei. Catastrophe analysis of submarine motion in dive plane[J]. Journal of Dalian Maritime University , 2008, 34 (4) :55–58. |
| [7] | DING H, WANG D S. The sailing stability of autonomous underwater vehicle with pitch controller[C]//Proceedings of 2009 IEEE International Conference on Mechatronics and Automation. Changchun:IEEE, 2009:4790-4794. |
| [8] |
白涛, 孙尧, 莫宏伟. 分叉分析在水下高速运动体稳定控制中的应用[J]. 哈尔滨工程大学学报 , 2008, 29 (10) :1067–1075.
BAI Tao, SUN Yao, MO Hong-wei. Application of bifurcation analysis to the stability control of underwater high-speed vehicles[J]. Journal of Harbin Engineering University , 2008, 29 (10) :1067–1075. |
| [9] |
白涛, 孙尧, 莫宏伟. 水下高速运动体运动稳定性的分叉分析[J]. 哈尔滨工业大学学报 , 2009, 41 (5) :95–98.
BAI Tao, SUN Yao, MO Hong-wei. Bifurcation analysis of motion stability for high-speed underwater vehic[J]. Journal of Harbin Institute of Technology , 2009, 41 (5) :95–98. |
| [10] |
杨明, 王德石, 蒋兴舟. 鱼雷大攻角运动的分叉分析[J]. 鱼雷技术 , 2000, 8 (2) :4–7, 17.
YANG Ming, WANG De-shi, JIANG Xing-zhou. Bifurcation analysis for pitch motion dynamics of a torpedo[J]. Torpedo Technology , 2000, 8 (2) :4–7, 17. |
| [11] |
丁浩, 王德石. 水下航行体纵倾航行稳定性研究[J]. 力学季刊 , 2009, 30 (4) :597–601.
DING Hao, WANG De-shi. Study on pitch sailing stability of torpedo[J]. Chinese Quarterly of Mechanics , 2009, 30 (4) :597–601. |
| [12] |
严卫生.
鱼雷航行力学[M]. 西安: 西北工业大学出版社, 2005 .
YAN Wei-sheng. Torpedo sailing dynamics[M]. Xi'an: Northwestern Polytechnical University Press, 2005 . |
| [13] |
陆启韶.
分岔与奇异性[M]. 上海: 上海科技教育出版社, 1995 .
LU Qi-shao. Bifurcation and singularity[M]. Shanghai: Shanghai Science and Technical Education Press, 1995 . |
| [14] |
陈予恕.
非线性振动系统的分叉和混沌理论[M]. 北京: 高等教育出版社, 1993 .
CHEN Yu-shu. Bifurcation and chaos theory of nonlinear vibration systems[M]. Beijing: Higher Education Press, 1993 . |
 2016, Vol. 38
2016, Vol. 38
