2. Key Laboratory of Vacuum Physics, University of Chinese Academy of Sciences, Beijing 100049, China
2. 中国科学院大学真空物理重点实验室, 北京 100049
Correlations are the no-signaling probability distributions characterizing the outcomes of mea-surements performed on entangled quantum states. These no-signaling probability distributions could be characterized into local and quantum depending upon the violations of the Bell inequalities. The correlations which do not violate Bell inequalities, which are derived under the assumption of local realism, fall into the category of local correlations while quantum (nonlocal) otherwise.
Actually, nonlocality is the pronounced characteristic of quantum theory which supports many advantages afforded by a quantum processing of information and announces itself in the violations of various Bell inequalities[1-3], which have been verified in photons[4], atoms[5], and hybrid systems[6]. Very recently Bell-CH inequality has been generalized to test local realism in high energy physics[7]. The violation of which stamps the authority of the Bell's theorem as one of the profound discoveries in the science world at the level where quanta obey only the quantum theory.
In 2003, Leggett introduced a class of nonlocal model, i.e., relax the requirement of locality while still keep the realism[8], and formulated an incompatible theorem between the nonlocal realism and QM in terms of the Leggett inequality.Soon after, experiments with photon were performed and shown in conflict with the Leggett model and agree with the quantum predictions[9-12]. Lately, the falsifications of Leggett model using neutron matter wave and with solid state spins were carried out[13-14]. Unlike the Bell inequality, there is no explicit requirement for active measurement in obtaining the Leggett inequality. Recently a scheme has been proposed to test the nonlocal hidden variable theory by the Leggett inequality in high energy physics[15]. Again, which supports the statement, in the interactions which obey only the quantum theory, any future extension of the theory that is in agreement with the experiments must abandon certain features of realistic descri-ption[9].
Whereas, going from two parties to three makes the situation much complicated as the discussions about whether the nonlocality is genuine or weak join the party.So, in the multipartite case, nonlocality displays much richer or more complicated structure compared to the case of bipartite. This makes the study and the characterization of multipartite nonlocal correlations an interesting, but challenging problem. Although much work has been done for multipartite Bell nonlocality (most importantly Svetlichny, first attempt towards genuine tripartite nonloclaity[16]) but multipartite Leggett-type nonlo-cality stays silent.
So, the main question we ask for in this paper is, does Leggett model for tripartite exist? We build inequalities for three parties standard Bell scenario, visualization of the experimental setup could be seen in Fig. 1, and check for the violations for tripartite states. In this work we provide an inequality containing only tripartite correlations with one additional assumption that bipartite correlations are zero for the GHZ state when measurements are restricted in the xy-plane.Which raises an intriguing question.Could GHZ tripartite correlations be constrained from single party marginals? We think yes, hence the current work. While the other inequality, which contain both bipartite and tripartite correlations, provides constraints to check for the violations of the W state in the xz-plane. Conditions are presented to check for the violations of the arbitrary three-qubit pure state as well. Generali-zation of the both inequalities to more parties seem possible.

|
Download:
|
|
A common source distributes photons with well-defined polarizations u, v and w to the three parties A, B and C which perform measurements along a, b and c respectively. And the outputs are recorded at the coincidences detector Fig. 1 Visualization of the experimental setup |
|
Conjecture 1 If bipartite correlation function is a fully mixed point in the probability space then it would be zero.
Which is certianly possible using the approach used in Ref.[17], if inputs are restricted in the xy-plane bipartite correlations become zero. But according to this approach single side averages become zero as well. Such a case raises an interesting question that QM perdicts single side probabilities to be zero where as we are obtaining constraints from local averages. So, we are extending Leggett's original idea to the tripartite case, i.e., sharp properties at the individual level of three parties.
Using measurement settings shown in Fig. 2, the following inequality is obtained,
|
Download:
|
|
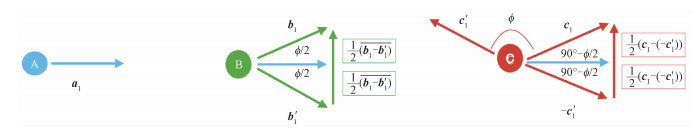
Alice has 3 measurement settings whereas Bob and Charlie have 6 settings each. Only one setting for Alice, which is in the middle of the two settings shown for Bob and in the middle of c1 and-c′1 for Charlie, is shown; similar settings for other two directions are chosen. For GHZ and the arbitrary three-qubit pure states measurements are restricted in xy-plane and for W state in xz-plane Fig. 2 Specific triple-measurement settings for the tripartite Leggett-type inequalities |
|
| $ \begin{gathered} \frac{1}{3} \sum\limits_{i=1}^3\left|E\left(\boldsymbol{a}_i, \boldsymbol{b}_i, \boldsymbol{c}_i\right)+E\left(\boldsymbol{a}_i, \boldsymbol{b}_i^{\prime}, \boldsymbol{c}_i^{\prime}\right)\right| \leqslant \\ 2-\frac{2}{3}\left(\sin \frac{\varphi}{2}+\cos \frac{\varphi}{2}\right) . \end{gathered} $ | (1) |
Here, tripartite correlation function is defined as,
| $ \begin{aligned} & E^{(\mathrm{ABC})}(\boldsymbol{a}, \boldsymbol{b}, \boldsymbol{c})=\int C_\lambda^{(\mathrm{ABC})}(\boldsymbol{a}, \boldsymbol{b}, \boldsymbol{c}) F(\lambda) \mathrm{d} \lambda= \\ & \int C_{\boldsymbol{u}, \boldsymbol{v}, \boldsymbol{w}}^{(\mathrm{ABC})}(\boldsymbol{a}, \boldsymbol{b}, \boldsymbol{c}) F(\boldsymbol{u}, \boldsymbol{v}, \boldsymbol{w}) \mathrm{d} \boldsymbol{u} \mathrm{d} \boldsymbol{v} \mathrm{d} \boldsymbol{w}, \end{aligned} $ | (2) |
and F(λ) is a distribution function of the source polarizations.
2 Constraints on bipartite and tripartite correlationsUsing measurement settings shown in Fig. 2, we get
| $ \begin{gathered} \frac{1}{3} \sum\limits_{i=1}^3 \mid E\left(\boldsymbol{a}_i, \boldsymbol{b}_i\right)+E\left(\boldsymbol{a}_i, \boldsymbol{b}_i^{\prime}\right)+E\left(\boldsymbol{a}_i, \boldsymbol{c}_i\right)- \\ E\left(\boldsymbol{a}_i, \boldsymbol{c}_i^{\prime}\right)-\left[E\left(\boldsymbol{a}_i, \boldsymbol{b}_i, \boldsymbol{c}_i\right)+\left[E\left(\boldsymbol{a}_i, \boldsymbol{b}_i^{\prime}, \boldsymbol{c}_i^{\prime}\right)\right] \mid-\right. \end{gathered} \\ \begin{gathered} \frac{1}{3} \sum\limits_{i=1}^3\left(E\left(\boldsymbol{b}_i, \boldsymbol{c}_i\right)-E\left(\boldsymbol{b}_i^{\prime}, \boldsymbol{c}_i^{\prime}\right)\right) \leqslant \\ 2-\frac{2}{3}\left(\sin \frac{\vartheta}{2}+\cos \frac{\vartheta}{2}\right) . \end{gathered} $ | (3) |
In our measurement settings Alice has three settings while Bob and Charlie have six settings each.
3 Comparison with the existing inequalityIn Ref.[18], Deng et al. made the first attempt, according to our knowledge, for multipartite Leggett-type inequalities and a tripartite inequality has been provided,
| $ \begin{gathered} \frac{1}{3} \sum\limits_{i=1}^3\left|E\left(\boldsymbol{a}_i, \boldsymbol{b}_i, \boldsymbol{c}_i\right)+E\left(\boldsymbol{a}_i^{\prime}, \boldsymbol{b}_i, \boldsymbol{c}_i\right)\right| \leqslant \\ 2-\frac{2}{3}\left|\sin \frac{\varphi}{2}\right|, \end{gathered} $ | (4) |
the shortcoming of which is that the bound does not change with the change of the parties. It has been generalized to n-parties as well but the bound stays the same even for n-parties.
4 QM expectation values and their violations 4.1 For GHZ stateFor a GHZ state of three spin-1/2 particle
| $ E(\boldsymbol{a}, \boldsymbol{b}, \boldsymbol{c})=\cos \left(\phi_1+\phi_2+\phi_3\right), $ | (5) |
In the xy-plane polar angle θ would be
For measurement set shown in Fig. 2, ϕ1=0, and
| $ E_{\mathrm{QM}_{\mathrm{GHZ}}}^{\mathrm{ABC}}=\cos \left(2\left(\frac{\varphi}{2}\right)\right), $ | (6) |
| $ E_{\mathrm{QM}_{\mathrm{GHZ}}}^{\mathrm{AB}^{\prime} \mathrm{C}^{\prime}}=\cos \left(\frac{\pi}{2}+\frac{\varphi}{2}\right). $ | (7) |
After using different values of the angle in (1), (6) and (7), compatibility between QM and nonlocal realism can be checked as shown in Fig. 3(a).

|
Download:
|
|
(a) Shows the violations of the QM values for GHZ state when the measurements are restricted in the xy-plane and the angle is the azimuthal angle (φ). Whereas (b) presentsthe results for W state for respective measurement set while restricting the inputs in the xz-plane and the polar angle ( Fig. 3 Violations of the two inequalities in the full range of the angle |
|
And for a W state
| $ \begin{gathered} E_W(\boldsymbol{a}, \boldsymbol{b}, \boldsymbol{c})=-\frac{2}{3} \cos \left(\theta_1+\theta_2+\theta_3\right)- \\ \frac{1}{3} \cos \theta_1 \cos \theta_2 \cos \theta_3 . \end{gathered} $ | (8) |
In the xy-plane, the predicted quantum expectation value vanishes for any choice of azimuthal a ngles[19].
Now all measurements are in the xz-plane and violations are checked according to polar angle θ. For first measurement set, θ1=0, θ2=θ3=θ′2=θ′3=
| $ \begin{gathered} L_{q m W}=\mid 2 \cos \left(\frac{\vartheta}{2}\right)+\cos \left(\frac{\pi}{2}-\frac{\vartheta}{2}\right)-\cos \left(\frac{\pi}{2}+\frac{\vartheta}{2}\right)+ \\ \frac{2}{3} \cos \left(\frac{2 \vartheta}{2}\right)+\frac{1}{3} \cos ^2\left(\frac{\vartheta}{2}\right)+\frac{2}{3} \cos \left(\frac{\pi}{2}-\frac{2 \vartheta}{2}\right)+ \end{gathered} \\ \frac{1}{3} \cos \left(\frac{\vartheta}{2} \cos \left(\frac{\pi}{2}+\frac{\vartheta}{2}\right)\right) \mid-2, $ | (9) |
using these values compatibility could be checked as shown in Fig. 3(b).
4.3 For an arbitrary three-qubit pure stateFive-parameter family of pure states of three qubits with equivalence upto local unitary transformations is reprsented as[20-21],
For μ1=μ2=μ3=0, it is equivalent to GHZ state, so the results are applicable to this state as well.
5 ConclusionsIn this work, in pursuit of understanding nonlocality in multipartite case specifically Leggett-type nonlocality which is still unknown, we developed inequalities for three parties and check do quantum mechanical values violate these inequalities which are built on the assumption of nonlocal realism as advocated by Leggett. Violations are found which is in favor of the claim that nonlocality is a phenomenon, i.e., a fact of observation[22]. These inequalities are compared with the inequality in the literature, our inequalities are certainly stronger and confirm more violations of three tripartite states, i.e., GHZ, W, and an arbitrary three-qubit pure states.
Whether it is a weak or genuine tripartite Leggett-type nonlocality remains an open question? However, it could be one of the first attempt towards certain kind of nonlocalities in multiparty case and towards a better understanding of nonlocality in thiscase and overall. As nonlocality is gaining focus with increasing frequency with the maturity of quantum information science, we believe our results would get influential with time. We have tried to write the paper as easy as possible and simplified mathematics because our focus is more on the conceptual grab of the nonlocality. As in quantum steering one side is measurement dependent, so we believe Leggett model has yet to contribute a lot in quantum information science generally and in quantum steeringspecifically, considering its dependence upon the measurements. In that regard, we believe this work would get prominence on the canvas.
NoteDetailed derivations of the inequalities appeared in this work will be presented elsewhere.
We miss our collaborator LI Junli very much for his various discussions on related topics.
| [1] |
Bell J S. On the Einstein-Podolsky-Rosen paradox[J]. Physics Physique Fizika, 1964, 1(3): 195-200. DOI:10.1103/PhysicsPhysiqueFizika.1.195 |
| [2] |
Clauser J F, Horne M A, Shimony A, et al. Proposed experiment to test local hidden-variable theories[J]. Physical Review Letters, 1969, 23(15): 880-884. DOI:10.1103/PhysrevLett.23.880 |
| [3] |
Clauser J F, Horne M A. Experimental consequences of objective local theories[J]. Physical Review D, 1974, 10(2): 526-535. DOI:10.1103/Physrevd.10.526 |
| [4] |
Aspect A. Bell's theorem: the naive view of an experimentalist[M]//Bertlmann R A, Zeilinger A. Quantum[Un] speakables: from Bell to quantum information. Berlin Heidelberg: Springer-Verlag, 2002. DOI: 10.1007/978-3-662-05032-3_9.
|
| [5] |
Rowe M A, Kielpinski D, Meyer V, et al. Experimental violation of a Bell's inequality with efficient detection[J]. Nature, 2001, 409(6822): 791-794. DOI:10.1038/35057215 |
| [6] |
Brunner N, Cavalcanti D, Pironio S, et al. Bell nonlocality[J]. Reviews of Modern Physics, 2014, 86(2): 419-478. DOI:10.1103/RevModPhys.86.839 |
| [7] |
Qian C, Li J L, Khan A S, et al. Nonlocal correlation of spin in high energy physics[J]. Physical Review D, 2020, 101(11): 116004. DOI:10.1103/Physrevd.101.116004 |
| [8] |
Leggett A J. Nonlocal hidden-variable theories and quantum mechanics: an incompatible theorem[J]. Foundations of Physics, 2003, 33(10): 1469-1493. DOI:10.1023/A:1026096313729 |
| [9] |
Gröeblacher S, Paterek T, Kaltenbaek R, et al. An experimental test of non-local realism[J]. Nature, 2007, 446(7138): 871-875. DOI:10.1038/nature05677 |
| [10] |
Paterek T, Fedrizzi A, Gröblacher S, et al. Experimental test of nonlocal realistic theories without the rotational symmetry assumption[J]. Physical Review Letters, 2007, 99(21): 210406. DOI:10.1103/PhysRevLett.99.210406 |
| [11] |
Branciard C, Ling A, Gisin N, et al. Experimental falsification of Leggett's nonlocal variable model[J]. Physical Review Letters, 2007, 99(21): 210407. DOI:10.1103/PhysrevLett.99.210407 |
| [12] |
Branciard C, Brunner N, Gisin N, et al. Testing quantum correlations versus single-particle properties within Leggett's model and beyond[J]. Nature Physics, 2008, 4(9): 681-685. DOI:10.1038/nphys1020 |
| [13] |
Hasegawa Y, Schmitzer C, Bartosik H, et al. Falsification of Leggett's model using neutron matter waves[J]. New Journal of Physics, 2012, 14(2): 023039. DOI:10.1088/1367-2630/14/2/023039 |
| [14] |
Huang X, Ouyang X, Lian W, et al. Experimental test of Leggett's inequalities with solid-state spins[J]. Physical Review A, 2020, 102(1): 012210. DOI:10.1103/PhysRevA.102.012210 |
| [15] |
Khan A S, Li J L, Qiao C F. Test of nonlocal hidden variable theory by the Leggett inequality in high energy physics[J]. Physical Review D, 2020, 101(9): 096016. DOI:10.1103/Physrevd.101.096016 |
| [16] |
Svetlichny G. Distinguishing three-body from two-body nonseparability by a Bell-type inequality[J]. Physical Review D, 1987, 35(10): 3066. DOI:10.1103/physrevd.35.3066 |
| [17] |
Bancal J D, Branciard C, Gisin N. Simulation of equatorial von Neumann measurements on GHZ states using nonlocal resources[J]. Advances in Mathematical Physics, 2010, 2010(293245): 1-14. DOI:10.1155/2010/293245 |
| [18] |
Deng D L, Wu C, Chen J L, et al. Multipartite Leggett-type inequalities[EB/OL]. arXiv: 1111.4119v1. (2011-11-07)[2021-06-01]. https://arxiv.org/abs/1111.4119.
|
| [19] |
Cereceda J L. Three-particle entanglement versus three-particle nonlocality[J]. Physical Review A, 2002, 66(2): 024102. DOI:10.1103/physreva.66.024102 |
| [20] |
Acín A, Andrianov A, Jané E, et al. Three-qubit pure-state canonical forms[J]. Journal of Physics A: Mathematical and General, 2001, 34(35): 6725. DOI:10.1088/0305-4470/34/35/301 |
| [21] |
Emary C, Beenakker C W J. Relation between entanglement measures and Bell inequalities for three qubits[J]. Physical Review A, 2004, 69(3): 032317. DOI:10.1103/physreva.69.032317 |
| [22] |
Scarani V. Bell nonlocality[M]. Oxford: Oxford University Press, 2019.
|
 2023, Vol. 40
2023, Vol. 40 


