The theory of minimal surfaces is an important part of modern differential geometry. The theory is particularly fruitful when the ambient space is a symmetric space. Calabi^[1] proved a rigidity theorem for minimal two-spheres of constant curvature in Sⁿ. Bolton et al.^[2] constructed all the minimal two-spheres of constant curvature in $\mathbb{C}$Pⁿ, and showed that a totally real minimal two-sphere in $\mathbb{C}$Pⁿ can be mapped, by a holomorphic isometry of $\mathbb{C}$Pⁿ, into $\mathbb{R}$Pⁿ⊂$\mathbb{C}$Pⁿ. Then He and Wang^[3] proved a similar rigidity result for totally real minimal two-spheres in $\mathbb{H}$Pⁿ.

In this paper, we present a construction of the complex Grassmannian G(2, n+2) due to Berndt^[4], which considers G(2, n+2) as a quotient of some minimal submanifold Qⁿ⁺¹ of $\mathbb{H}$Pⁿ⁺¹. A Riemannian metric can be given on G(2, n+2) so that the projection π: Qⁿ⁺¹→G(2, n+2) is a Riemannian submersion. Then we show that a surface in G(2, n+2) can be horizontally lifted to Qⁿ⁺¹ if and only if it is totally real.

Our result is a special case of Ref.[5], where the author considered a general Riemannian submersion N→B, and characterized the existence of horizontal lifts of a submanifold of B using a family J of (1, 1)-tensors on B. In our paper, we make use of the fact that the projection Qⁿ⁺¹→G(2, n+2) is a principal bundle, thus obtain a characterization by a first order PDE. Our method is largely inspired by Ref.[3], where the authors considered the Riemannian submersion S⁴ⁿ⁺³→$\mathbb{H}$Pⁿ.

1 Preliminaries

We denote by $\mathbb{H}$ the algebra of quaternions. This is a 4-dimensional vector space over $\mathbb{R}$ with basis {1, i, j, k}, and the multiplication is defined by

$ \mathrm{i}^{2}=\mathrm{j}^{2}=\mathrm{k}^{2}=-1, $

$ \mathrm{ij}=\mathrm{k}=-\mathrm{ji}, \mathrm{jk}=\mathrm{i}=-\mathrm{kj}, \mathrm{ki}=\mathrm{j}=-\mathrm{ik} . $

Thus $\mathbb{H}$ is associative but not commutative. $\mathbb{R}$ and $\mathbb{C}$ are naturally embedded into $\mathbb{H}$ as follows:

$ \mathbb{R}=\mathbb{R} \cdot 1 \subset \mathbb{H}, \quad \mathbb{C}=\mathbb{R} \cdot 1 \oplus \mathbb{R} \cdot \mathrm{i} \subset \mathbb{H}, $

and we sometimes express an element of $\mathbb{H}$ as q=z+wj, where z, w∈$\mathbb{C}$.

Conjugation is defined for quaternions:

$ \overline{a+b \mathrm{i}+c \mathrm{j}+d \mathrm{k}}=a-b \mathrm{i}-c \mathrm{j}-d \mathrm{k}(a, b, c, d \in \mathbb{R}). $

Or equivalently,

$ \overline{z+w \mathrm{j}}=\bar{z}-w \mathrm{j}(z, w \in \mathbb{C}). $

Then we have pq=qp, for any p, q∈$\mathbb{H}$.

Let $\mathbb{H}$ⁿ be the space of n-dimensional quaternion column vectors. We consider it as a right $\mathbb{H}$-module. If p=(p₁, …, p_n)^T, q=(q₁, …, q_n)^T∈$\mathbb{H}$ⁿ, two inner products of p, q are defined:

$ \langle\boldsymbol{p}, \boldsymbol{q}\rangle_{\mathbb{H}}=\sum\limits_{l} \bar{p}_{l} q_{l},\langle\boldsymbol{p}, \boldsymbol{q}\rangle_{\mathbb{R}}=\operatorname{Re}\langle\boldsymbol{p}, \boldsymbol{q}\rangle_{\mathbb{H}}. $

It is easily verified that 〈, 〉_$\mathbb{R}$ is just the usual Euclidean inner product if $\mathbb{H}$ⁿ is identified as $\mathbb{R}$⁴ⁿ, and that the following properties hold:

$ \langle\boldsymbol{p} x, \boldsymbol{q} y\rangle_{\mathbb{H}} =\bar{x}\langle\boldsymbol{p}, \boldsymbol{q}\rangle_{\mathbb{H}} y, $

$ \langle\boldsymbol{p}, \boldsymbol{q}\rangle_{\mathbb{H}} =\overline{\langle\boldsymbol{q}, \boldsymbol{p}\rangle}_{\mathbb{H}}, $

where p, q∈$\mathbb{H}$ⁿ, x, y∈$\mathbb{H}$.

Similarly, for z=(z₁, …, z_n)^t, w=(w₁, …, w_n)^t∈$\mathbb{C}$ⁿ, we define their inner products:

$ \langle \boldsymbol{z}, \boldsymbol{w}\rangle_{\mathbb{C}}=\sum\limits_{1} \overline{\mathrm{z}}_{1} \mathrm{w}_{1},\langle \boldsymbol{z}, \boldsymbol{w}\rangle_{\mathbb{R}}=\operatorname{Re}\langle \boldsymbol{z}, \boldsymbol{w}\rangle_{\mathbb{C}}. $

We will often omit the subscripts $\mathbb{C}$and $\mathbb{H}$ for simplicity.

Next we consider the quaternion projective space $\mathbb{H}$Pⁿ, the set of quaternionic lines in $\mathbb{H}$ⁿ⁺¹. Equivalently, $\mathbb{H}$Pⁿ=S⁴ⁿ⁺³/Sp(1), where S⁴ⁿ⁺³ is the unit sphere in $\mathbb{H}$ⁿ⁺¹$\cong \mathbb{R}$⁴ⁿ⁺⁴, and Sp(1), the multiplicative group of unit quaternions, acts on S⁴ⁿ⁺³ by right multiplication. Since this is an isometric action, there is a unique Riemannian metric on $\mathbb{H}$Pⁿ, called the Fubini-Study metric, such that the quotient map τ: S⁴ⁿ⁺³→$\mathbb{H}$Pⁿ is a Riemannian submersion. For any q∈S⁴ⁿ⁺³, let H_q be the horizontal space of τ at q, i.e. the normal space to the fibre τ^-1(τ(q)). Then H_q={q′∈$\mathbb{H}$ⁿ⁺¹|〈q, q′〉_$\mathbb{H}$=0}. Let τ_q=dτ_q|_Hq. By assumption, τ_q: H_q→T_τ(q)$\mathbb{H}$Pⁿ is a linear isometry.

2 The submanifold Qⁿ⁺¹⊂$\mathbb{H}$Pⁿ⁺¹; the complex Grassmannian G(2, n+2)

We quote some results from Ref.[4].

SU(n+2) acts on S⁴ⁿ⁺⁷⊂$\mathbb{H}$ⁿ⁺² isometrically via

$ S U(n+2) \times S^{4 n+7} \rightarrow S^{4 n+7}, $

$ (\boldsymbol{A}, \boldsymbol{z}+\boldsymbol{v} \mathrm{j}) \mapsto \boldsymbol{A} \boldsymbol{z}+(\boldsymbol{A} \boldsymbol{v}) \mathrm{j}, $

where z, v∈$\mathbb{C}$ⁿ⁺², with |z|²+|v|²=1. This action commutes with the Sp(1)-action on S⁴ⁿ⁺⁷ defined in the last section, hence descends to an isometric action on $\mathbb{H}$Pⁿ⁺¹.

By some straightforward calculations, we find that this SU(n+2)-action on $\mathbb{H}$Pⁿ⁺¹ has only two singular orbits, namely,

$ \mathbb{C} P^{n+1}=\left\{\boldsymbol{\tau}(\boldsymbol{z}+0 \cdot \mathrm{j}) \mid \boldsymbol{z} \in S^{2 n+3}\right\}, $

(1)

and

$ \begin{aligned} Q^{n+1} &=\{\boldsymbol{\tau}((1 / \sqrt{2})(\boldsymbol{z}+\boldsymbol{v} \mathrm{j})) \mid \boldsymbol{z},\\ \boldsymbol{v} &\left.\in S^{2 n+3},\langle \boldsymbol{z}, \boldsymbol{v}\rangle=0\right\}, \end{aligned} $

(2)

where S²ⁿ⁺³ is the unit sphere of $\mathbb{C}$ⁿ⁺².

We have the following proposition from Ref.[4]:

Proposition 2.1  The singular orbits of the SU(n+2)-action on $\mathbb{H}$Pⁿ⁺¹ are $\mathbb{C}$Pⁿ⁺¹ and Qⁿ⁺¹.Qⁿ⁺¹ has codimension 3 in $\mathbb{H}$Pⁿ⁺¹, and is isometric to the homogeneous space SU(n+2)/SU(2)×SU(n) equipped with a suitable invariant metric. Furthermore, Qⁿ⁺¹ is a minimal submanifold of $\mathbb{H}$Pⁿ⁺¹.

Now consider an action of U(1) on Qⁿ⁺¹:

$ U(1) \times Q^{n+1} \rightarrow Q^{n+1},\left(\mathrm{e}^{\mathrm{i} t}, \boldsymbol{\tau}(\boldsymbol{q})\right) \mapsto \boldsymbol{\tau}\left(\mathrm{e}^{\mathrm{i} t} \boldsymbol{q}\right), $

where t∈$\mathbb{R}$. Again this is an isometric action. A vector field ξ on Qⁿ⁺¹ is defined:

$ \begin{aligned} \boldsymbol{\xi}_{\boldsymbol{\tau}(\boldsymbol{q})} &=\left.\frac{\mathrm{d}}{\mathrm{d} t}\right|_{t=0} \mathrm{e}^{\mathrm{i} t} \cdot \boldsymbol{\tau}(\boldsymbol{q})=\left.\frac{\mathrm{d}}{\mathrm{d} t}\right|_{t=0} \boldsymbol{\tau}\left(\mathrm{e}^{\mathrm{i} t} \boldsymbol{q}\right) \\ &=\mathrm{d} \boldsymbol{\tau}_{\boldsymbol{q}}(\mathrm{i} \boldsymbol{q})=\boldsymbol{\tau}_{\boldsymbol{q}}(\mathrm{i} \boldsymbol{q}). \end{aligned} $

(3)

Here $\boldsymbol{q}=\frac{1}{\sqrt{2}}(\boldsymbol{z}+\boldsymbol{v} \mathrm{j}) \in \boldsymbol{\tau}^{-1}\left(Q^{n+1}\right), \boldsymbol{z}, \boldsymbol{v} \in S^{2 n+3}$, 〈z, v〉=0. For the last equality, we note that 〈q, iq〉_$\mathbb{H}$=0 for q∈τ^-1(Qⁿ⁺¹), i.e., iq∈H_q. Thus ξ is the field of tangent vectors to the orbits of the U(1)-action.

Let Bⁿ⁺¹=Qⁿ⁺¹/U(1). Since U(1) acts on Qⁿ⁺¹ isometrically, there is a unique Riemannian metric on Bⁿ⁺¹ such that the natural projection π: Qⁿ⁺¹→Bⁿ⁺¹ is a Riemannian submersion.

For τ(q)∈Qⁿ⁺¹, let $\mathcal{H}$_τ(q) be the orthogonal complement of ξ_τ(q) in T_τ(q)Qⁿ⁺¹, i.e. the horizontal space of the Riemannian submersion π: Qⁿ⁺¹→Bⁿ⁺¹. Then the map $\pi_{\tau(\boldsymbol{q})}=\mathrm{d} \pi_{\tau(\boldsymbol{q})} \mid _{\mathcal{H}_{\tau(\boldsymbol{q})}}\;: \mathcal{H}_{\tau(\boldsymbol{q})}$→T_π(τ(q))Bⁿ⁺¹ is a linear isometry. By Ref.[4], the horizontal lift of $\mathcal{H}_{\tau(\boldsymbol{q})}$ through τ: S⁴ⁿ⁺⁷→$\mathbb{H}$Pⁿ⁺¹ is

$ \boldsymbol{\tau}_{\boldsymbol{q}}^{-1} \mathcal{H}_{\boldsymbol{\tau}(\boldsymbol{q})}=\left\{\boldsymbol{X} \in \mathbb{H}^{n+2} \mid\langle\boldsymbol{X}, \boldsymbol{q}\rangle=\langle\boldsymbol{X}, \mathrm{i} \boldsymbol{q}\rangle=0\right\}. $

(4)

We define a (1, 1)-tensor φ on Qⁿ⁺¹ as φX=$-\nabla_{\boldsymbol{X}}^{Q^{n+1}} \boldsymbol{\xi}, \boldsymbol{X} \in T Q^{n+1}$, where $\nabla^{Q^{n+1}}$ is the Riemannian connection on Qⁿ⁺¹. Using the O'Neil formula for Riemannian submersions (see, for example, Proposition 4.5.1 of Ref.[6]), it can be shown that

$ \varphi X= \begin{cases}0, & \boldsymbol{X}=\boldsymbol{\xi}, \\ \boldsymbol{\tau}_{\boldsymbol{q}}\left(-\mathrm{i} \cdot \boldsymbol{\tau}_{\boldsymbol{q}}^{-1}(\boldsymbol{X})\right), & \boldsymbol{X} \in \mathcal{H}_{\boldsymbol{\tau}(\boldsymbol{q})} .\end{cases} $

(5)

Since by definition T_τ(q)Qⁿ⁺¹=$\mathbb{R}$·$\boldsymbol{\xi}_{\tau(\boldsymbol{q})} \oplus \mathcal{H}_{\tau(\boldsymbol{q})}$, this completely determines φ. In particular, $\varphi(\mathcal{H})\subset \mathcal{H}$.

Finally, notice that φ commutes with the U(1)-action on Qⁿ⁺¹. In other words, if L_t denotes the map Qⁿ⁺¹→Qⁿ⁺¹, $\tau(\boldsymbol{q}) \mapsto \tau\left(\mathrm{e}^{\mathrm{i} t} \boldsymbol{q}\right)$, then $\mathrm{d} L_{t} \circ \varphi=\varphi \circ \mathrm{d} L_{t}$ for all t∈$\mathbb{R}$. Therefore, there exists a (1, 1)-tensor J on Bⁿ⁺¹ satisfying Jπ_*=π_*φ. As φ²X=-X for all $\boldsymbol{X} \in \mathcal{H}$, it follows that J is an almost Hermitian structure on Bⁿ⁺¹. Actually, as is proved in Ref.[4], J turns out to be Kähler, and Bⁿ⁺¹ is holomorphically isometric to the complex Grass-mannian

$ G(2, n+2)=U(n+2) / U(2) \times U(n), $

where the metric on G(2, n+2) is induced by the following bi-invariant metric on U(n+2):

$ \langle\boldsymbol{X}, \boldsymbol{Y}\rangle=-\frac{1}{4} \operatorname{tr}(\boldsymbol{X} \boldsymbol{Y}),(\boldsymbol{X}, \boldsymbol{Y} \in \mathfrak{U}(n+2)). $

Thus, for example, B² is isometric to G(2, 3)=$\mathbb{C}$P², with the Fubini-Study metric of constant holomorphic sectional curvature 8.

Remark  The isometry between G(2, n+2) and Bⁿ⁺¹ can be explicitly given as

$ G(2, n+2) \rightarrow B^{n+1}, $

$ \mathbb{C} \boldsymbol{z} \oplus \mathbb{C} \boldsymbol{v} \mapsto \boldsymbol{\pi}\left(\boldsymbol{\tau}\left(\frac{1}{\sqrt{2}}(\boldsymbol{z}+\boldsymbol{v} \mathrm{j})\right)\right), $

where z, v∈$\mathbb{C}$ⁿ⁺², |z|=|v|=1, 〈z, v〉_$\mathbb{C}$=0.

3 The main theorem

Definition 3.1  Suppose N is a Hermitian manifold, J is its complex structure, f: M→N is an immersion from a surface M to N. Then f is called totally real if J Im f_*p⊥Imf_*p for all p∈M.

If we choose a local frame X, Y for M, then fis totally real if and only if Jf_*X⊥f_*Y everywhere. This follows easily from the Hermitian condition 〈Ju, Jv〉=〈u, v〉, J²=-1, where 〈, 〉 is the Riemannian metric on N.

Now we can state our main result.

Theorem 3.1  Suppose M is a surface, ψ: M→Bⁿ⁺¹ an immersion, then the following are equivalent:

1) ψ is totally real;

2) ψ has local horizontal lifts to Qⁿ⁺¹, that is, for any p∈M, there is a neighborhood U of p, and an immersion η: U→Qⁿ⁺¹, such that $\pi \circ \eta=\psi$, and Im $\eta_{*} \subset \mathcal{H}$.

Furthermore, η is minimal in Qⁿ⁺¹ if and only if ψ is minimal in Bⁿ⁺¹.

We prove the theorem step by step.

Step 1  Let U be an open subset of M, η: U→Qⁿ⁺¹ an immersion, we shall find a sufficient and necessary condition for η to be horizontal.

First, since τ: S⁴ⁿ⁺⁷→$\mathbb{H}$Pⁿ⁺¹ is a submersion, η can be lifted to S⁴ⁿ⁺⁷, that is, there is an immersion $\boldsymbol{q}=\frac{1}{\sqrt{2}}(\boldsymbol{Z}+\boldsymbol{V} \mathrm{j}): U \rightarrow \tau^{-1}\left(Q^{n+1}\right) \subset S^{4 n+7}$ such that $\eta=\tau \circ q$, where Z, V: U→$\mathbb{C}$ⁿ⁺², |Z|=|V|=1, 〈Z, V〉=0. Now

$ \begin{aligned} \mathrm{d} \eta &=\mathrm{d} \boldsymbol{\tau} \mathrm{d} \boldsymbol{q} \\ &=\mathrm{d} \boldsymbol{\tau}(\mathrm{d} \boldsymbol{q}-\boldsymbol{q}\langle\boldsymbol{q}, \mathrm{d} \boldsymbol{q}\rangle), \end{aligned} $

(6)

so the horizontal lift of dη to S⁴ⁿ⁺⁷ is τ_q^-1dη=dq-q〈q, dq〉, namely the orthogonal projection of dq onto H_q, the horizontal space of τ at q.

Recall from the last section that

η is horizontal with respect to π

$\Leftrightarrow \operatorname{Im}(\mathrm{d} \eta) \subset \mathcal{H}$

$\Leftrightarrow$〈τ_q^-1dη, q〉=〈τ_q^-1dη, iq〉=0

$\Leftrightarrow$〈dq-q〈q, dq〉, iq〉=0

$\Leftrightarrow$〈dq, iq〉=0.

For the last equivalence note that q∈τ^-1(Qⁿ⁺¹) implies 〈q, iq〉=0.

Write $\boldsymbol{q}=\frac{1}{\sqrt{2}}(\boldsymbol{Z}+\boldsymbol{V} \mathrm{j}), \mathrm{d} \boldsymbol{q}=\frac{1}{\sqrt{2}}(\mathrm{~d} \boldsymbol{Z}+\mathrm{d} \boldsymbol{V} \cdot \mathrm{j})$. Differentiating 〈V, V〉=1, 〈Z, V〉=0 gives

$ \left\{\begin{array}{l} \langle\mathrm{d} \boldsymbol{V}, \boldsymbol{V}\rangle+\langle\boldsymbol{V}, \mathrm{d} \boldsymbol{V}\rangle=0, \\ \langle\mathrm{d} \boldsymbol{Z}, \boldsymbol{V}\rangle+\langle\boldsymbol{Z}, \mathrm{d} \boldsymbol{V}\rangle=0. \end{array}\right. $

Then

$ \begin{aligned} \langle&\mathrm{d} \boldsymbol{q}, \mathrm{i} \boldsymbol{q}\rangle=0 \\ \Leftrightarrow 0=&\langle\mathrm{d} \boldsymbol{Z}+\mathrm{d} \boldsymbol{V} \cdot \mathrm{j}, \boldsymbol{Z} \mathrm{i}+\boldsymbol{V} \boldsymbol{\mathrm { k }}\rangle \\ =&(\langle\mathrm{d} \boldsymbol{Z}, \boldsymbol{Z}\rangle-\langle\boldsymbol{V}, \mathrm{d} \boldsymbol{V}\rangle) \mathrm{i}+\\ &(\langle\boldsymbol{Z}, \mathrm{d} \boldsymbol{V}\rangle+\langle\mathrm{d} \boldsymbol{Z}, \boldsymbol{V}\rangle) \mathrm{k} \\ =&(\langle\mathrm{d} \boldsymbol{Z}, \boldsymbol{Z}\rangle+\langle\mathrm{d} \boldsymbol{V}, \boldsymbol{V}\rangle) \mathrm{i} . \end{aligned} $

In summary, we have proved

Lemma 3.1  Suppose $\eta=\tau\left(\frac{1}{\sqrt{2}}(\boldsymbol{Z}+\boldsymbol{V} \mathrm{j})\right)$ : U→Qⁿ⁺¹ is an immersion. Then η is horizontal with respect to π: Qⁿ⁺¹→Bⁿ⁺¹ if and only if

$ \langle\mathrm{d} \boldsymbol{Z}, \boldsymbol{Z}\rangle+\langle\mathrm{d} \boldsymbol{V}, \boldsymbol{V}\rangle=0. $

(7)

Step 2  Let ψ: M→Bⁿ⁺¹ be an immersion of a surface M into Bⁿ⁺¹. We look for the condition under which ψ has a local horizontal lift to Qⁿ⁺¹.

Let $\eta=\tau \circ \boldsymbol{q}=\tau\left(\frac{1}{\sqrt{2}}(\boldsymbol{Z}+\boldsymbol{V} \mathrm{j})\right): U \rightarrow Q^{n+1}$ be any local lift of ψ, and η₀ a horizontal lift of ψ. Recall that Bⁿ⁺¹ is defined as the quotient of Qⁿ⁺¹ under the U(1)-action e^it·τ(q)=τ(e^itq), then for any p∈U, η(p) and η₀(p) lie in the same orbit. It follows that there is a map λ: U→U(1) such that η₀(p)=λ(p)·η(p) for all p∈U. In short,

$ \eta_{0}=\lambda \cdot \eta=\boldsymbol{\tau}\left(\frac{1}{\sqrt{2}}(\lambda \boldsymbol{Z}+\lambda \boldsymbol{V} \mathrm{j})\right). $

(8)

Since η₀ is horizontal, we apply Lemma 1 to obtain

$ \begin{aligned} 0 &=\langle\mathrm{d}(\lambda \boldsymbol{Z}), \lambda \boldsymbol{Z}\rangle+\langle\mathrm{d}(\lambda \boldsymbol{V}), \lambda \boldsymbol{V}\rangle \\ &=\langle\mathrm{d} \lambda \cdot \boldsymbol{Z}+\lambda \mathrm{d} \boldsymbol{Z}, \lambda \boldsymbol{Z}\rangle+\langle\mathrm{d} \lambda \cdot \boldsymbol{V}+\lambda \mathrm{d} \boldsymbol{V}, \lambda \boldsymbol{V}\rangle \\ &=\lambda \mathrm{d} \bar{\lambda}(\langle\boldsymbol{Z}, \boldsymbol{Z}\rangle+\langle\boldsymbol{V}, \boldsymbol{V}\rangle)+\lambda \bar{\lambda}(\langle\mathrm{d} \boldsymbol{Z}, \boldsymbol{Z}\rangle+\langle\mathrm{d} \boldsymbol{V}, \boldsymbol{V}\rangle) \\ &=-2 \bar{\lambda} \mathrm{d} \lambda+\langle\mathrm{d} \boldsymbol{Z}, \boldsymbol{Z}\rangle+\langle\mathrm{d} \boldsymbol{V}, \boldsymbol{V}\rangle. \end{aligned} $

Here we have used λλ=1 and λdλ+λdλ=0. Since λdλ=λ^-1dλ=d(logλ) we get

$ 2 \mathrm{~d}(\log \lambda)=\langle\mathrm{d} \boldsymbol{Z}, \boldsymbol{Z}\rangle+\langle\mathrm{d} \boldsymbol{V}, \boldsymbol{V}\rangle. $

(9)

If we take a local coordinate (x, y) on M, this amounts to

$ \left\{\begin{array}{l} 2 \frac{\partial \log {\lambda}}{\partial x}=\left\langle\boldsymbol{Z}_{x}, \boldsymbol{Z}\right\rangle+\left\langle\boldsymbol{V}_{x}, \boldsymbol{V}\right\rangle, \\ 2 \frac{\partial \log \lambda}{\partial y}=\left\langle\boldsymbol{Z}_{y}, \boldsymbol{Z}\right\rangle+\left\langle\boldsymbol{V}_{y}, \boldsymbol{V}\right\rangle, \end{array}\right. $

(10)

where $\boldsymbol{Z}_{x}=\frac{\partial \boldsymbol{Z}}{\partial x}, \boldsymbol{Z}_{y}=\frac{\partial \boldsymbol{Z}}{\partial y}$, etc. This is a system of first-order PDEs inλ. By the Frobenius theorem for PDEs, an initial value problem of such a system is solvable if and only if the integrability condition

$ \frac{\partial}{\partial y}\left(\frac{\partial \log \lambda}{\partial x}\right)=\frac{\partial}{\partial x}\left(\frac{\partial \log \lambda}{\partial y}\right), $

that is,

$ \frac{\partial}{\partial y}\left(\left\langle\boldsymbol{Z}_{x}, \boldsymbol{Z}\right\rangle+\left\langle\boldsymbol{V}_{x}, \boldsymbol{V}\right\rangle\right)=\frac{\partial}{\partial x}\left(\left\langle\boldsymbol{Z}_{y}, \boldsymbol{Z}\right\rangle+\left\langle\boldsymbol{V}_{y}, \boldsymbol{V}\right\rangle\right) $

holds. This equation simplifies to

$ \left\langle\boldsymbol{Z}_{x}, \boldsymbol{Z}_{y}\right\rangle+\left\langle\boldsymbol{V}_{x}, \boldsymbol{V}_{y}\right\rangle=\left\langle\boldsymbol{Z}_{y}, \boldsymbol{Z}_{x}\right\rangle+\left\langle\boldsymbol{V}_{y}, \boldsymbol{V}_{x}\right\rangle . $

(11)

Thus we obtain

Lemma 3.2  Suppose $ \psi=\pi \circ \tau\left(\frac{1}{\sqrt{2}}(\boldsymbol{Z}+\right.\boldsymbol{V}\text{ j) ) }$ : M→Bⁿ⁺¹ is an immersion. Then ψ has local horizontal lifts to Qⁿ⁺¹ if and only if (11) holds.

Step 3  Let $\psi=\pi \circ \tau \circ q: M \rightarrow B^{n+1}$, where q=$\frac{1}{\sqrt{2}}(\boldsymbol{Z}+\boldsymbol{V} \mathrm{j}): M \rightarrow \tau^{-1}\left(Q^{n+1}\right) \subset S^{4 n+7}$. We shall find out the equation for ψ to be totally real.

We have

$ \begin{aligned} \mathrm{d} \psi &=\mathrm{d} \pi \mathrm{d} \boldsymbol{\tau} \mathrm{d} \boldsymbol{q} \\ &=\mathrm{d} \pi \mathrm{d} \boldsymbol{\tau}(\mathrm{d} \boldsymbol{q}-\boldsymbol{q}\langle\boldsymbol{q}, \mathrm{d} \boldsymbol{q}\rangle-\mathrm{i} \boldsymbol{q}\langle\mathrm{i} \boldsymbol{q}, \mathrm{d} \boldsymbol{q}\rangle) \\ &=\mathrm{d} \pi \mathrm{d} \boldsymbol{\tau}\left(\mathrm{d} \boldsymbol{q}^{\mathcal{H}}\right), \end{aligned} $

(12)

where $\mathrm{d} \boldsymbol{q}^{\mathcal{H}}=\mathrm{d} \boldsymbol{q}-\boldsymbol{q}\langle\boldsymbol{q}, \mathrm{d} \boldsymbol{q}\rangle-\mathrm{i} \boldsymbol{q}\langle\mathrm{i} \boldsymbol{q}, \mathrm{d} \boldsymbol{q}\rangle$ is the orthogonal projection of dq onto $\tau_{\boldsymbol{q}}^{-1} \mathcal{H}_{\tau(\boldsymbol{q})}$. In other words, $\mathrm{d} \boldsymbol{q}^{\mathcal{H}}=\tau_{\boldsymbol{q}}^{-1} {\pi}_{\tau(\boldsymbol{q})}^{-1}(\mathrm{~d} \psi)$.

Choose a local coordinate (x, y) on M. Then, using the definitions of the tensors φ, J (see (5)), and the fact that τ, π are Riemannian submersions, we obtain

ψ is totally real

$ \begin{aligned} \Leftrightarrow 0 &=\left\langle\psi_{x}, J \psi_{y}\right\rangle_{B^{n+1}} \\ &=\left\langle\pi_{\boldsymbol{\tau}(\boldsymbol{q})}^{-1} \psi_{x}, \pi_{\boldsymbol{\tau}(\boldsymbol{q})}^{-1} J \psi_{y}\right\rangle_{Q^{n+1}} \\ &=\left\langle{\pi}_{\boldsymbol{\tau}(\boldsymbol{q})}^{-1} \psi_{x}, {\varphi} \pi_{\boldsymbol{\tau}(\boldsymbol{q})}^{-1} \psi_{y}\right\rangle_{Q^{n+1}} \\ &=\left\langle\boldsymbol{\tau}_{\boldsymbol{q}}^{-1} \pi_{\boldsymbol{\tau}(\boldsymbol{q})}^{-1} \psi_{x}, \boldsymbol{\tau}_{\boldsymbol{q}}^{-1} \varphi \pi_{\boldsymbol{\tau}(\boldsymbol{q})}^{-1} \psi_{y}\right\rangle_{\mathbb{R}} \\ &=\left\langle\boldsymbol{\tau}_{\boldsymbol{q}}^{-1} \pi_{\boldsymbol{\tau}(\boldsymbol{q})}^{-1} \psi_{x},-\mathrm{i} \cdot \boldsymbol{\tau}_{\boldsymbol{q}}^{-1} \pi_{\boldsymbol{\tau}(\boldsymbol{q})}^{-1} \psi_{y}\right\rangle_{\mathbb{R}} \\ &=\left\langle\boldsymbol{q}_{x}^{\mathcal{H}},-\mathrm{i} \boldsymbol{q}_{y}^{\mathcal{H}}\right\rangle_{\mathbb{R}} . \end{aligned} $

(13)

Since $\left\langle\boldsymbol{q}_{x}^{\mathcal{H}}, \mathrm{i} \boldsymbol{q}_{y}^{\mathcal{H}}\right\rangle_{\mathbb{R}}=\operatorname{Re}\left\langle\boldsymbol{q}_{x}^{\mathcal{H}}, \mathrm{i} \boldsymbol{q}_{y}^{\mathcal{H}}\right\rangle$, let us calculate $\left\langle\boldsymbol{q}_{x}^{\mathcal{H}}, \mathrm{i} \boldsymbol{q}_{y}^{\mathcal{H}}\right\rangle$ first. Now

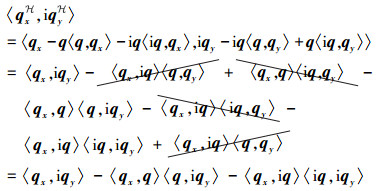
For the second step note that q∈τ^-1(Qⁿ⁺¹) implies 〈q, iq〉=0. Differentiating〈q, q〉=1 yields

$ \begin{aligned} 0 &=\left\langle\boldsymbol{q}_{x}, \boldsymbol{q}\right\rangle+\left\langle\boldsymbol{q}, \boldsymbol{q}_{x}\right\rangle \\ &=\left\langle\boldsymbol{q}_{x}, \boldsymbol{q}\right\rangle+\overline{\left\langle\boldsymbol{q}_{x}, \boldsymbol{q}\right\rangle}, \end{aligned} $

i.e., 〈q_x, q〉∈Im$\mathbb{H}$. Similarly, differentiating 〈q, iq〉=0 yields

$ \begin{aligned} 0 &=\left\langle\boldsymbol{q}_{y}, \mathrm{i} \boldsymbol{q}\right\rangle+\left\langle\boldsymbol{q}, \mathrm{i} \boldsymbol{q}_{y}\right\rangle \\ &=-\left\langle\mathrm{i} \boldsymbol{q}_{y}, \boldsymbol{q}\right\rangle+\left\langle\boldsymbol{q}, \mathrm{i} \boldsymbol{q}_{y}\right\rangle \\ &=-\overline{\left\langle\boldsymbol{q}, \mathrm{i} \boldsymbol{q}_{y}\right\rangle}+\left\langle\boldsymbol{q}, \mathrm{i} \boldsymbol{q}_{y}\right\rangle, \end{aligned} $

i.e., 〈q, iq_y〉∈$\mathbb{R}$. Therefore〈q_x, q〉〈q, iq_y〉∈Im$\mathbb{H}$. Similarly 〈q_x, iq〉〈iq, iq_y〉∈Im$\mathbb{H}$. Thus we get

$ \begin{aligned} & 2\left\langle\boldsymbol{q}_{x}^{\mathcal{H}}, \mathrm{i} \boldsymbol{q}_{y}^{\mathcal{H}}\right\rangle_{\mathbb{R}} \\ =& 2 \operatorname{Re}\left\langle\boldsymbol{q}_{x}^{\mathcal{H}}, \mathrm{i} \boldsymbol{q}_{y}^{\mathcal{H}}\right\rangle \\ =& 2 \operatorname{Re}\left\langle\boldsymbol{q}_{x}, \mathrm{i} \boldsymbol{q}_{y}\right\rangle \\ =& \operatorname{Re}\left\langle\boldsymbol{Z}_{x}+\boldsymbol{V}_{x} \mathrm{j}, \boldsymbol{Z}_{y} \mathrm{i}+\boldsymbol{V}_{y} \mathrm{k}\right\rangle \\ =& \operatorname{Re}\left(\left\langle\boldsymbol{Z}_{x}, \boldsymbol{Z}_{y}\right\rangle \mathrm{i}-\left\langle\boldsymbol{V}_{y}, \boldsymbol{V}_{x}\right\rangle \mathrm{i}\right) \\ =& \operatorname{Im}\left(\left\langle\boldsymbol{V}_{y}, \boldsymbol{V}_{x}\right\rangle-\left\langle\boldsymbol{Z}_{x}, \boldsymbol{Z}_{y}\right\rangle\right) . \end{aligned} $

(14)

Finally, from (13) and (14) we obtain

Lemma 3.3  $\psi=\pi \circ \tau\left(\frac{1}{\sqrt{2}}(\boldsymbol{Z}+\boldsymbol{V} \mathrm{j})\right): \boldsymbol{M}$→Bⁿ⁺¹ is totally real if and only if

$ \operatorname{Im}\left(\left\langle\boldsymbol{V}_{y}, \boldsymbol{V}_{x}\right\rangle-\left\langle\boldsymbol{Z}_{x}, \boldsymbol{Z}_{y}\right\rangle\right)=0, $

or equivalently,

$ \left\langle\boldsymbol{V}_{y}, \boldsymbol{V}_{x}\right\rangle-\left\langle\boldsymbol{Z}_{x}, \boldsymbol{Z}_{y}\right\rangle=\left\langle\boldsymbol{V}_{x}, \boldsymbol{V}_{y}\right\rangle-\left\langle\boldsymbol{Z}_{y}, \boldsymbol{Z}_{x}\right\rangle $

(15)

Comparing with Lemma 3.2, we find that ψ have a local horizontal lift to Qⁿ⁺¹ if and only if it is totally real.

Step 4  We need a simple lemma.

Lemma 3.4  Suppose $\pi: \bar{N} \rightarrow N$ is a Riemannian submersion, $\bar{M} \subset \bar{N}$ is a horizontal submanifold, and $M=\pi(\bar{M}) \subset N$. Then

$ \boldsymbol{H}_{M}(\pi(p))=\pi_{*}\left(\boldsymbol{H}_{\bar{M}}(p)\right) $

for any p∈M. Furthermore, H_M is horizontal. Here H_M, H_M are the mean curvature vectors of M, M, respectively.

Proof  Let e₁, …, e_m be an orthonormal frame on M, then, since $\left.\pi\right|_{\bar{M}}: \bar{M} \rightarrow M$ is an isometry, e₁=π_*(e₁), _…, e_m=π_*(e_m) is an orthonormal frame on M. By O'Neil's formula, $\nabla_{\bar{\boldsymbol{e}}_{i}}^{\bar{N}} \bar{\boldsymbol{e}}_{i}$ is the horizontal lift of $\nabla_{\boldsymbol{e}_{i}}^{N} \boldsymbol{e}_{i}$, hence horizontal, and $\boldsymbol{B}_{\bar{M}}\left(\overline{\boldsymbol{e}}_{i}, \overline{\boldsymbol{e}}_{i}\right)$=$\nabla_{\overline{\boldsymbol{e}}_{i}}^{\bar{N}} \overline{\boldsymbol{e}}_{i}-\nabla_{\overline{\boldsymbol{e}}_{i}}^{\bar{M}} \overline{\boldsymbol{e}}_{i}$ is also horizontal. Thus H_M=$\sum\limits_{i} \boldsymbol{B}_{\bar{M}}\left(\overline{\boldsymbol{e}}_{i}, \overline{\boldsymbol{e}}_{i}\right)$ is horizontal. On the other hand,

$ \begin{aligned} \nabla_{\boldsymbol{e}_{i}}^{N} \boldsymbol{e}_{i} &=\pi_{*}\left(\nabla_{\bar{\boldsymbol{e}}_{i}}^{\bar{N}} \overline{\boldsymbol{e}}_{i}\right) \\ &=\pi_{*}\left(\nabla_{\bar{\boldsymbol{e}}}^{\bar{M}} \overline{\boldsymbol{e}}_{i}+\boldsymbol{B}_{\bar{M}}\left(\overline{\boldsymbol{e}}_{i}, \overline{\boldsymbol{e}}_{i}\right)\right) \\ &=\nabla_{\boldsymbol{e}_{i}}^{M} \boldsymbol{e}_{i}+\pi_{*}\left(\boldsymbol{B}_{\bar{M}}\left(\overline{\boldsymbol{e}}_{i}, \overline{\boldsymbol{e}}_{i}\right)\right). \end{aligned} $

(16)

Comparing with the Gauss equation in N, we find that

$ \boldsymbol{B}_{M}\left(\boldsymbol{e}_{i}, \boldsymbol{e}_{i}\right)=\pi_{*}\left(\boldsymbol{B}_{\bar{M}}\left(\overline{\boldsymbol{e}}_{i}, \overline{\boldsymbol{e}}_{i}\right)\right). $

(17)

The conclusion follows immediately.

From the above lemma, we see that H_M=$0 \Leftrightarrow \boldsymbol{H}_{\bar{M}}=0$. That is, $M {\rm { minimal }} \Leftrightarrow \bar{M} \rm { minimal }$. This applies to our situation and the main theorem is fully proved.