2. 西安测绘总站,西安市西影路36号,710054;
3. 信息工程大学地理空间信息学院,郑州市科学大道62号,450001
CGCS2000坐标的启用确立了已有测绘成果的统一标准[1]。CGCS2000坐标原点位于包括海洋和大气的整个地球的质量中心,轴向在1984.0时与国际时间局定向一致,整个地球水平构造运动的无净旋转可保证定向随时间演变[2-3]。CGCS2000由约2 600个GPS大地点在2000.0历元坐标和速度下实现,以ITRF97为参考框架,其实现精度在2000.0历元时优于3 cm[4]。CGCS2000坐标系的成果转换需经过框架转换和历元归算,其中历元归算可根据已有的速度场模型或已知速度的点位进行计算[5]。中国大陆速度场模型的建立方法有欧拉矢量法、多曲面函数拟合法、克里金插值法和最小二乘配置法等,研究表明这些方法均具有相同量级的精度[5-8]。在速度场模型应用方面,已知速度测站的不均匀分布会导致格网精度不一致,因此在实际历元归算应用中,该类格网的速度精度具有较大的改善空间。本文利用中国大陆2 074个GNSS站数据解算的各站坐标及速度,基于欧拉矢量法建立3°×3°格网速度。通过与魏子卿等[4]建立的相同空间分辨率的格网速度进行比较,并基于速度场模型和已知速度的测站,对采用不同内插方法计算的历元归算精度进行分析。
1 欧拉矢量法速度场建模根据欧拉定理,将地球近似为球体,对于变化速度单位为cm/a的板块运动来说,地球半径变化可忽略不计。则欧拉矢量可表示为[9]:
| $ \boldsymbol{V}={\mathit{\pmb{Ω}}} \times \boldsymbol{r} $ | (1) |
式中,V为块体内某点的运动速度,Ω为该点的欧拉矢量,r为该点的矢径。欧拉定理在空间直角坐标系中的表示形式为[8]:
| $ \left[\begin{array}{l} \boldsymbol{V}_{x} \\ \boldsymbol{V}_{y} \\ \boldsymbol{V}_{z} \end{array}\right]=\left[\begin{array}{ccc} 0 & z & -y \\ -z & 0 & x \\ y & -x & 0 \end{array}\right]\left[\begin{array}{c} {\mathit{\pmb{Ω}}}_{x} \\ {\mathit{\pmb{Ω}}}_{y} \\ {\mathit{\pmb{Ω}}}_{z} \end{array}\right] $ | (2) |
式中,Vx、Vy、Vz为该点在地心坐标系下的速度,(x,y,z)为该点的地心坐标,Ωx、Ωy、Ωz为欧拉矢量的3个分量。
2 速度场内插方法 2.1 最近邻点插值法最近邻点插值法的原理为任意网格点的属性值与其几何位置最近点的属性值相同,即将每一个网格节点的最近邻点Lmin(xi, yi, zi)值作为待求点L(x, y, z)的节点值,其插值如下[7, 10]:
| $ V_{L}=V_{d_{i(\min )}} $ | (3) |
式中
反距离加权法是一种加权平均插值法。设在空间分布一系列离散点W(x, y, z),已知待插值点坐标为W(xi, yi, zi),根据周围离散点数值Zi(i=1, 2, …, n),通过距离加权插值法求得W点数值为[7]:
| $ Z_{W}=\sum\limits_{i=1}^{n} \frac{Z_{i}}{\left[d_{i}(x, y, z)\right]^{k}} / \sum\limits_{i=1}^{n} \frac{1}{\left[d_{i}(x, y, z)\right]^{k}} $ | (4) |
式中,0≤k≤2(本文取k =1),di(x, y, z)=
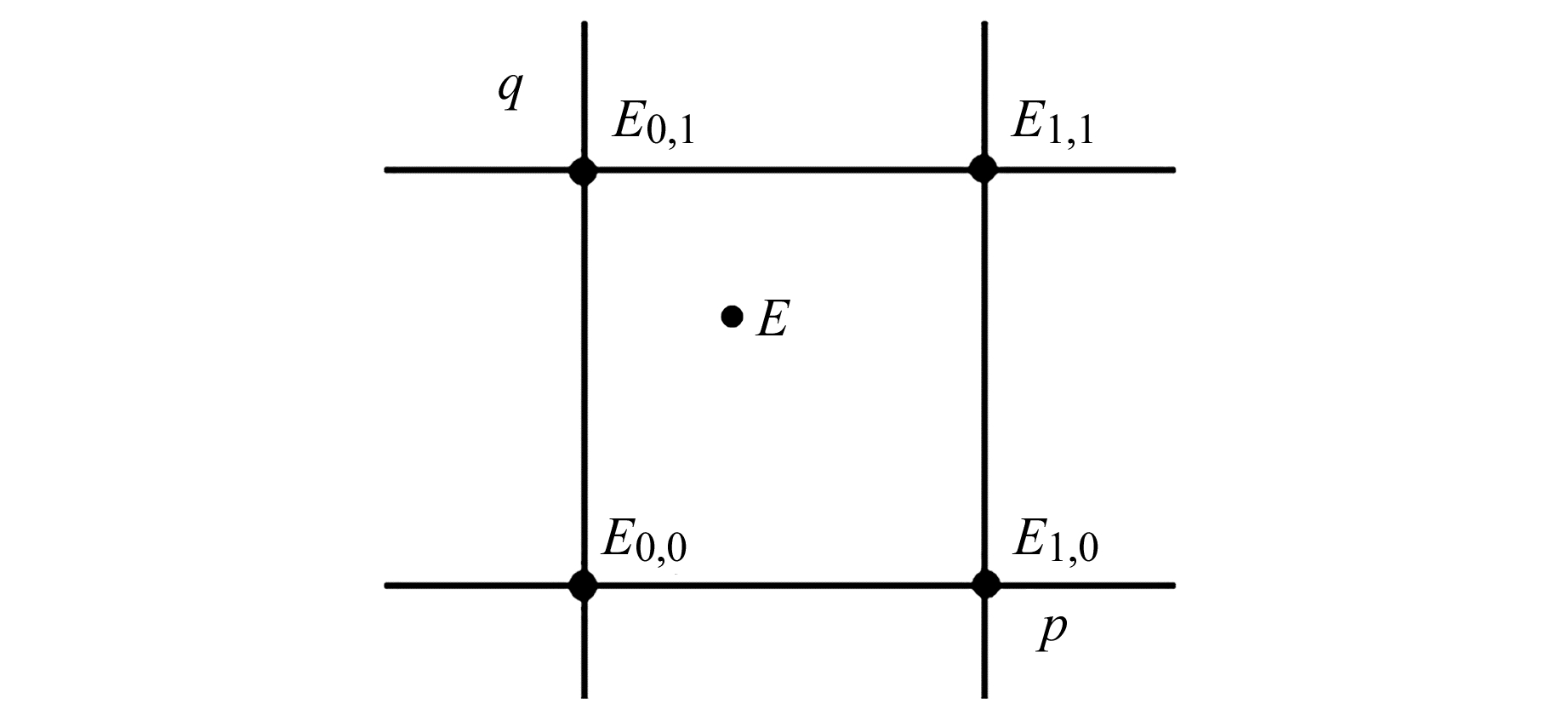
利用已建立的N°×N°速度场格网模型,选取未知点邻近的4个格网点进行双线性内插,从而得到未知点速度(图 1)。格网双线性内插公式为:
| $ \begin{array}{c} V_{E}\left(\lambda_{0}+p \Delta \lambda, \beta_{0}+q \Delta \beta\right)=(1-p)(1-q) V_{E_{0, 0}}+ \\ p(1-q) V_{E_{1, 0}}+q(1-p) V_{E_{0, 1}}+p q V_{E_{1, 1}} \end{array} $ | (5) |
|
图 1 格网双线性内插 Fig. 1 Grid bilinear interpolation |
式中,0≤p<1,0≤q<1,Δλ和Δβ分别为经线和纬线方向上的格网宽度。
3 CGCS2000坐标转换中历元归算方法CGCS2000历元归算可根据相应的速度场模型将测站观测历元的坐标归算至2000.0历元上。任意历元T1的站点坐标转换至2000.0历元可表示为[11]:
| $ \left(\begin{array}{l} X \\ Y \\ Z \end{array}\right)_{2000.0}^{X}=\left(\begin{array}{c} Y \\ Y \\ Z \end{array}\right)_{T_{1}}+\left(T_{1}-2000.0\right)\left(\begin{array}{c} V_{X} \\ V_{Y} \\ V_{Z} \end{array}\right) $ | (6) |
式中,T1为观测历元,VX、VY、VZ为测站在ITRF框架下的速度。
4 速度场建模与应用分析 4.1 速度场格网模型建立利用中国大陆2013~2019年2 074个GNSS站观测数据,以中国周边16个IGS站为基准进行单日解算和网平差,得到每个测站的单日解,并通过对测站坐标单日解时间序列进行一元线性回归解算得到各测站速度。
根据实际解算的2 074个测站速度数据建立不同方法、不同分辨率下的中国大陆速度场模型。速度场格网模型建立步骤如下:1)将经纬度范围(72°~135°E,18°~54°N)按3°×3°空间分辨率分为137个格网;2)利用每个格网中已知速度的测站,根据最小二乘原理及式(2)计算每个格网的欧拉矢量;3)采用式(2)计算格网中心点速度并作为该格网的速度(中心点格网速度场);4)采用式(2)计算每个格网点的速度,并取相邻4个格网点的平均值作为该格网点的速度(4点格网速度场),用于格网内插。
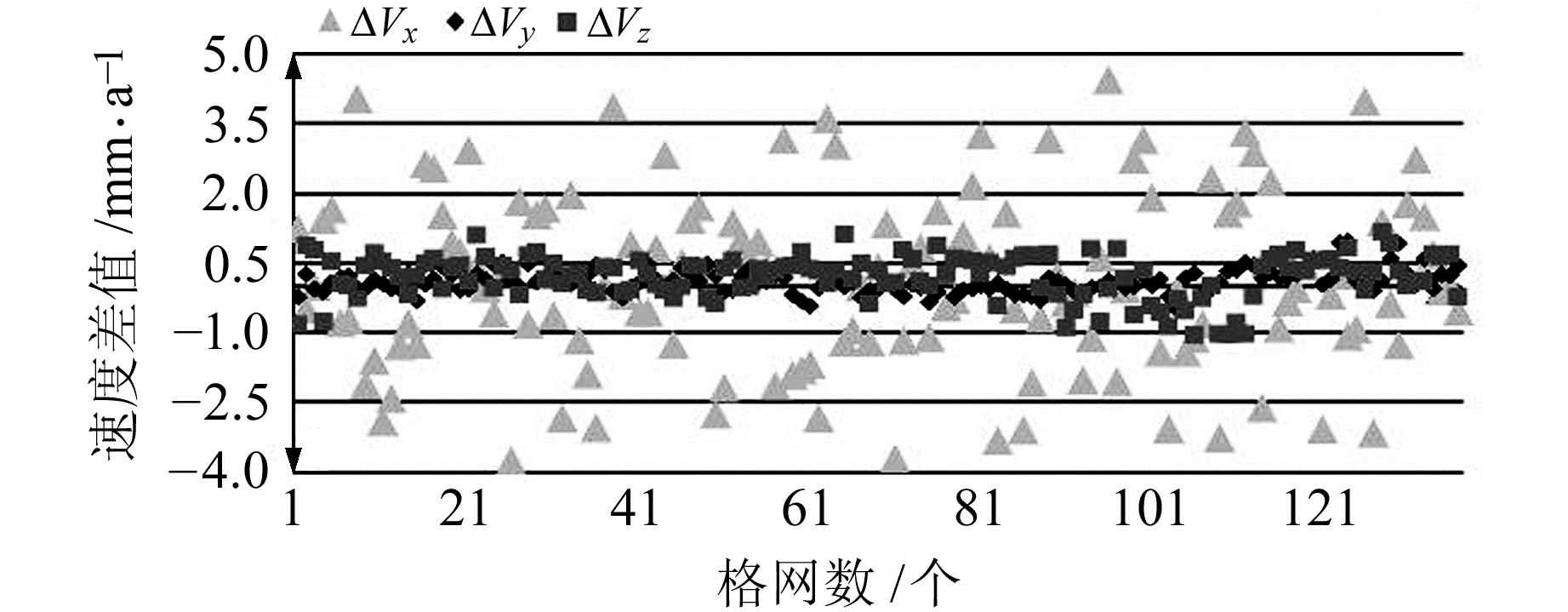
本文建立的中心点格网速度场与文献[4]对应的格网速度差值及统计值如图 2和表 1(单位mm/a)所示。
|
图 2 中心点格网速度场速度差值 Fig. 2 The difference of velocity field of central point grid |
|
|
表 1 中心点格网速度场速度差值统计 Tab. 1 Statistics of the difference of velocity field of central point grid |
由图 2可知,两个格网速度场中X方向的速度差值比Y和Z方向大一个量级,其原因为X方向速度比Y和Z方向大一个量级,相同量级的速度作差后其差值也相差一个量级。由表 1可知,两个速度场对应的每个格网中X、Y、Z三个方向差值的最值、平均值和绝对值平均值,相对其3个方向的平均速度(-31.72 mm/a、-6.42 mm/a、-9.6 mm/a)[4]差异较小。总体而言,两个速度场模型精度相当,进一步证明本文建立的速度场具有可靠性。
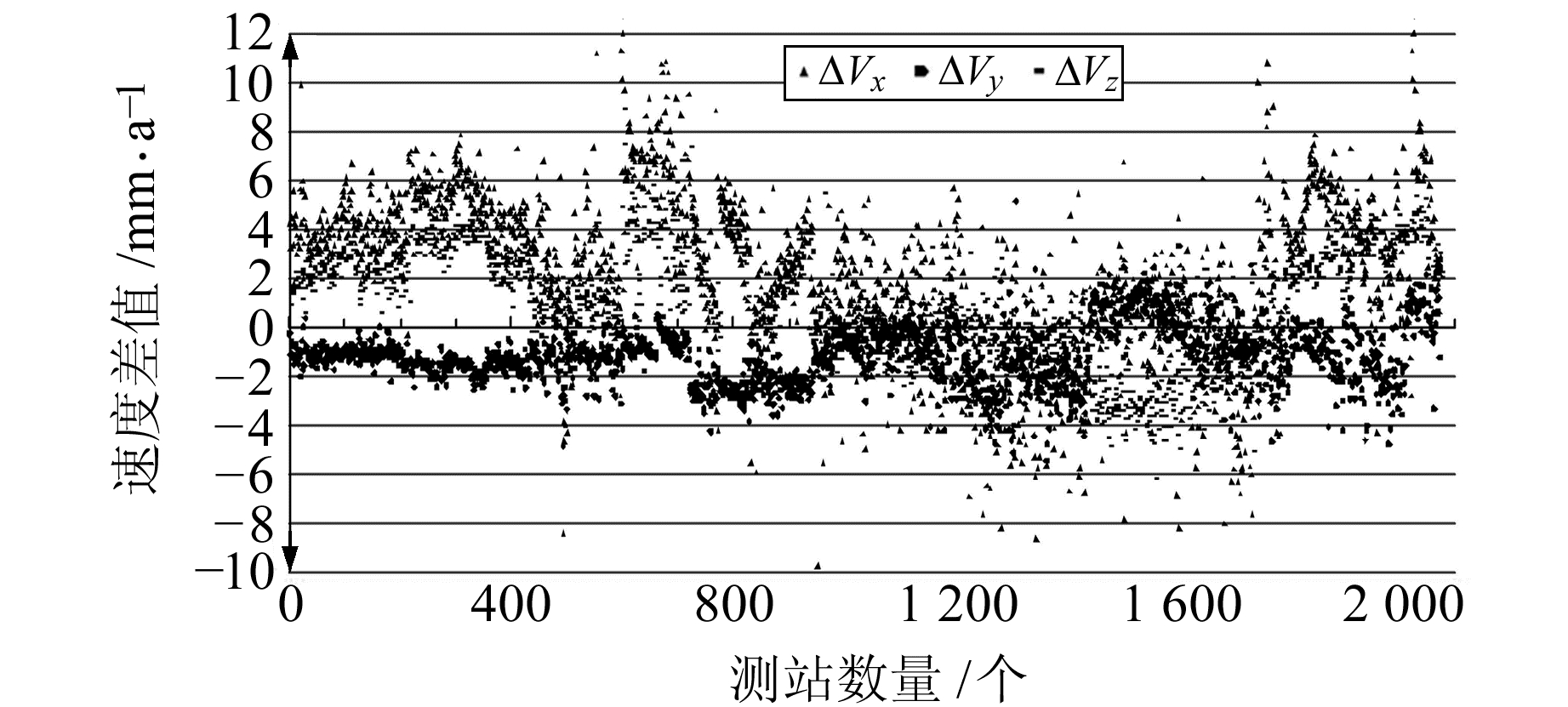
4.2 速度场在历元归算中的应用分析统计2 074个测站实测速度与其所在中心点格网速度场的速度差值,结果如图 3和表 2(单位mm/a)所示,从2020.0历元归算至2000.0历元的坐标差值如表 3(单位cm)所示。
|
图 3 2 074个测站实测速度与其所在中心点格网速度场速度差值 Fig. 3 The difference between the measured velocity of 2 074 stations and the velocity field of their central point grid |
|
|
表 2 2 074个测站实测速度与其所在中心点格网速度场速度差值统计 Tab. 2 Statistics of the difference between the measured velocity of 2 074 stations and the velocity field of their central point grid |
|
|
表 3 采用实测速度与中心点格网速度场对2 074个测站历元归算(2020.0归算至2000.0)后的坐标差值统计 Tab. 3 Statistics of the coordinate difference after epoch conversion (from 2020.0 to 2000.0) of 2 074 stations using the measured velocity and the velocity field of central point grid |
由图 3和表 2可知,2 074个测站实测速度与中心点格网速度场速度存在明显差异,X、Y、Z三个方向的最大值分别为12.00 mm/a、5.45 mm/a和8.91 mm/a,这是由于格网分辨率过大且欧拉矢量法建模存在一定精度限制所致。平均值和绝对值平均值较小,说明格网模型具有一定的合理性和有效性,能满足普遍情况下的历元估算精度。
由表 3可知,在从2020.0历元归算至2000.0历元时,平均值和绝对值平均值较小,说明利用速度场模型进行历元归算的普适性较好。测站较少区域的速度场模型精度有限,因此历元归算精度也偏低。速度间差异导致X、Y、Z三个方向的坐标差异最大达24.00 cm、10.89 cm和17.82 cm,空间三维误差达31.82 cm,表明不同测站的坐标均存在不同程度的差异,且随着时间增加差异逐渐增大。
利用本文建立的3°×3°中心点格网速度场对2 074个测站实测速度进行历元归算。为减小历元归算误差、提高归算精度,基于本文建立的3°×3°格网速度场和2 074个测站实测速度两类数据,充分考虑两者的实际有效性和适用范围后,根据每个待求速度测站周围的已知速度的测站个数及待求速度测站与已知速度测站之间的距离,采用不同方法进行速度内插,以提高历元归算精度。不同内插方法适用表见表 4。
|
|
表 4 不同内插方法适用表 Tab. 4 Applicable table of different interpolation methods |
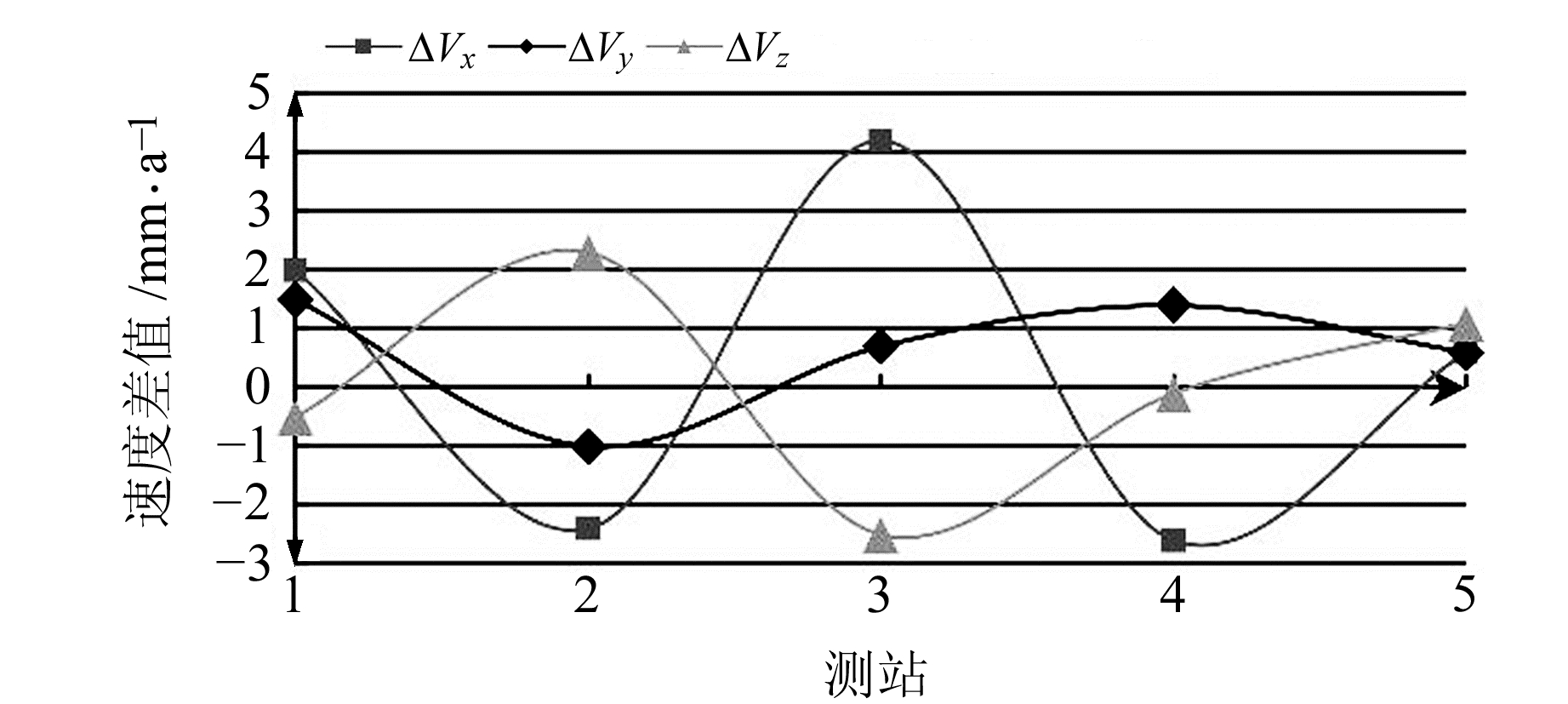
在2 074个测站中任意选取5个测站,在数据库中删除这5个测站的实际速度数据,其实际速度如表 5(单位mm/a)所示。将5个测站作为未知速度测站,比较采用表 4中内插方法计算的测站速度值与实际速度值的差异,结果如表 6(单位mm/a)和图 4所示。
|
|
表 5 5个测站的实测速度 Tab. 5 The measured velocity of 5 stations |
|
|
表 6 5个测站实测速度与不同内插方法组合速度差值统计 Tab. 6 Statistics of the difference between the measured velocity of 5 stations and the combination velocity of different interpolation methods |
|
图 4 5个测站实测速度与不同内插方法组合速度差值 Fig. 4 The difference between the measured velocity of 5 stations and the combination velocity of different interpolation methods |
由表 6和图 4可知,根据3°×3°格网速度场和2 074个测站的已知速度,采用不同内插方法得到的速度与5个测站的实测速度在X、Y、Z三个方向上差值的绝对值平均值分别为2.4 mm/a、1.0 mm/a和1.3 mm/a,两者差异较小,且差值平均值均小于1 mm/a,表明不同内插方法组合计算的速度更接近实际速度。通过对比表 6和表 2可知,充分利用2 074个测站的实测速度并组合不同内插方法计算得到的测站速度,相比于格网速度场模型速度具有更高的精度,且更接近实际值,可达到进一步提高历元归算精度的目的。
5 结语速度场精度会直接影响CGCS2000坐标系历元归算的精度,即点位坐标在CGCS2000坐标系中的精度,而单纯采用速度场模型进行的历元归算,未顾及小范围内板块相对运动较小的特点以及在已知速度测站较少的区域内建立的速度场模型精度存在局限性。基于上述原因,本文在充分利用已知速度的测站并结合4点格网速度场模型的前提下,根据不同区域范围,组合利用最邻近点插值、反距离加权和格网内插3种方法对区域内未知点速度进行历元归算。结果表明,组合不同内插方法可合理利用不同范围和不同情况下区域内已知的测站速度,相比于单纯利用速度场格网更加科学合理,可使整体归算精度得到较大幅度提升,在X、Y、Z三个方向上的历元归算精度最高可提高24.00 cm、10.89 cm和17.82 cm,对不同情况下利用已知速度的测站提高历元归算精度具有一定指导意义。
| [1] |
魏子卿. 2000中国大地坐标系及其与WGS84的比较[J]. 大地测量与地球动力学, 2008, 28(5): 1-5 (Wei Ziqing. China Geodetic Coordinate System 2000 and Its Comparison with WGS84[J]. Journal of Geodesy and Geodynamics, 2008, 28(5): 1-5)
(  0) 0) |
| [2] |
程鹏飞, 文汉江, 成英燕, 等. 2000国家大地坐标系椭球参数与GRS80和WGS84的比较[J]. 测绘学报, 2009, 38(3): 189-194 (Cheng Pengfei, Wen Hanjiang, Cheng Yingyan, et al. Parameters of the CGCS2000 Ellipsoid and Comparisons with GRS80 and WGS84[J]. Acta Geodaetica et Cartographica Sinica, 2009, 38(3): 189-194)
(  0) 0) |
| [3] |
高涵. 基于高精度时序GPS处理的区域速度场及地壳形变特征研究[D]. 西安: 长安大学, 2014 (Gao Han. Research on the Velocity Field of Areas and Crustal Deformation Characteristics on the Basis of High Precision GPS Time Series Processing[D]. Xi'an: Chang'an University, 2014)
(  0) 0) |
| [4] |
魏子卿, 刘光明, 吴富梅. 2000中国大地坐标系: 中国大陆速度场[J]. 测绘学报, 2011, 40(4): 403-410 (Wei Ziqing, Liu Guangming, Wu Fumei. China Geodetic Coordinate System 2000: Velocity Field in Mainland China[J]. Acta Geodaetica et Cartographica Sinica, 2011, 40(4): 403-410)
(  0) 0) |
| [5] |
吴富梅, 刘光明, 魏子卿. 利用局域欧拉矢量法建立CGCS2000速度场模型[J]. 武汉大学学报: 信息科学版, 2012, 37(4): 432-435 (Wu Fumei, Liu Guangming, Wei Ziqing. Velocity Field Model of CGCS2000 Based on Euler Vector of Local Area[J]. Geomatics and Information Science of Wuhan University, 2012, 37(4): 432-435)
(  0) 0) |
| [6] |
宁津生, 王华, 程鹏飞, 等. 2000国家大地坐标系框架体系建设及其进展[J]. 武汉大学学报: 信息科学版, 2015, 40(5): 569-573 (Ning Jinsheng, Wang Hua, Cheng Pengfei, et al. System Construction and Its Progress of China Geodetic Coordinate System 2000[J]. Geomatics and Information Science of Wuhan University, 2015, 40(5): 569-573)
(  0) 0) |
| [7] |
张勇. 不同数值内插方法建立我国速度场模型[D]. 北京: 中国测绘科学研究院, 2011 (Zhang Yong. Different Numerical Interpolation Method to Establish Velocity Model[D]. Beijing: Chinese Academy of Surveying and Mapping, 2011)
(  0) 0) |
| [8] |
任雅奇. 基于GPS数据的中国地壳运动速度场模型的建立[D]. 郑州: 信息工程大学, 2012 (Ren Yaqi. Establishment of Chinese Crustal Movement Velocity Model Based on the GPS Data[D]. Zhengzhou: Information Engineering University, 2012)
(  0) 0) |
| [9] |
陈醒. 中国大陆地壳运动速度场模型的构建方法研究[D]. 阜新: 辽宁工程技术大学, 2013 (Chen Xing. Study of the Algorithm on Establishing Model of Crustal Movement Velocity in China Mainland[D]. Fuxin: Liaoning Technical University, 2013)
(  0) 0) |
| [10] |
程鹏飞, 成英燕. 基于GNSS的CGCS2000数据处理技术综述[J]. 武汉大学学报: 信息科学版, 2018, 43(12): 2 071-2 078 (Cheng Pengfei, Cheng Yingyan. An Overview of the CGCS2000 Coordinate Processing Technique Based on GNSS[J]. Geomatics and Information Science of Wuhan University, 2018, 43(12): 2 071-2 078)
(  0) 0) |
| [11] |
吴伟伟. 华北地区GPS连续站坐标序列特征研究[D]. 北京: 中国地震局地震预测研究所, 2014 (Wu Weiwei. Insight into the Characteristics of GPS Time Series in North China[D]. Beijing: Institute of Earthquake Forecasting, CEA, 2014)
(  0) 0) |
2. Xi'an Technical Division of Surveying and Mapping, 36 Xiying Road, Xi'an 710054, China;
3. School of Surveying and Mapping, Information Engineering University, 62 Kexue Road, Zhengzhou 450001, China
 2022, Vol. 42
2022, Vol. 42


