2. 许昌学院城乡规划与园林学院,河南省许昌市八一路88号,461000
二十世纪中叶以来,随着科学技术的发展和计算机应用的日益广泛和深入,四元数理论在计算机图形学、捷联惯性导航、机器与机构、多体系统力学、人造卫星姿态控制等领域已得到广泛应用[1]。国内外学者[2-4]对四元数的理论和应用进行了大量研究,本文在前人研究基础上,根据四元数运算法则所导出的向量的共轭、逆和范数(模)的概念,通过向量的格拉斯曼乘积来表示平面角、大圆弧和球面角所对应的四元数,推导球面三角形公式。
1 四元数的定义与性质四元数为标量部与向量部的“和”,如:
| $ \mathit{\boldsymbol{P}} = {\mathit{\boldsymbol{p}}_0} + \mathit{\boldsymbol{p}}, \mathit{\boldsymbol{Q}} = {\mathit{\boldsymbol{q}}_0} + \mathit{\boldsymbol{q}} $ |
四元数乘积(用“
| $ \mathit{\boldsymbol{P}} \circ \mathit{\boldsymbol{Q}} = ({\mathit{\boldsymbol{p}}_0}{\mathit{\boldsymbol{q}}_0} - \mathit{\boldsymbol{p}} \cdot \mathit{\boldsymbol{q}}) + ({\mathit{\boldsymbol{p}}_0}\mathit{\boldsymbol{q}} + {\mathit{\boldsymbol{q}}_0}\mathit{\boldsymbol{p}} + \mathit{\boldsymbol{p}} \times \mathit{\boldsymbol{q}}) $ | (1) |
定义四元数Q的共轭Q *、范数‖ Q ‖、模| Q |和逆Q -1分别为[5]:
| $ \left\{ {\begin{array}{*{20}{l}} {{\mathit{\boldsymbol{Q}}^*} = {\mathit{\boldsymbol{q}}_0} - \mathit{\boldsymbol{q}}}\\ {\left\| \mathit{\boldsymbol{Q}} \right\| = |\mathit{\boldsymbol{Q}}{|^2} = \mathit{\boldsymbol{Q}} \circ {\mathit{\boldsymbol{Q}}^*} = {\mathit{\boldsymbol{Q}}^*} \circ \mathit{\boldsymbol{Q}} = \mathit{\boldsymbol{q}}_0^2 + \mathit{\boldsymbol{q}} \cdot \mathit{\boldsymbol{q}}}\\ {{\mathit{\boldsymbol{Q}}^{ - 1}} = {{\left\| \mathit{\boldsymbol{Q}} \right\|}^{ - 1}}{\mathit{\boldsymbol{Q}}^*}} \end{array}} \right. $ | (2) |
显然存在
单位四元数
| $ \mathit{\boldsymbol{\hat Q}} = {\rm{cos}}\theta + \mathit{\boldsymbol{\hat qsin}}\theta $ | (3) |
式中,
| $ \mathit{\boldsymbol{\hat a}} \cdot \mathit{\boldsymbol{\hat b}} = {\rm{cos}}\theta , \mathit{\boldsymbol{\hat a}} \times \mathit{\boldsymbol{\hat b}} = \mathit{\boldsymbol{\hat q}}{\rm{sin}}\theta $ |
则单位四元数
| $ \begin{array}{*{20}{c}} {\mathit{\boldsymbol{\hat Q}} = \mathit{\boldsymbol{\hat a}} \cdot \mathit{\boldsymbol{\hat b}} + \mathit{\boldsymbol{\hat a}} \times \mathit{\boldsymbol{\hat b}} = }\\ { - \mathit{\boldsymbol{\hat b}} \circ \mathit{\boldsymbol{\hat a}} = \mathit{\boldsymbol{\hat b}} \circ {{\mathit{\boldsymbol{\hat a}}}^*} = \mathit{\boldsymbol{\hat b}} \circ {{\mathit{\boldsymbol{\hat a}}}^{ - 1}}} \end{array} $ | (4) |
根据式(4)可知,单位四元数可用2个单位向量的格拉斯曼乘积表示。将具有相同起点的2个单位向量

|
图 1 四元数在单位圆上的表示 Fig. 1 The representation of quaternion on a unit circle |
如图 1所示,大圆弧
| $ \theta \mathit{\boldsymbol{\hat q}} = \overset\frown{AB} \buildrel \wedge \over = \mathit{\boldsymbol{\hat Q}} = {\rm{cos}}\theta + \mathit{\boldsymbol{\hat q}}{\rm{sin}}\theta = \mathit{\boldsymbol{\hat b}} \circ {{\mathit{\boldsymbol{\hat a}}}^{ - 1}} $ | (5) |
式(5)表明,平面角的单位四元数对应终边单位向量和始边单位向量逆的格拉斯曼乘积。
由式(3)可知,当θ=0或θ=π时,
| $ \begin{array}{*{20}{c}} { - \theta \mathit{\boldsymbol{\hat q}} = \overset\frown{BA} \buildrel \wedge \over = {{\mathit{\boldsymbol{\hat Q}}}^{ - 1}} = {{\mathit{\boldsymbol{\hat Q}}}^*} = }\\ {{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\rm{cos}}\theta - \mathit{\boldsymbol{\hat q}}{\rm{sin}}\theta = \mathit{\boldsymbol{\hat a}} \circ {{\mathit{\boldsymbol{\hat b}}}^{ - 1}}} \end{array} $ |
与四元数-
| $ \begin{array}{*{20}{c}} { - \mathit{\boldsymbol{\hat Q}} = - {\rm{cos}}\theta - \mathit{\boldsymbol{\hat q}}{\rm{sin}}\theta = - \mathit{\boldsymbol{\hat b}} \circ {{\mathit{\boldsymbol{\hat a}}}^{ - 1}} = }\\ {\mathit{\boldsymbol{\hat b}} \circ {{( - \mathit{\boldsymbol{\hat a}})}^{ - 1}} \buildrel \wedge \over = - (\pi - \theta )\mathit{\boldsymbol{\hat q}} = \overset\frown{A'B}} \end{array} $ |
即从径对称点A′到点B的大圆弧
将球面上不在同一直径上的3个点A、B和C用3个大圆弧连接起来,所围成的图形为球面三角形,3个大圆弧为球面三角形的边,2个大圆弧相交所成的角为球面角。设大圆弧

|
图 2 球面三角形边和角的四元数表示 Fig. 2 The representation of quaternion of side and angle in a spherical triangle |
| $ \begin{array}{*{20}{c}} {\overrightarrow {OA} = \mathit{\boldsymbol{\hat a}}, \overrightarrow {OB} = \mathit{\boldsymbol{\hat b}}, \overrightarrow {OC} = \mathit{\boldsymbol{\hat c}}}\\ {{{\mathit{\boldsymbol{\hat c}}}_ \bot } = \overrightarrow {OD} = \frac{{\mathit{\boldsymbol{\hat a}} \times \mathit{\boldsymbol{\hat b}}}}{{|\mathit{\boldsymbol{\hat a}} \times \mathit{\boldsymbol{\hat b}}|}} \bot \overset\frown{AB} = c, }\\ {{{\mathit{\boldsymbol{\hat b}}}^\prime }_ \bot = \overrightarrow {OE} = \frac{{\mathit{\boldsymbol{\hat a}} \times \mathit{\boldsymbol{\hat b}}}}{{|\mathit{\boldsymbol{\hat a}} \times \mathit{\boldsymbol{\hat b}}|}} \bot \overset\frown{AC} = b} \end{array} $ |
根据式(5)可知:
| $ \begin{array}{l} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} c{{\mathit{\boldsymbol{\hat c}}}_ \bot } = \overset\frown{AB} \buildrel \wedge \over = {\mathit{\boldsymbol{C}}_{{\rm{边}}}} = {\rm{cos}}c + {{\mathit{\boldsymbol{\hat c}}}_ \bot } {\rm{sin}}c = \mathit{\boldsymbol{\hat b}} \circ {{\mathit{\boldsymbol{\hat a}}}^{ - 1}}\\ {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} b\mathit{\boldsymbol{\hat b}}_ \bot ^\prime = \overset\frown{AC} \buildrel \wedge \over = {\mathit{\boldsymbol{B}}_{边}} = {\rm{cos}}b + \mathit{\boldsymbol{\hat b}}_ \bot ^\prime {\rm{sin}}b = \mathit{\boldsymbol{\hat c}} \circ {{\mathit{\boldsymbol{\hat a}}}^{ - 1}}\\ A\mathit{\boldsymbol{\hat a}} = \measuredangle BAC = \angle {B^\prime }O{C^\prime } = \overset\frown{B'C'} = \overset\frown{DE} \buildrel \wedge \over = {\mathit{\boldsymbol{A}}_{{\rm{角}}}} = \\ {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\rm{cos}}A + \mathit{\boldsymbol{\hat a}}{\rm{sin}}A = \mathit{\boldsymbol{\hat b}}_ \bot ^\prime \circ \mathit{\boldsymbol{\hat c}}_ \bot ^{ - 1} \end{array} $ |
式中,C边和B边分别表示c边和b边的四元数,A角表示A角的四元数。如果图 2中球面三角形b边沿相反方向(朝A点)度量,由于本文已约定角的度量方向与其所在平面的单位法向量方向一致,因此存在:
| $ b{\mathit{\boldsymbol{\hat b}}_ \bot } = \overset\frown{CA} = {\mathit{\boldsymbol{B}}_{{\rm{123}}}} = {\rm{cos}}b + {\mathit{\boldsymbol{\hat b}}_ \bot }{\rm{sin}}b = \mathit{\boldsymbol{\hat a}} \circ {\mathit{\boldsymbol{\hat c}}^{ - 1}} $ | (6) |
| $ \begin{array}{*{20}{c}} {A\mathit{\boldsymbol{\hat a}} = \overset\frown{DE} \buildrel \wedge \over = {\mathit{\boldsymbol{A}}_{{\rm{123}}}} = {{\mathit{\boldsymbol{\hat b}}}_ \bot } \circ \mathit{\boldsymbol{\hat c}}_ \bot ^{ - 1} = }\\ {{\rm{cos}}(\pi + A) + \mathit{\boldsymbol{\hat a}}{\rm{sin}}(\pi + A)} \end{array} $ | (7) |
式中,
上述分析表明,球面三角形边长的四元数为球心指向边长终点的单位向量与球心指向边长始点的单位向量逆的格拉斯曼乘积。如果利用四元数表示球面三角形的二面角,按照球面角的度量方向(本文约定指向球面角顶点的方向为正),角的四元数为终大圆弧(指向顶点)平面法向的单位向量与始大圆弧(背向顶点)平面法向的单位向量逆的格拉斯曼乘积,式(7)为其三角函数形式。
4 利用四元数推导球面三角形公式本节将在3个角都小于90°的条件下推导球面三角形的有关公式,该结论适用于任何球面三角形。设存在球面三角形ABC,将各顶点与球心O连接,可得球心三面角O-ABC (图 3)。球心O指向A点、B点和C点的单位向量分别为
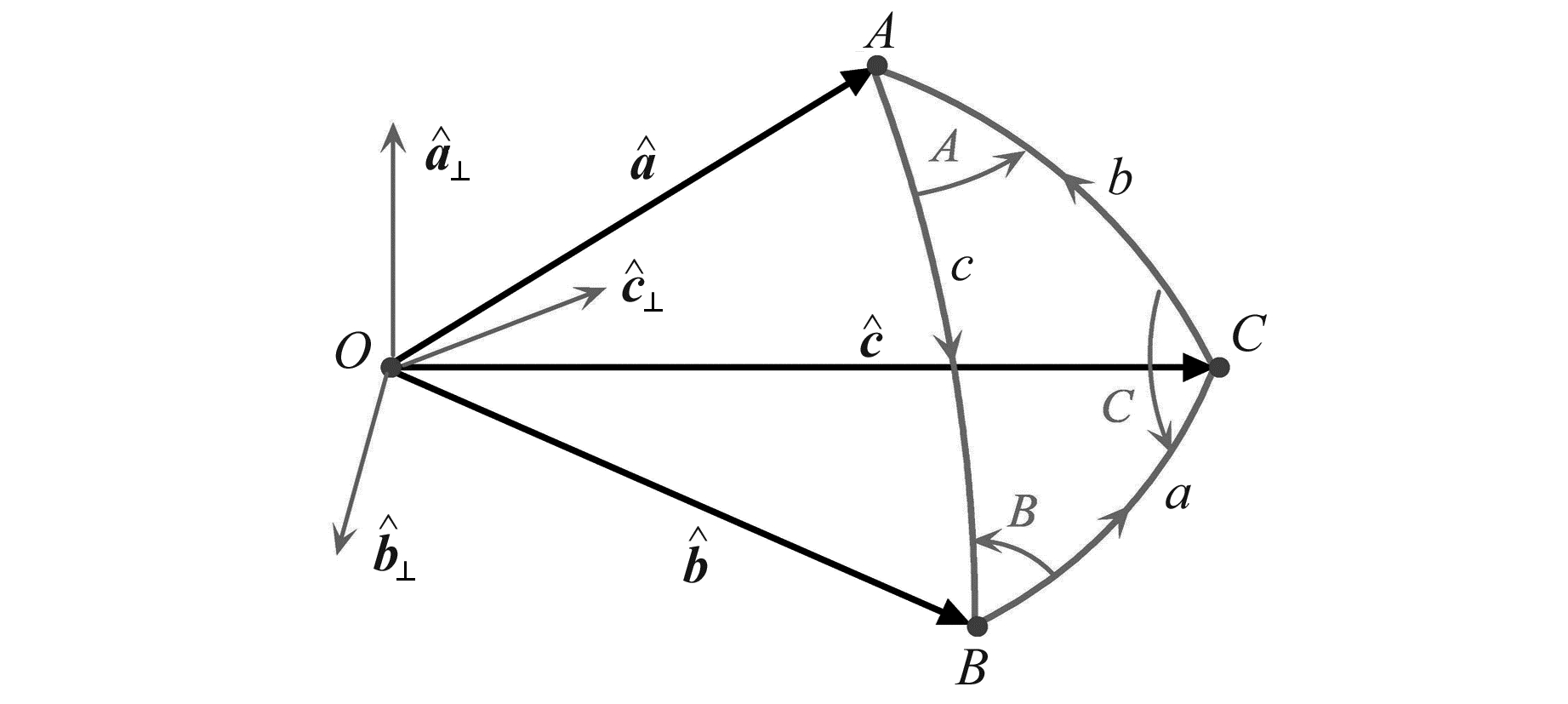
|
图 3 球面三角形与单位四元数 Fig. 3 Spherical triangle and unit quaternion |
| $ \begin{array}{*{20}{l}} {{{\mathit{\boldsymbol{\hat a}}}_ \bot } = \frac{{\mathit{\boldsymbol{\hat b}} \times \mathit{\boldsymbol{\hat c}}}}{{\mathit{\boldsymbol{\hat b}} \times \mathit{\boldsymbol{\hat c}}}} = \frac{{\mathit{\boldsymbol{\hat b}} \times \mathit{\boldsymbol{\hat c}}}}{{{\rm{sin}}a}}, }\\ {{{\mathit{\boldsymbol{\hat b}}}_ \bot } = \frac{{\mathit{\boldsymbol{\hat c}} \times \mathit{\boldsymbol{\hat a}}}}{{|\mathit{\boldsymbol{\hat c}} \times \mathit{\boldsymbol{\hat a}}|}} = \frac{{\mathit{\boldsymbol{\hat c}} \times \mathit{\boldsymbol{\hat a}}}}{{{\rm{sin}}b}}, }\\ {{{\mathit{\boldsymbol{\hat c}}}_ \bot } = \frac{{\mathit{\boldsymbol{\hat a}} \times \mathit{\boldsymbol{\hat b}}}}{{|\mathit{\boldsymbol{\hat a}} \times \mathit{\boldsymbol{\hat b}}|}} = \frac{{\mathit{\boldsymbol{\hat a}} \times \mathit{\boldsymbol{\hat b}}}}{{{\rm{sin}}c}}} \end{array} $ |
根据上节的讨论,可知球面三角形角的四元数为:
| $ \left\{ \begin{array}{l} \begin{array}{*{20}{c}} {A\mathit{\boldsymbol{\hat a}} \buildrel \wedge \over = {\mathit{\boldsymbol{A}}_{{\rm{角}}}} = {{\mathit{\boldsymbol{\hat b}}}_ \bot } \circ \mathit{\boldsymbol{\hat c}}_ \bot ^{ - 1} = {\rm{cos}}(\pi + A) + }\\ {\mathit{\boldsymbol{\hat a}}{\rm{sin}}(\pi + A) = {{\mathit{\boldsymbol{\hat c}}}_ \bot } \cdot {{\mathit{\boldsymbol{\hat b}}}_ \bot } + {{\mathit{\boldsymbol{\hat c}}}_ \bot } \times {{\mathit{\boldsymbol{\hat b}}}_ \bot }} \end{array}\\ \begin{array}{*{20}{c}} {B\mathit{\boldsymbol{\hat b}} \buildrel \wedge \over = {\mathit{\boldsymbol{B}}_{{\rm{角}}}} = {{\mathit{\boldsymbol{\hat c}}}_ \bot } \circ \mathit{\boldsymbol{\hat a}}_ \bot ^{ - 1} = {\rm{cos}}(\pi + B)}\\ {\mathit{\boldsymbol{\hat b}}{\rm{sin}}(\pi + B) = {{\mathit{\boldsymbol{\hat a}}}_ \bot } \cdot {{\mathit{\boldsymbol{\hat c}}}_ \bot } + {{\mathit{\boldsymbol{\hat a}}}_ \bot } \times {{\mathit{\boldsymbol{\hat c}}}_ \bot }} \end{array}\\ \begin{array}{*{20}{c}} {C\mathit{\boldsymbol{\hat c}} \buildrel \wedge \over = {\mathit{\boldsymbol{C}}_{{\rm{角}}}} = {{\mathit{\boldsymbol{\hat a}}}_ \bot } \circ \mathit{\boldsymbol{\hat b}}_ \bot ^{ - 1} = {\rm{cos}}(\pi + C) + }\\ {\mathit{\boldsymbol{\hat c}}{\rm{sin}}(\pi + C) = {{\mathit{\boldsymbol{\hat b}}}_ \bot } \cdot {{\mathit{\boldsymbol{\hat a}}}_ \bot } + {{\mathit{\boldsymbol{\hat b}}}_ \bot } \times {{\mathit{\boldsymbol{\hat a}}}_ \bot }} \end{array} \end{array} \right. $ | (8) |
球面三角形边的四元数为:
| $ \left\{ \begin{array}{l} \begin{array}{*{20}{l}} {a{{\mathit{\boldsymbol{\hat a}}}_ \bot } = \overset\frown {BC} \buildrel \wedge \over = {\mathit{\boldsymbol{a}}_{{\rm{边}}}} = \mathit{\boldsymbol{\hat c}} \circ {{\mathit{\boldsymbol{\hat b}}}^{ - 1}} = {\rm{cos}}a + }\\ {{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {{\mathit{\boldsymbol{\hat a}}}_ \bot }{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\rm{sin}}a = \mathit{\boldsymbol{\hat b}} \cdot \mathit{\boldsymbol{\hat c}} + \mathit{\boldsymbol{\hat b}} \times \mathit{\boldsymbol{\hat c}}} \end{array}\\ \begin{array}{*{20}{l}} {b{{\mathit{\boldsymbol{\hat b}}}_ \bot } = \overset\frown {CA} \buildrel \wedge \over = {\mathit{\boldsymbol{b}}_{{\rm{边}}}} = \mathit{\boldsymbol{\hat a}} \circ {{\mathit{\boldsymbol{\hat c}}}^{ - 1}} = {\rm{cos}}b + }\\ {{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {{\mathit{\boldsymbol{\hat b}}}_ \bot }{\kern 1pt} {\kern 1pt} {\kern 1pt} {\rm{sin}}b = \mathit{\boldsymbol{\hat c}} \cdot \mathit{\boldsymbol{\hat a}} + \mathit{\boldsymbol{\hat c}} \times \mathit{\boldsymbol{\hat a}}} \end{array}\\ \begin{array}{*{20}{l}} {c{{\mathit{\boldsymbol{\hat c}}}_ \bot } = \overset\frown {AB} \buildrel \wedge \over = {\mathit{\boldsymbol{c}}_{{\rm{边}}}} = \mathit{\boldsymbol{\hat b}} \circ {{\mathit{\boldsymbol{\hat a}}}^{ - 1}} = {\rm{cos}}c + }\\ {{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {{\mathit{\boldsymbol{\hat c}}}_ \bot }{\kern 1pt} {\kern 1pt} {\kern 1pt} {\rm{sin}}c = \mathit{\boldsymbol{\hat a}} \cdot \mathit{\boldsymbol{\hat b}} + \mathit{\boldsymbol{\hat a}} \times \mathit{\boldsymbol{\hat b}}} \end{array} \end{array} \right. $ | (9) |
根据式(9)可得:
| $ {\mathit{\boldsymbol{c}}_{{\rm{边}}}} \circ {\mathit{\boldsymbol{b}}_{{\rm{边}}}} = (\mathit{\boldsymbol{\hat b}} \circ {\mathit{\boldsymbol{\hat a}}^{ - 1}}) \circ (\mathit{\boldsymbol{\hat a}} \circ {\mathit{\boldsymbol{\hat c}}^{ - 1}}) = \mathit{\boldsymbol{\hat b}} \circ {\mathit{\boldsymbol{\hat c}}^{ - 1}} = \mathit{\boldsymbol{a}}_{{\rm{边}}}^{ - 1} $ |
将上式写成三角函数形式:
| $ \begin{array}{*{20}{c}} {{\rm{cos}}a - {{\mathit{\boldsymbol{\hat a}}}_ \bot }{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\rm{sin}}a = }\\ {({\rm{cos}}c + {{\mathit{\boldsymbol{\hat c}}}_ \bot }{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\rm{sin}}c) \cdot ({\rm{cos}}b + {{\mathit{\boldsymbol{\hat b}}}_ \bot }{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\rm{sin}}b)} \end{array} $ | (10) |
按照式(1)进行乘法运算,使得式(10)等号两边的标量部分相等,则:
| $ {\rm{cos}}a = {\rm{cos}}b{\rm{cos}}c - {\mathit{\boldsymbol{\hat c}}_ \bot } \cdot {\mathit{\boldsymbol{\hat b}}_ \bot }{\kern 1pt} {\kern 1pt} {\kern 1pt} {\rm{sin}}b{\rm{sin}}c $ |
根据式(8),可将式(10)写为:
| $ {\rm{cos}}a = {\rm{cos}}b{\rm{cos}}c + {\rm{sin}}b{\rm{sin}}c{\rm{cos}}A $ | (11) |
式(11)即为球面三角形边余弦公式。
令式(10)等号两边的向量部分相等,则:
| $ \begin{array}{*{20}{c}} { - {{\mathit{\boldsymbol{\hat a}}}_ \bot }{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\rm{sin}}a = {{\mathit{\boldsymbol{\hat c}}}_ \bot }{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\rm{cos}}b{\rm{sin}}c + }\\ {{{\mathit{\boldsymbol{\hat b}}}_ \bot }{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\rm{sin}}b{\rm{cos}}c + {{\mathit{\boldsymbol{\hat c}}}_ \bot } \times {{\mathit{\boldsymbol{\hat b}}}_ \bot }{\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\rm{sin}}b{\rm{sin}}c} \end{array} $ | (12) |
将式(12)等号两边分别点乘
| $ \left\{ {\begin{array}{*{20}{l}} {{\rm{sin}}a{\kern 1pt} {\rm{cos}}B = {\rm{cos}}b{\kern 1pt} {\rm{sin}}c - \sin b{\rm{cos}}c{\kern 1pt} {\rm{cos}}A}\\ {{\rm{sin}}a{\kern 1pt} {\rm{cos}}C = {\rm{cos}}c{\kern 1pt} {\rm{sin}}b - \sin c{\rm{cos}}b{\kern 1pt} {\rm{cos}}A} \end{array}} \right. $ | (13) |
式(13)为球面三角形的第一五元素公式。
另外,将式(12)等号两边点乘
| $ \mathit{\boldsymbol{\hat a}} \cdot {{\mathit{\boldsymbol{\hat b}}}_ \bot } = 0, \mathit{\boldsymbol{\hat a}} \cdot {{\mathit{\boldsymbol{\hat c}}}_ \bot } = 0, \mathit{\boldsymbol{\hat a}} \cdot {{\mathit{\boldsymbol{\hat a}}}_ \bot } = \frac{{\mathit{\boldsymbol{\hat a}} \cdot (\mathit{\boldsymbol{\hat b}} \times \mathit{\boldsymbol{\hat c}})}}{{{\rm{sin}}a}} $ |
可得:
| $ \frac{{{\rm{sin}}A}}{{{\rm{sin}}a}} = \frac{{\mathit{\boldsymbol{\hat a}} \cdot (\mathit{\boldsymbol{\hat b}} \times \mathit{\boldsymbol{\hat c}})}}{{{\rm{sin}}a{\kern 1pt} {\rm{sin}}b{\kern 1pt} {\rm{sin}}c}} $ | (14) |
式(14)即为球面三角形正弦公式的另一种形式。
根据式(9)可得:
| $ \begin{array}{l} {\mathit{\boldsymbol{a}}_{{\rm{边}}}} \circ {\mathit{\boldsymbol{c}}_{{\rm{边}}}} = (\mathit{\boldsymbol{\hat c}} \circ {{\mathit{\boldsymbol{\hat b}}}^{ - 1}}) \circ (\mathit{\boldsymbol{\hat b}} \circ {{\mathit{\boldsymbol{\hat a}}}^{ - 1}}) = \mathit{\boldsymbol{\hat c}} \circ {{\mathit{\boldsymbol{\hat a}}}^{ - 1}} = \mathit{\boldsymbol{b}}_{{\rm{边}}}^{ - 1}\\ {\mathit{\boldsymbol{b}}_{{\rm{边}}}} \circ {\mathit{\boldsymbol{a}}_{{\rm{边}}}} = (\mathit{\boldsymbol{\hat a}} \circ {{\mathit{\boldsymbol{\hat c}}}^{ - 1}}) \circ (\mathit{\boldsymbol{\hat c}} \circ {{\mathit{\boldsymbol{\hat b}}}^{ - 1}}) = \mathit{\boldsymbol{\hat a}} \circ {{\mathit{\boldsymbol{\hat b}}}^{ - 1}} = \mathit{\boldsymbol{c}}_{{\rm{边}}}^{ - 1} \end{array} $ |
采用类似的推导过程,可分别得到球面三角形边的余弦公式:
| $ {{\rm{cos}}b = {\rm{cos}}c{\kern 1pt} {\rm{cos}}a + {\rm{sin}}c{\kern 1pt} {\rm{sin}}a{\kern 1pt} {\rm{cos}}B} $ | (15) |
| $ {{\rm{cos}}c = {\rm{cos}}a{\kern 1pt} {\rm{cos}}b + {\rm{sin}}a{\kern 1pt} {\rm{sin}}b{\kern 1pt} {\rm{cos}}C} $ | (16) |
第一五元素公式为:
| $ \left\{ {\begin{array}{*{20}{l}} {{\rm{sin}}b{\kern 1pt} {\rm{cos}}C = {\rm{cos}}c{\kern 1pt} {\rm{sin}}a - {\rm{sin}}c{\kern 1pt} {\rm{cos}}a{\kern 1pt} {\rm{cos}}B}\\ {{\rm{sin}}b{\kern 1pt} {\rm{cos}}A = {\rm{cos}}a{\kern 1pt} {\rm{sin}}c - {\rm{sin}}a{\kern 1pt} {\rm{cos}}c{\kern 1pt} {\rm{cos}}B} \end{array}} \right. $ | (17) |
| $ \left\{ {\begin{array}{*{20}{l}} {{\rm{sin}}c{\kern 1pt} {\kern 1pt} {\rm{cos}}B = {\rm{cos}}b{\kern 1pt} {\rm{sin}}a - {\rm{sin}}b{\kern 1pt} {\rm{cos}}a{\kern 1pt} {\rm{cos}}C}\\ {{\rm{sin}}c{\kern 1pt} {\kern 1pt} {\rm{cos}}A = {\rm{cos}}a{\kern 1pt} {\rm{sin}}b - {\rm{sin}}a{\kern 1pt} {\rm{cos}}b{\kern 1pt} {\rm{cos}}C} \end{array}} \right. $ | (18) |
正弦公式为:
| $ {\frac{{{\rm{sin}}B}}{{{\rm{sin}}b}} = \frac{{\mathit{\boldsymbol{\hat b}} \cdot (\mathit{\boldsymbol{\hat c}} \times \mathit{\boldsymbol{\hat a}})}}{{{\rm{sin}}a{\kern 1pt} {\rm{sin}}b{\kern 1pt} {\rm{sin}}c}}} $ | (19) |
| $ {\frac{{{\rm{sin}}C}}{{{\rm{sin}}c}} = \frac{{\mathit{\boldsymbol{\hat c}} \cdot (\mathit{\boldsymbol{\hat a}} \times \mathit{\boldsymbol{\hat b}})}}{{{\rm{sin}}a{\kern 1pt} {\rm{sin}}b{\kern 1pt} {\rm{sin}}c}}} $ | (20) |
因为
| $ \mathit{\boldsymbol{\hat a}} \cdot (\mathit{\boldsymbol{\hat b}} \times \mathit{\boldsymbol{\hat c}}) = \mathit{\boldsymbol{\hat b}} \cdot (\mathit{\boldsymbol{\hat c}} \times \mathit{\boldsymbol{\hat a}}) = \mathit{\boldsymbol{\hat c}} \cdot (\mathit{\boldsymbol{\hat a}} \times \mathit{\boldsymbol{\hat b}}) $ |
则:
| $ \frac{{{\rm{sin}}A}}{{{\rm{sin}}a}} = \frac{{{\rm{sin}}B}}{{{\rm{sin}}b}} = \frac{{{\rm{sin}}C}}{{{\rm{sin}}c}} $ | (21) |
式(21)为通常情况下的球面三角形的正弦公式。
4.2 角余弦公式和第二五元素公式推导根据式(8)可得:
| $ \begin{array}{l} {\mathit{\boldsymbol{B}}_{{\rm{角}}}} \circ {\mathit{\boldsymbol{C}}_{{\rm{角}}}} = ({{\mathit{\boldsymbol{\hat c}}}_ \bot } \circ \mathit{\boldsymbol{\hat a}}_ \bot ^{ - 1}) \circ ({{\mathit{\boldsymbol{\hat a}}}_ \bot } \circ \mathit{\boldsymbol{\hat b}}_ \bot ^{ - 1}) = \\ {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {\kern 1pt} {{\mathit{\boldsymbol{\hat c}}}_ \bot } \circ \mathit{\boldsymbol{\hat b}}_ \bot ^{ - 1} = \mathit{\boldsymbol{A}}_{{\rm{角}}}^{ - 1} \end{array} $ |
将上式写成三角函数形式:
| $ \begin{array}{*{20}{l}} {{\rm{cos}}(\pi + A) - \mathit{\boldsymbol{\hat a}}{\rm{sin}}(\pi + A) = [{\rm{cos}}(\pi + B) + }\\ {\mathit{\boldsymbol{\hat b}}{\rm{sin}}(\pi + B)] \circ [{\rm{cos}}(\pi + C) + \mathit{\boldsymbol{\hat c}}{\rm{sin}}(\pi + C)]} \end{array} $ |
令等号两边的标量部分和向量部分分别相等,则:
| $ \left\{ {\begin{array}{*{20}{l}} {{\rm{ - cos}}A = {\rm{cos}}B{\rm{cos}}C - \mathit{\boldsymbol{\hat c}} \cdot \mathit{\boldsymbol{\hat b}}{\rm{sin}}B{\rm{sin}}C}\\ {\mathit{\boldsymbol{\hat a}}{\rm{sin}}A = \mathit{\boldsymbol{\hat b}}{\rm{sin}}B{\rm{cos}}C + \mathit{\boldsymbol{\hat c}}{\rm{cos}}B{\rm{sin}}C + \mathit{\boldsymbol{\hat b}} \times \mathit{\boldsymbol{\hat c}}{\rm{sin}}B{\rm{sin}}C} \end{array}} \right. $ | (22) |
根据式(22)的第1式和式(9),可得球面三角形的角余弦公式为:
| $ {\rm{cos}}A = - {\rm{cos}}B{\rm{cos}}C + {\rm{sin}}B{\rm{sin}}C{\rm{cos}}a $ | (23) |
将式(22)的第2式等号两边分别点乘
| $ \left\{ \begin{array}{l} {\rm{sin}}A{\rm{cos}}c = {\rm{cos}}C{\rm{sin}}B + {\rm{sin}}C{\rm{cos}}B{\rm{cos}}a\\ {\rm{sin}}A{\rm{cos}}b = {\rm{cos}}B{\rm{sin}}C + {\rm{sin}}B{\rm{cos}}C{\rm{cos}}a \end{array} \right. $ | (24) |
将式(22)的第2式等号两边点乘
| $ \begin{array}{*{20}{l}} {\mathit{\boldsymbol{\hat b}} \cdot {{\mathit{\boldsymbol{\hat a}}}_ \bot } = \mathit{\boldsymbol{\hat c}} \cdot {{\mathit{\boldsymbol{\hat a}}}_ \bot } = 0, \mathit{\boldsymbol{\hat b}} \times \mathit{\boldsymbol{\hat c}} = }\\ {{\rm{sin}}a{{\mathit{\boldsymbol{\hat a}}}_ \bot }, \mathit{\boldsymbol{\hat a}} \cdot {{\mathit{\boldsymbol{\hat a}}}_ \bot } = \frac{{\mathit{\boldsymbol{\hat a}} \cdot (\mathit{\boldsymbol{\hat b}} \times \mathit{\boldsymbol{\hat c}})}}{{{\rm{sin}}a}}} \end{array} $ |
可得:
同理,根据式(8)可得:
| $ \begin{array}{l} \begin{array}{*{20}{c}} {{\mathit{\boldsymbol{C}}_{{\rm{角}}}} \circ {\mathit{\boldsymbol{A}}_{{\rm{角}}}} = ({{\mathit{\boldsymbol{\hat a}}}_ \bot } \circ \mathit{\boldsymbol{\hat b}}_ \bot ^{ - 1}) \circ ({{\mathit{\boldsymbol{\hat b}}}_ \bot } \circ \mathit{\boldsymbol{\hat c}}_ \bot ^{ - 1})}\\ {{{\mathit{\boldsymbol{\hat a}}}_ \bot } \circ \mathit{\boldsymbol{\hat c}}_ \bot ^{ - 1} = \mathit{\boldsymbol{B}}_{{\rm{角}}}^{ - 1}} \end{array}\\ \begin{array}{*{20}{c}} {{\mathit{\boldsymbol{A}}_{{\rm{角}}}} \circ {\mathit{\boldsymbol{B}}_{{\rm{角}}}} = ({{\mathit{\boldsymbol{\hat b}}}_ \bot } \circ \mathit{\boldsymbol{\hat c}}_ \bot ^{ - 1}) \circ ({{\mathit{\boldsymbol{\hat c}}}_ \bot } \circ \mathit{\boldsymbol{\hat a}}_ \bot ^{ - 1}) = }\\ {{{\mathit{\boldsymbol{\hat b}}}_ \bot } \circ \mathit{\boldsymbol{\hat a}}_ \bot ^{ - 1} = \mathit{\boldsymbol{C}}_{{\rm{角}}}^{ - 1}} \end{array} \end{array} $ |
采用类似的推导过程,可分别得到球面三角形的角余弦公式为:
| $ \left\{ {\begin{array}{*{20}{l}} {{\rm{cos}}B = - {\rm{cos}}C{\rm{cos}}A + {\rm{sin}}C{\rm{sin}}A{\rm{cos}}b}\\ {{\rm{cos}}C = - {\rm{cos}}A{\rm{cos}}B + {\rm{sin}}A{\rm{sin}}B{\rm{cos}}c} \end{array}} \right. $ | (26) |
第二五元素公式为:
| $ \left\{ {\begin{array}{*{20}{l}} {{\rm{sin}}B{\rm{cos}}a = {\rm{cos}}A{\rm{sin}}C + {\rm{sin}}A{\rm{cos}}C{\rm{cos}}b}\\ {{\rm{sin}}B{\rm{cos}}c = {\rm{cos}}C{\rm{sin}}A + {\rm{sin}}C{\rm{cos}}A{\rm{cos}}b} \end{array}} \right. $ | (27) |
| $ \left\{ {\begin{array}{*{20}{l}} {{\rm{sin}}C{\rm{cos}}b = {\rm{cos}}B{\rm{sin}}A + {\rm{sin}}B{\rm{cos}}A{\rm{cos}}c}\\ {{\rm{sin}}C{\rm{cos}}a = {\rm{cos}}A{\rm{sin}}B + {\rm{sin}}A{\rm{cos}}B{\rm{cos}}c} \end{array}} \right. $ | (28) |
及
| $ \frac{{{\rm{sin}}A{\rm{sin}}B{\rm{si}}{{\rm{n}}^2}c}}{{{\rm{sin}}C}} = \mathit{\boldsymbol{\hat c}} \cdot (\mathit{\boldsymbol{\hat a}} \times \mathit{\boldsymbol{\hat b}}){\kern 1pt} $ | (30) |
结合式(25)、式(29)和式(30),可得到通常情况下的球面三角形的正弦公式(式(21))。
5 结语实数单位四元数实际上为2个单位向量的格拉斯曼乘积。在向量理论中,引用四元数共轭和逆的概念可方便地表示角度,球面上的大圆弧对应的四元数为球心指向大圆弧终点的单位向量与球心指向大圆弧始点的单位向量逆的格拉斯曼乘积。
如果约定球面角的度量方向指向球面角的顶点,那么球面角对应的四元数只与构成球面角的2个大圆弧的法向矢量有关。球面角对应的四元数为终大圆弧(指向顶点)平面法向的单位向量与始大圆弧(背向顶点)平面法向的单位向量逆的格拉斯曼乘积。利用四元数运算法则可方便地推导球面三角形的边或角正弦和余弦公式及第一和第二五元素公式。
| [1] |
程薇.四元数矩阵代数中的若干问题研究[D].长沙: 国防科学技术大学, 2009 (Cheng Wei. Researches on Some Problems of Quaternion Matrix Algebra[D]. Changsha: National University of Defense Technology, 2009) http://cdmd.cnki.com.cn/article/cdmd-90002-2009213117.htm
(  0) 0) |
| [2] |
Vince J. Imaginary Mathematics for Computer Science[M]. Berlin: Springer International Publishing, 2018
(  0) 0) |
| [3] |
Svehla D. Geometrical Theory of Satellite Orbits and Gravity Field[M]. Berlin: Springer International Publishing, 2018
(  0) 0) |
| [4] |
高宁, 赵星涛, 纪磊, 等. 单位四元数和物方几何约束的绝对定向[J]. 测绘科学, 2018, 43(9): 22-27 (Gao Ning, Zhao Xingtao, Ji Lei, et al. An Absolute Orientation Method for Unit Quaternion and Object Geometric Constraints[J]. Science of Surveying and Mapping, 2018, 43(9): 22-27)
(  0) 0) |
| [5] |
Hamilton W R. Lecture on Quaternions[M]. London: Cambridge Press, 1853
(  0) 0) |
2. School of Urban and Rural Planning and Landscape Architecture, Xuchang University, 88 Bayi Road, Xuchang 461000, China
 2020, Vol. 40
2020, Vol. 40

