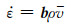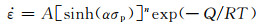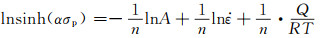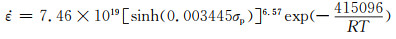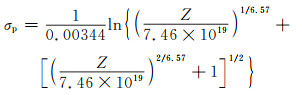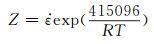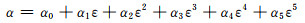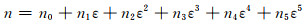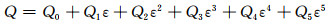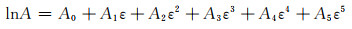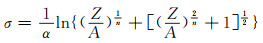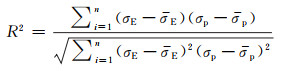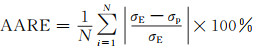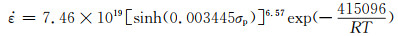1. 贵州大学 材料与冶金学院, 贵阳 550025;
2. 贵州大学 贵州省材料结构与强度重点实验室, 贵阳 550025
收稿日期: 2020-04-11; 修订日期: 2020-10-12
基金项目: 国家自然科学基金资助项目(51804087);贵州省联合基金([2017]7240,[2017]5788);贵州省基础研究计划项目(黔科合基础[2019]1091);贵州省教育厅青年人才成长项目(黔教合KY字[2018]107);贵州大学大学生创新创业训练计划资助项目(贵大(国)创字[2019]007)
通讯作者:
谭元标(1987-), 男, 副教授, 博士, 研究方向:金属材料热加工组织演变与力学性能, 联系地址:贵州省贵阳市花溪区贵州大学材料与冶金学院(550025), E-mail:ybtan1@gzu.edu.cn.
Hot deformation behavior and constitutive equation of TB8 titanium alloy with a lamellar structure of α phase
1. College of Materials and Metallurgy, Guizhou University, Guiyang 550025, China;
2. Guizhou Key Laboratory of Materials Mechanical Behaviour and Microstructure, Guizhou University, Guiyang 550025, China
Abstract: The hot deformation behavior of TB8 titanium alloy with a lamellar α structure in the α+β dulex phase region was investigated. The results show that at the strain rate of 1 s
-1, a continuous flow softening phenomenon is observed in the curve of the samples deformed at 650 ℃, while a discontinuous yield phenomenon is visual in the curve of the samples when the deformation temperature is higher than 650 ℃. The discontinuous yield phenomenon is gradually disappeared with increasing deformation temperature and strain rate. When the strain rate is 0.001 s
-1 and the deformation temperature is 750 ℃ as well as 800 ℃, typical characteristics of dynamic recrystallization is presented in the curve of the samples. The relationship among peak stress
σp temperature
T and strain rate
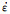
and is characterized by Arrhenius-type constitutive equation. The equation between the material constants (
α,
Q,
n and ln
A) and strain is constructed. The effect of strain on the material constants (
α,
Q,
n and ln
A) of the Arrhenius-type constitutive equation is analyzed. The value of α is increased with true strain, while the values of
Q,
n and ln
A are gradually decreased. The correlation coefficient (
R2) and the AARE value between the experimental and the predicted stress are 0.945 and 9.08%, respectively. This indicates that the strain-compensates Arrhenius type constitutive equation can better predict the flow stress value under different deformation conditions for the TB8 titanium alloy with a lamellar α structure deformed in the α+β dulex phase region.
钛合金因优异的室温和高温力学性能、良好的焊接性能和耐腐蚀性能被广泛用作航空领域的钣金零件、大型锻件、焊接件以及紧固件[1-2]。钛合金构件的力学性能取决于热成形加工后所形成的微观组织。采用合适的热成形加工工艺可获得力学性能优异的微观组织[3]。目前,对于钛合金的热锻成形加工方法,主要在α+β双相区加工、近β相区加工和单β相区加工[4-6]。但是,在不同温度区间进行热加工,合金构件的组织会有所区别,这会导致其力学性能也呈现出较大差异。
TB8钛合金是一种亚稳态β型钛合金,因超高的强度以及高的合金含量,导致其热加工范围较窄。为了控制合金构件的微观组织和改善合金的力学性能,需要深入研究该合金的热变形行为。近年来,关于TB8钛合金热变形行为的研究仅有少量文献报道[7-9]。Duan等[7]和Tang等[8]研究了锻态具有少量α相组织的TB8钛合金的热变形行为,构建了该合金的热变形本构方程。前期工作中,本课题组已研究了具有不同初始β晶粒尺寸的TB8钛合金单β相区的热变形行为,分析了不同晶粒尺寸和热加工参数对该合金热加工行为的作用机理,构建了热变形本构模型。基于热加工图分析,获得了具有不同晶粒尺寸的TB8钛合金的最优热加工参数[9]。TB8钛合金的热变形行为是显著依赖于合金的初始组织和热加工参数。根据文献报道[10-12],对于具有层片状α相组织的钛合金在α+β双相区进行热加工,可获得球状α相和β相的双相组织。这种组织表现出高的塑性和室温强度以及优异的疲劳性能[2]。然而,具有层片状α相组织的TB8钛合金在α+β双相区的热变形行为鲜见报道。
为了改善TB8钛合金的疲劳性能,本工作将深入研究具有层片状α相组织的TB8钛合金在α+β双相区的热变形行为,分析具有层片状α相组织的TB8钛合金的流变行为,建立热加工参数与流变应力之间的本构模型,并预测不同变形条件下的流变应力。
1 实验材料与方法
本工作采用TB8钛合金为锻造棒材,Tβ转变温度为815 ℃[9],该合金的成分如表 1所示。将初始态合金进行1200 ℃,保温1 h的退火热处理,随后炉冷至室温。合金经热处理后的初始组织如图 1所示,粗大的β相晶粒内部包含大量层片状α相。将经热处理的样品加工成ϕ8 mm×12 mm的热变形试样。为了研究具有初始层片状α相组织的TB8钛合金的热变形行为及本构模型,采用Gleeble 3500热模拟压缩机对合金进行热变形实验。变形温度为650~800 ℃,温度间隔为50 ℃,应变速率为0.001~1 s-1,真应变为0.7。将试样以10 ℃/s的速率直接加热到实验设定的变形温度,保温5 min以消除试样内外的温度梯度。变形后立即水冷到室温。
表 1 TB8钛合金的化学成分(质量分数/%)
Table 1
Chemical compositions of the TB8 titanium alloys (mass fraction/%)
| Mo |
Al |
Nb |
Si |
Fe |
C |
N |
O |
H |
Ti |
| 14.5 |
2.9 |
2.85 |
0.19 |
0.07 |
0.02 |
0.02 |
0.09 |
0.002 |
Bal |
2 实验结果与讨论
2.1 流变曲线
图 2为TB8钛合金在不同变形条件下的真应力-应变曲线,在变形条件为650 ℃/1 s-1时,如图 2(a)所示,流变应力随真应变增加快速增加,并达到一个峰值,随后随真应变的增加缓慢降低,呈现一个连续屈服现象。类似的现象也曾在具有初始层片状α相组织的47Zr-45Ti-5Al-3V合金中观察到[12]。这种连续的屈服现象主要与流变局部带的形成、变形热、层状α相的球化等微观组织变化有关。随着变形温度从700 ℃升高到800 ℃,变形初期阶段的流变曲线可观察到一个显著的不连续屈服现象。对于钛合金,这种不连续屈服现象主要发生在β相区的热变形过程中或者是具有单β相组织的钛合金在β相区的热变形过程中。在钛合金中,这种不连续屈服现象目前有两种理论可以解释:静态理论[13]和动态理论[14-15]。但Ankem等[16]研究发现Ti-V和Ti-Mn合金在高温拉伸变形后,在同一温度下再次变形时并未再现不连续屈服现象。因此,他们认为静态理论并不能很好地解释这种现象。对于动态理论,该理论认为不连续屈服现象与大量可动位错突然从晶界增殖有关。在本工作中,具有初始层片状α相的TB8钛合金的热成形是在α+β双相区进行,这表明合金在变形过程中发生了α→β相的转变。众所周知,热变形过程中,可动位错密度是依赖于应变速率。两者之间可用如下关系描述[16]:
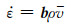
|
(1) |
式中: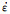 是应变速率;b是柏氏矢量;ρ是位错密度;v是位错运动平均速率。在较低变形温度和高应变速率条件下,一旦塑性变形开始,合金内部的可动位错就会快速增殖,高密度的位错促进了α→β相的转变[17]。在给定的变形温度条件下,随着应变速率的增加,流变应力逐渐降低(见图 2(b), (c))。在应变速率为0.1 s-1时,流变曲线呈现出锯齿流变现象,这种锯齿流变现象可能由于合金热变形过程中发生动态应变时效引起的[18]。当应变速率降低到0.001 s-1时,变形温度为750 ℃和800 ℃的流变曲线展现出典型的动态再结晶特征(见图 2(d))。
是应变速率;b是柏氏矢量;ρ是位错密度;v是位错运动平均速率。在较低变形温度和高应变速率条件下,一旦塑性变形开始,合金内部的可动位错就会快速增殖,高密度的位错促进了α→β相的转变[17]。在给定的变形温度条件下,随着应变速率的增加,流变应力逐渐降低(见图 2(b), (c))。在应变速率为0.1 s-1时,流变曲线呈现出锯齿流变现象,这种锯齿流变现象可能由于合金热变形过程中发生动态应变时效引起的[18]。当应变速率降低到0.001 s-1时,变形温度为750 ℃和800 ℃的流变曲线展现出典型的动态再结晶特征(见图 2(d))。
2.2 动力学分析
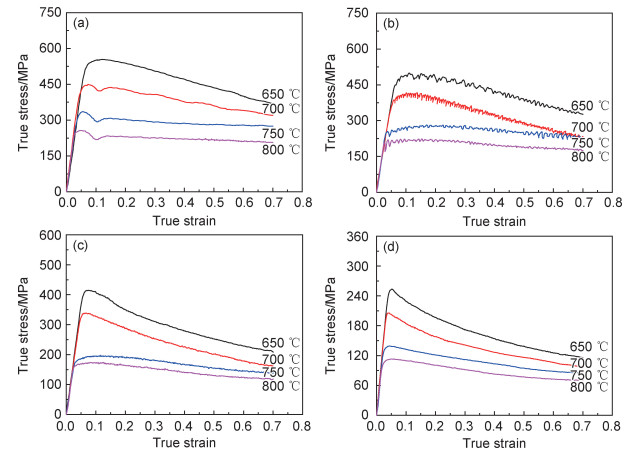
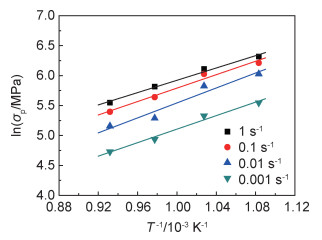
热变形过程中,应变速率(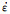 )和变形温度(T)对流变应力(σ)有显著的影响。为了定量描述σ,T和
)和变形温度(T)对流变应力(σ)有显著的影响。为了定量描述σ,T和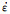 之间的关系,可选取所有变形条件下应力-应变曲线的峰值应力(σp)作为代表应力进行分析。图 3和图 4为TB8钛合金峰值应力与应变速率及变形温度的关系曲线,由图可知,σp随T的降低和
之间的关系,可选取所有变形条件下应力-应变曲线的峰值应力(σp)作为代表应力进行分析。图 3和图 4为TB8钛合金峰值应力与应变速率及变形温度的关系曲线,由图可知,σp随T的降低和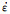 的增加而增加,三者之间的关系可用如下方程进行描述[9]:
的增加而增加,三者之间的关系可用如下方程进行描述[9]:
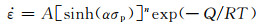
|
(2) |
式中:Q为形变激活能;R为理想气体常数;A,α,n为与温度无关的材料常数。将式(2)取对数,得出以下公式:
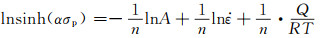
|
(3) |
将实验数据代入式(3),计算分析可得:α=0.00344 MPa-1;Q=415.096 kJ/mol;n=6.57;A=7.46×1019 s-1。
因此,σp, T和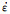 三者之间的关系模型如下:
三者之间的关系模型如下:
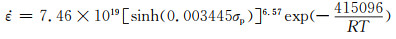
|
(4) |
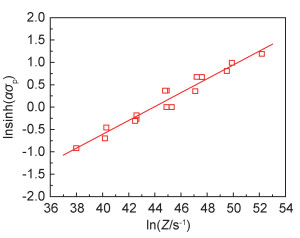
在金属热加工过程中,T和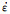 对合金流变行为的影响可用Zener-Hollomon (Z=
对合金流变行为的影响可用Zener-Hollomon (Z=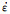 exp(Q/RT))参数进行表征。在获得材料常数之后,即可计算出不同变形条件下的Z参数。图 5为TB8钛合金峰值应力与Z参数之间的关系曲线,由图可知,Z参数与ln[sinh(ασp)]之间呈良好线性关系。这说明本工作建立的本构方程是合理的。当不同变形条件的Z参数确定之后,σp可描述为Z参数的函数,如式(5)所示:
exp(Q/RT))参数进行表征。在获得材料常数之后,即可计算出不同变形条件下的Z参数。图 5为TB8钛合金峰值应力与Z参数之间的关系曲线,由图可知,Z参数与ln[sinh(ασp)]之间呈良好线性关系。这说明本工作建立的本构方程是合理的。当不同变形条件的Z参数确定之后,σp可描述为Z参数的函数,如式(5)所示:
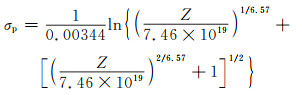
|
(5) |
通过式(3)计算出Q=415.096 kJ/mol, 代入Zener-Hollomon(Z=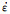 exp(Q/RT))参数,得出以下公式:
exp(Q/RT))参数,得出以下公式:
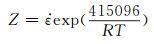
|
(6) |
通常,根据公式(3)计算合金热变形激活能以及材料常数时忽略了应变对流变行为的作用。然而,一些文献已报道,热变形过程中的热变形激活能和材料常数会受到应变的影响[19-20]。为了分析热变形激活能和材料常数与应变之间的定量关系,可认为Q,α,n和lnA是与应变相关的多项式函数,在本构方程中考虑应变的影响。α,A,n和Q与真应变ε之间可分别用式(7)~(10)的五次多项式表示。
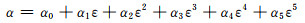
|
(7) |
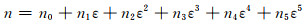
|
(8) |
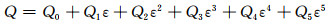
|
(9) |
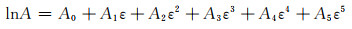
|
(10) |
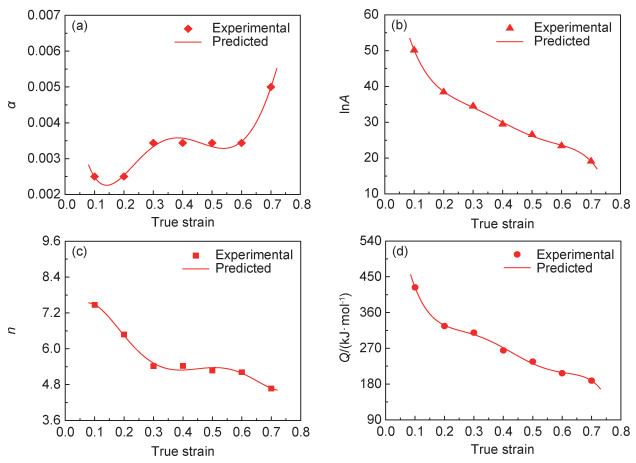
先根据式(2),(3)计算出不同应变条件下的α,A,n和Q值。然后将其带入式(7)~(10)求出方程的各项系数,即可获得α,A,n和Q值与ε之间多项式关系表达式。式(7)~(10)的各项系数如表 2所示。不同应变条件下α,lnA,n和Q值的变化情况如图 6所示,表明根据实验数据和多项式函数计算获得的形变激活能和材料常数保持良好的相关性。从图 6中可以观察到,α值是随真应变的增加而增加,而A,n和Q值是随真应变的增加而逐渐降低。
表 2 TB8钛合金的α, n, Q和lnA的参数拟合结果
Table 2
Polynomial fitting results of α, n, Q and lnA for the TB8 titanium alloys
| α |
n |
Q/(kJ·mol-1) |
ln(A/s-1) |
| α0=0.00653 |
n0=6.36 |
Q0=816.93 |
A0=87.37 |
| α1=-0.07831 |
n1=35.27 |
Q1=-6683.08 |
A1=-604.09 |
| α2=0.4905 |
n2=-330.13 |
Q2=-35624.08 |
A2=2994.58 |
| α3=-1.24693 |
n3=-1019.87 |
Q3=-92680.42 |
A3=-7689.02 |
| α4=1.3697 |
n4=-1327.65 |
Q4=-112958.33 |
A4=9511.36 |
| α5=-0.525 |
n5=620.83 |
Q5=51991.67 |
A5=-4516.67 |
当修正的α,A,n和Q值计算出之后,可根据如下方程预测不同应变条件下的流变应力值:
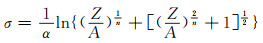
|
(11) |
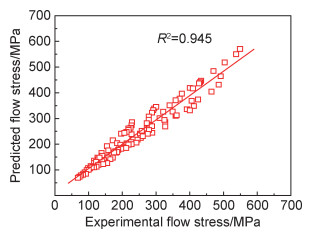
图 7为TB8钛合金在不同应变速率下实验流变应力与预测流变应力的比较。如图 7所示,在所有变形条件下实验获得的流变应力值与式(11)预测的流变应力值之间都保持较高的吻合度。为了进一步分析实验获得的流变应力与本构方程预测的流变应力之间的相关性,可利用两者之间的相关系数(R2)和平均相对误差(AARE)参数来进行定量分析。两者之间的R2和AARE值可通过式(12),(13)进行计算[20-21]:
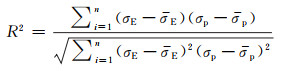
|
(12) |
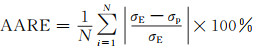
|
(13) |
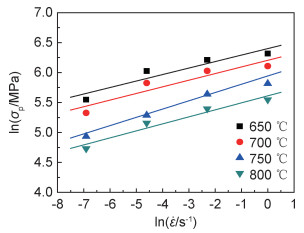
式中:σE和σp分别为实验应力和预测应力;σE和σp分别为对应的应力平均值;N为本工作测试的实验条件总数。图 8为不同变形条件下预测应力与实验应力的相关性,由图可知,实验应力和本构方程预测的流变应力之间具有良好的相关性。在本工作中,R和AARE值经计算分别为0.945和9.08%,这表明了上述建立的本构方程能够很好地表征σp,T和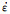 三者之间的定量关系,并精良地预测具有初始层片状α相的TB8钛合金在α+β双相区热变形过程中不同变形条件下的流变应力值。
三者之间的定量关系,并精良地预测具有初始层片状α相的TB8钛合金在α+β双相区热变形过程中不同变形条件下的流变应力值。
3 结论
(1) 在应变速率为1 s-1时,650 ℃变形的流变曲线展现出连续的流变软化,当温度高于650 ℃时,流变曲线展现出一个应力降现象。随应变速率的降低和温度的增加,应力降现象消失。当应变速率为0.001 s-1时,750 ℃和800 ℃的流变曲线呈现出动态再结晶特征。
(2) σp,T和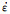 三者可通过Arrhenius-type本构方程进行描述,具体关系如下:
三者可通过Arrhenius-type本构方程进行描述,具体关系如下:
(3) α,A,n和Q值与真应变ε之间的关系模型已建立。应变对α,A,n和Q值的影响已分析。α值是随真应变的增加而增加,而A,n和Q值是随应变的增加而逐渐降低。实验应力值和预测应力值之间的相关系数和平均相对误差参数分别为0.945和9.08%。
 2021, Vol. 49
2021, Vol. 49






 三者之间的关系已通过Arrhenius-type本构方程进行表征,建立了材料常数α,A,n和Q值与真应变之间的关系模型,并分析了应变对α,A,n和Q值的影响。α值随真应变的增加而增加,而A,n和Q的值随真应变的增加而逐渐降低。实验应力值和预测应力值之间的相关系数和平均相对误差参数分别为0.945和9.08%。这表明本工作建立的应变补偿的热变形本构方程能够很好地预测具有层片状α相组织的TB8钛合金在α+β双相区热变形过程中的流变应力。
三者之间的关系已通过Arrhenius-type本构方程进行表征,建立了材料常数α,A,n和Q值与真应变之间的关系模型,并分析了应变对α,A,n和Q值的影响。α值随真应变的增加而增加,而A,n和Q的值随真应变的增加而逐渐降低。实验应力值和预测应力值之间的相关系数和平均相对误差参数分别为0.945和9.08%。这表明本工作建立的应变补偿的热变形本构方程能够很好地预测具有层片状α相组织的TB8钛合金在α+β双相区热变形过程中的流变应力。